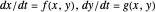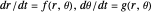Details

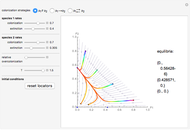
Some of the characteristics of the systems of ODEs selected for this tour are described. These insights suggest interesting explorations.

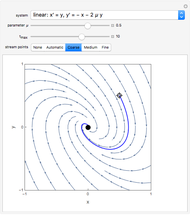
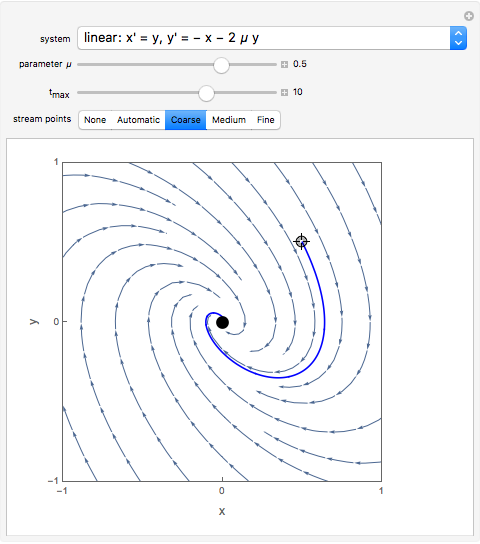
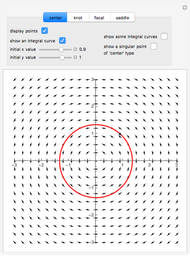
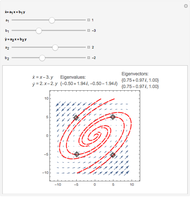
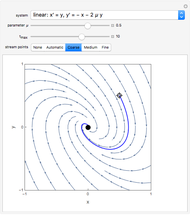
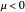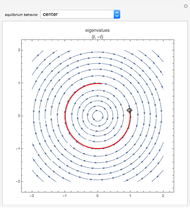
For the linear system, produces an unstable spiral, 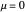is a center, and 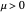produces a stable spiral.

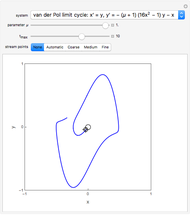
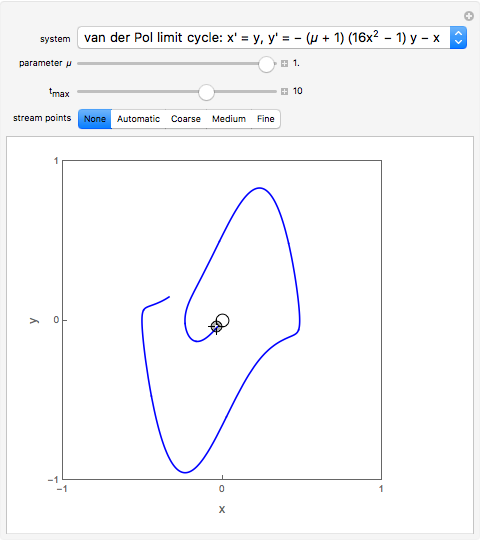
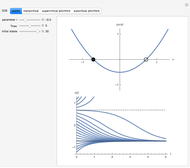
For the van der Pol equation, 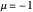reduces to a linear center. As increases, a limit cycle forms.

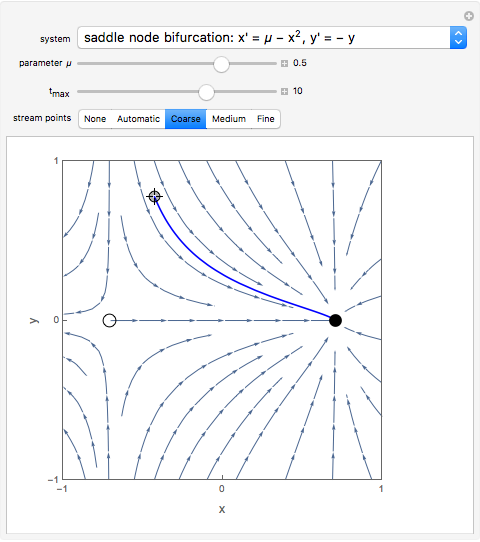
The critical value of the parameter for all the bifurcation cases occurs at 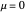, where the number and stability of the fixed points change, causing a qualitatively different dynamical picture.

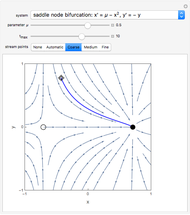
The saddle node has no fixed points for 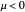and fixed points at 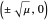for .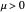

The transcritical fixed points at 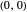and 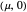switch stability at .

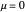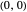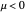The supercritical pitchfork has one stable fixed point at for . When 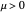, 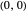becomes unstable and a pair of symmetric stable points forms at .

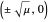The subcritical pitchfork has a stable fixed point at 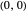and a symmetric pair of unstable points at 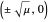for 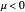. When 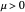, 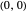becomes unstable and all trajectories diverge.

For both the supercritical and subcritical Hopf cases, the origin is a stable spiral when 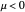and bifurcates to an unstable spiral when 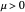. When 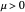, the trajectories approach limit cycles.

Reference:

S. H. Strogatz, Nonlinear Dynamics and Chaos, Jackson, TN: Perseus Books Publishing, 1994.