Conical Anamorphic Projection of Photographic Images

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
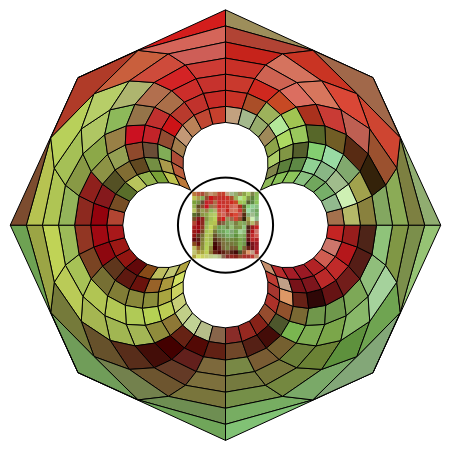
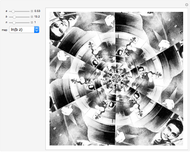
This Demonstration explores the anamorphic projection of rasterized photographic images.
[more]
Contributed by: Erik Mahieu (November 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
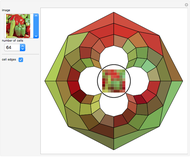
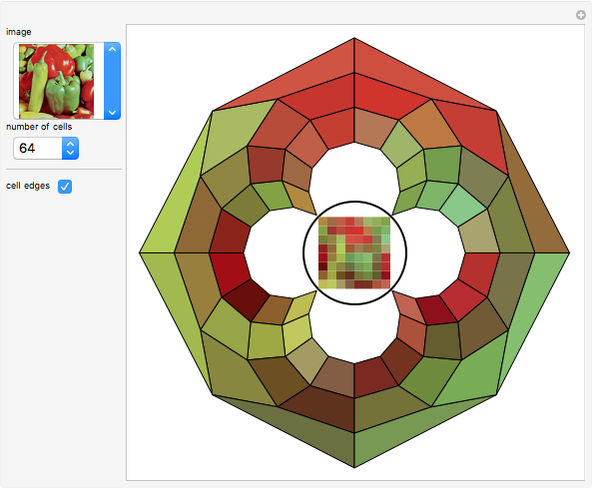
Snapshot 1 shows how the different cells of a simple 8×8 raster are projected.
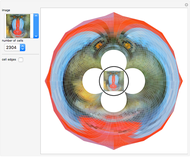
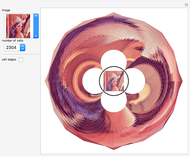
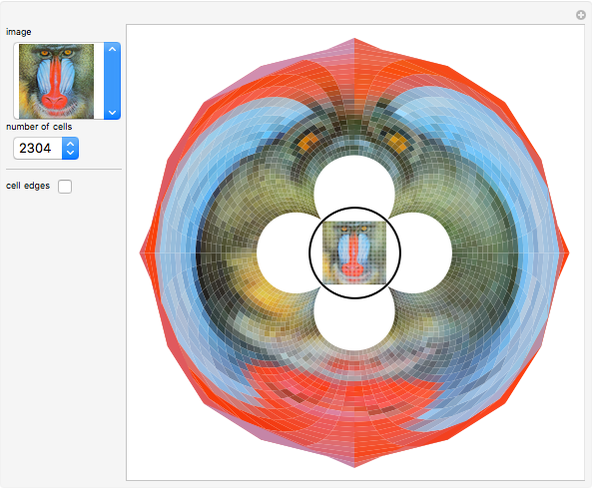
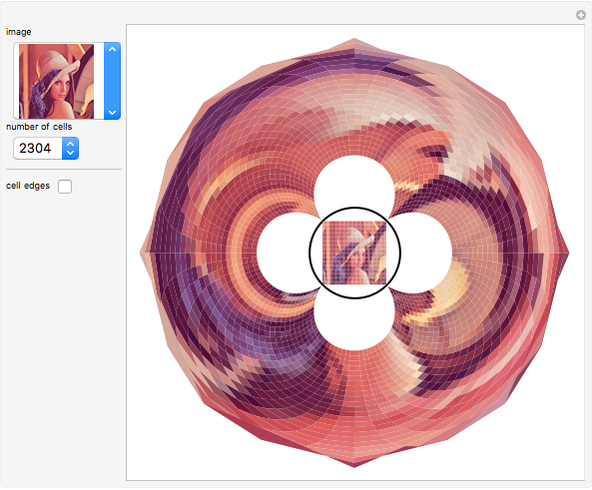
Snapshots 2 and 3 are projections of images divided in a raster of 48×48 cells.
Permanent Citation
"Conical Anamorphic Projection of Photographic Images"
http://demonstrations.wolfram.com/ConicalAnamorphicProjectionOfPhotographicImages/
Wolfram Demonstrations Project
Published: November 4 2011