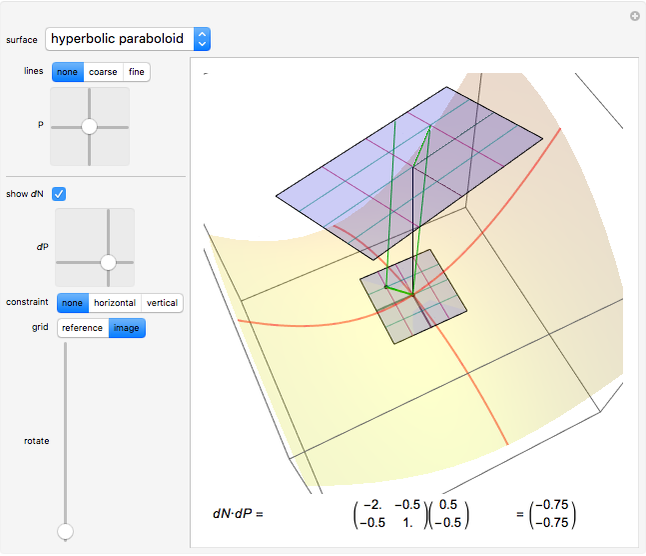
The Geometry of Lagrange Multipliers

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
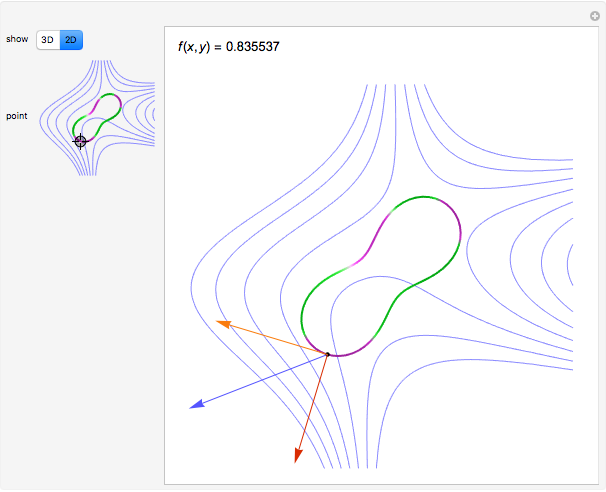
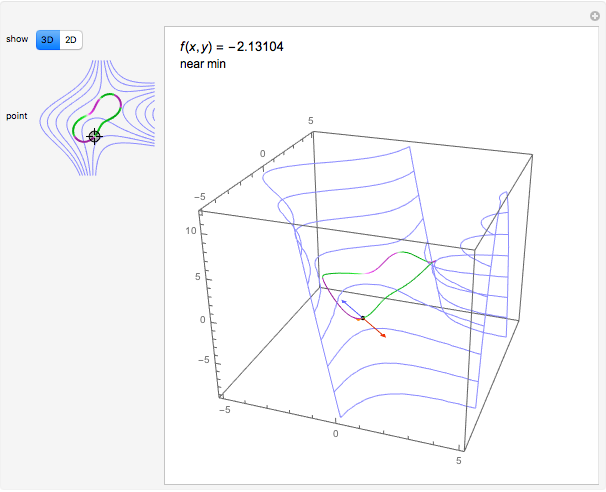
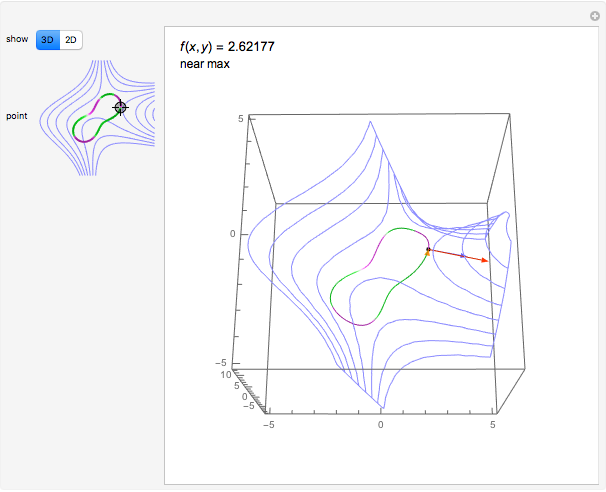
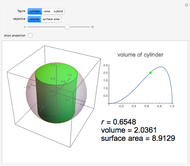
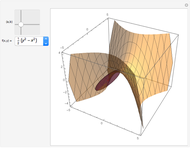
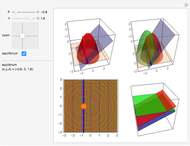
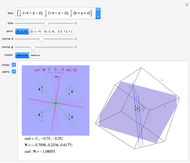
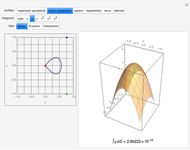
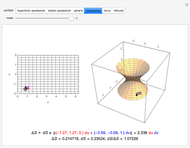
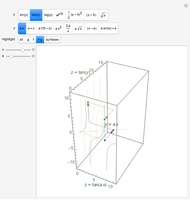
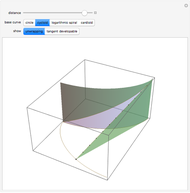
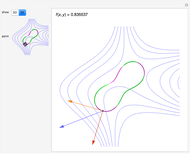
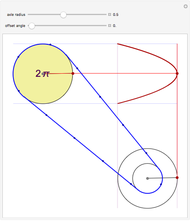
Move a point around a constraint curve to see the relationship between the blue gradient of the function  to be optimized and the red gradient of the function
to be optimized and the red gradient of the function  for the constraint
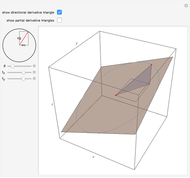
for the constraint  . The orange vector is the projection of the gradient of
. The orange vector is the projection of the gradient of  onto the tangent line of the constraint curve; its direction is the direction of increase along the constraint and its magnitude is the slope (that is, the directional derivative) of
onto the tangent line of the constraint curve; its direction is the direction of increase along the constraint and its magnitude is the slope (that is, the directional derivative) of  in that direction. There are both 2D and 3D views, with the constraint curve laid out upon the graph of the function.
in that direction. There are both 2D and 3D views, with the constraint curve laid out upon the graph of the function.
Contributed by: Michael Rogers (Oxford College of Emory University) (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Permanent Citation