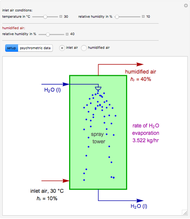
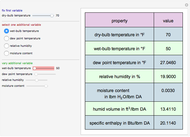
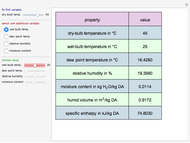
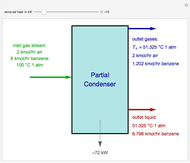
If , one recovers the explicit Euler integration scheme.

If , one recovers the implicit Euler integration scheme.

If , one recovers the Crank–Nicholson integration scheme.

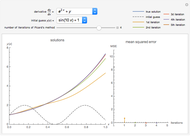
Absolute stability means that the global error does not grow without bound.

Absolute stability of the numerical integration method requires the following condition:

, where the dimensionless time step is .

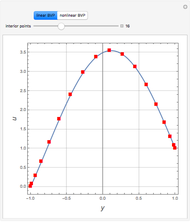
Consider the Euler integration scheme (i.e., ); the global error, , satisfies , where is the local truncation error at time step . This equation reveals the origin of exponential growth in the global error. Indeed, at each time step the global error is multiplied by . If is outside the disk with center at of radius 1, the global error will grow out of bounds and the numerical method will fail to give an accurate solution of the differential equation. Thus, the choice of the time step that gives good solutions is restricted to a specific domain and the method is not absolutely stable.

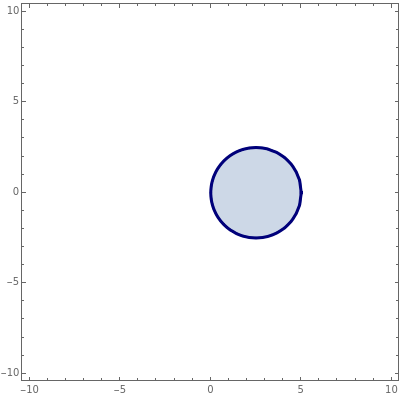
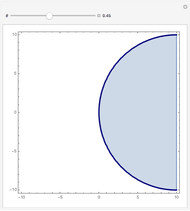
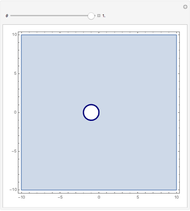
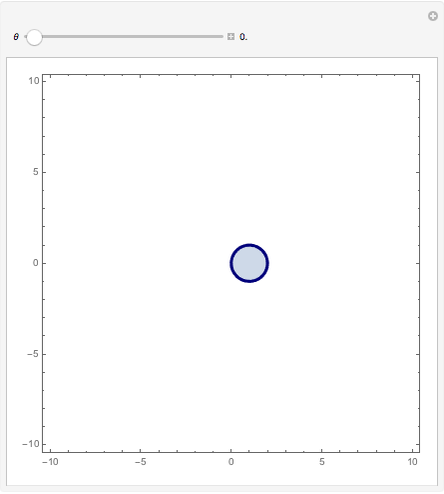
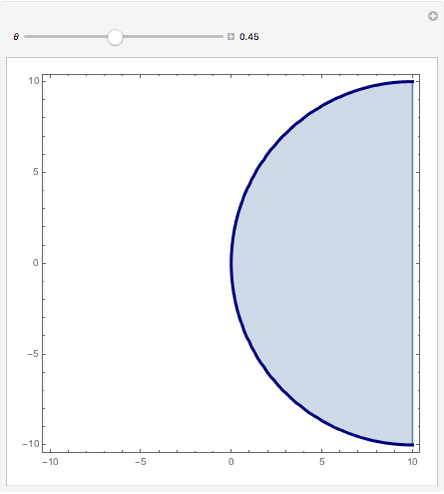
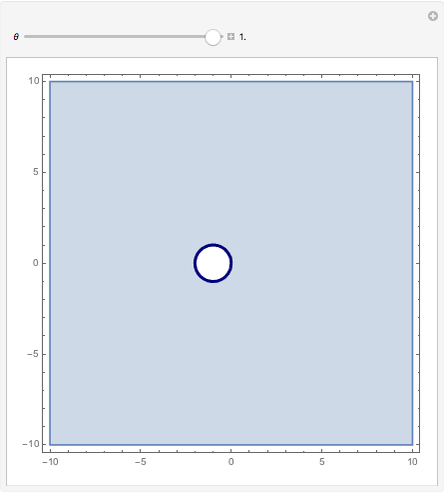
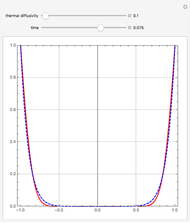
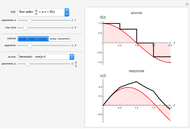
The present Demonstration shows the region plot in the plane for user-set values of . With , the light blue region corresponds to and the blue curve is where . Thus the light blue region represents choices of that give absolute stability.

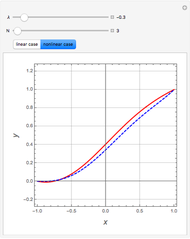
Ifis real, one can conclude that the explicit Euler method is not absolutely stable, while both the implicit Euler and Crank–Nicholson methods are absolutely stable.