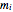Diffusion Coefficients for Multicomponent Gases

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
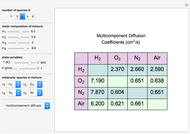
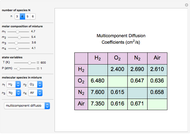
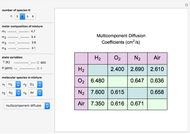
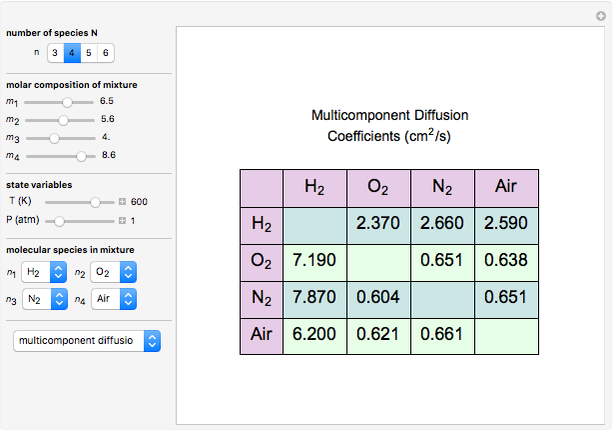
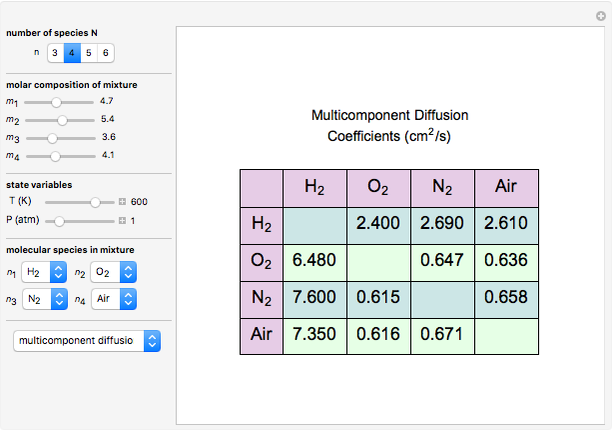
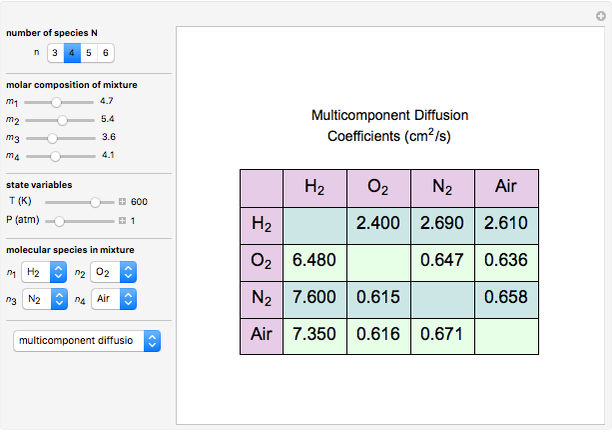
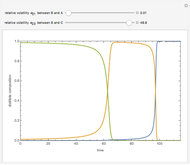
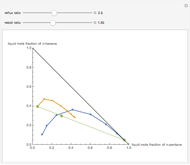
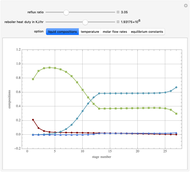
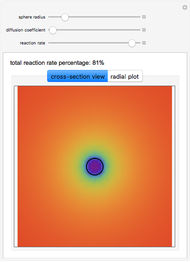
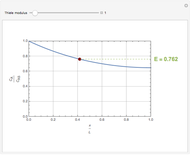
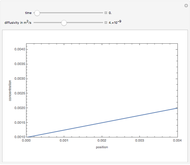
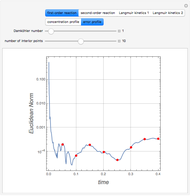
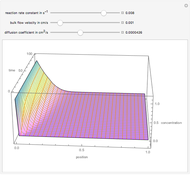
In the analysis of combustion problems and other multicomponent reacting systems, it is important to account for the relevant transport properties of the reacting mixture. This Demonstration is concerned with computing the ordinary diffusion coefficients of a mixture. The thermal diffusion coefficients, which describe the Soret effect, are not considered.
[more]
Contributed by: Brian G. Higgins and Housam Binous (March 2013)
Open content licensed under CC BY-NC-SA
Snapshots
Details
References
[1] S. R. Turns, An Introduction to Combustion: Concepts and Applications, 3rd ed., New York: McGraw–Hill, 2012.
[2] G. Dixon-Lewis, "Flame Structure and Flame Reaction Kinetics. II. Transport Phenomena in Multicomponent Systems," Proceedings of the Royal Society of London, Series A, 307, 1968 pp. 111–135. www.jstor.org/stable/2416190.
[3] R. B. Bird, W. E. Stewart, and E. N. Lightfoot, Transport Phenomena, 2nd ed., New York: John Wiley & Sons, 2002.
Permanent Citation