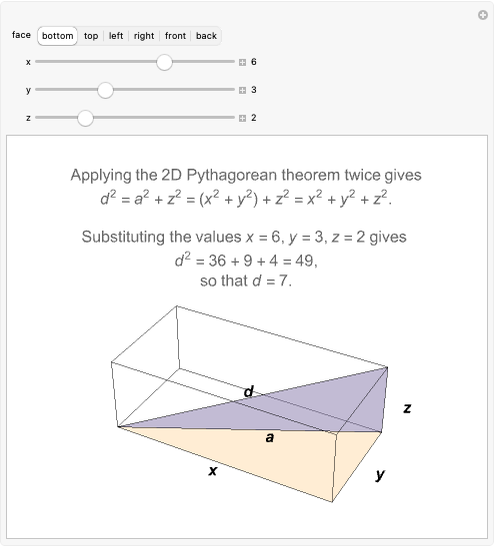
Japanese Theorem for Three Tangent Circles and a Triangle

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
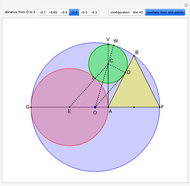
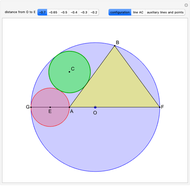
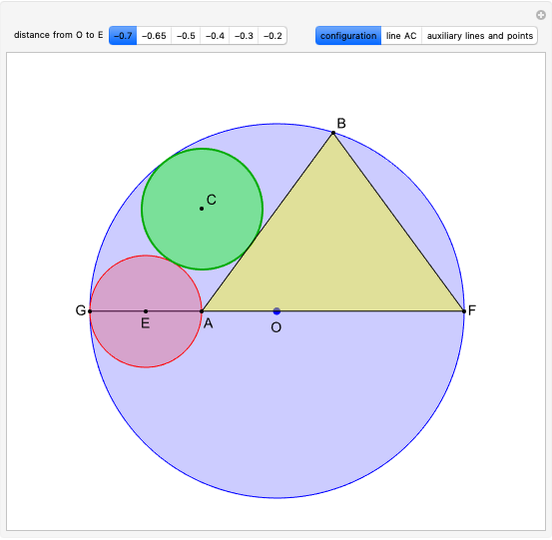
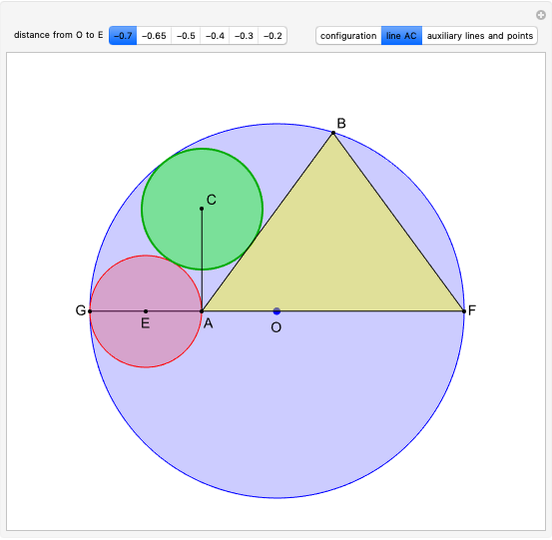
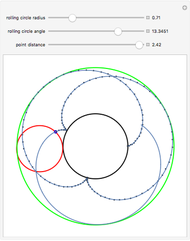
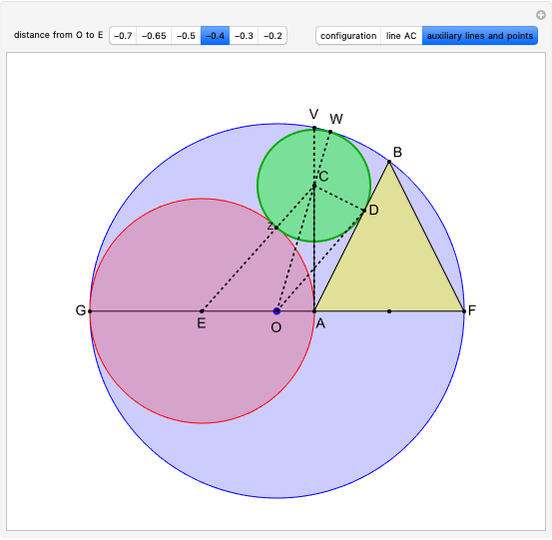
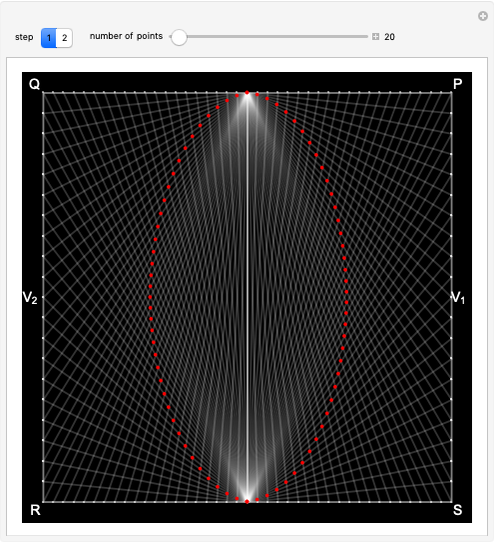
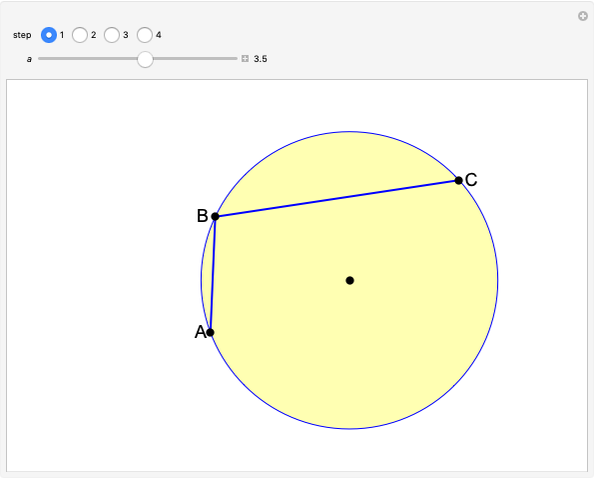
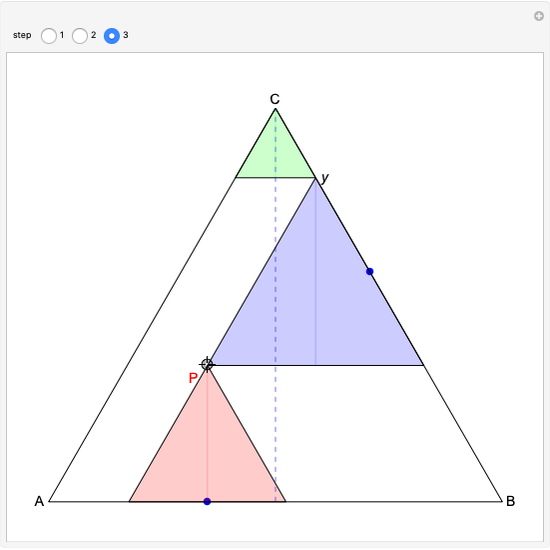
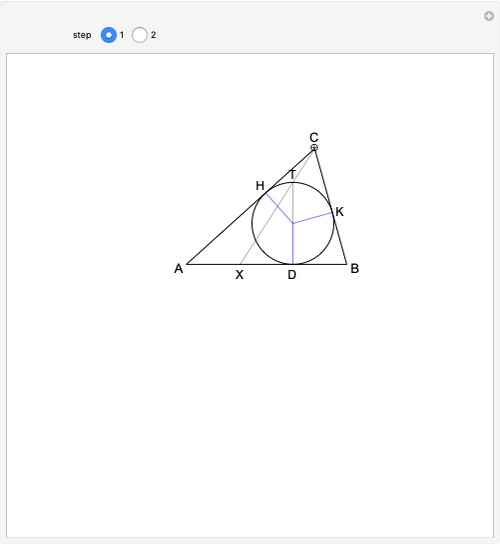
This Japanese classical theorem (Sangaku) deals with a configuration of three tangent circles (blue, purple and green) and an isosceles triangle that is tangent to one of the three circles (green) and has two of its vertices on the circumference of the largest circle (blue). The theorem states that the line joining the left vertex of the triangle to the center of the green circle is perpendicular to the horizontal diameter.
Contributed by: Tomas Garza (August 2022)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Each of the three circles is tangent to the other two. The green circle is tangent to the side  of the yellow isosceles triangle. The theorem states that the line from
of the yellow isosceles triangle. The theorem states that the line from  to
to  is perpendicular to the diameter
is perpendicular to the diameter  of the blue circle. You can change the position of the center of the purple circle.
of the blue circle. You can change the position of the center of the purple circle.
Click the "configuration" button to see the triangle and circles. Click the "line AC" button to see the subject of the theorem. Click the "auxiliary points and lines" button to see the construction used in the proof of the theorem.
Permanent Citation