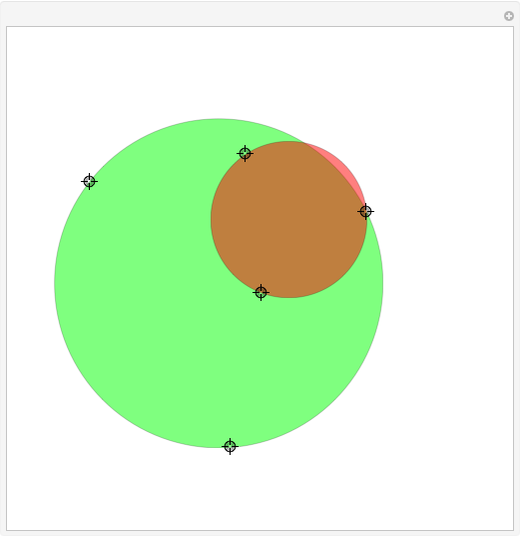
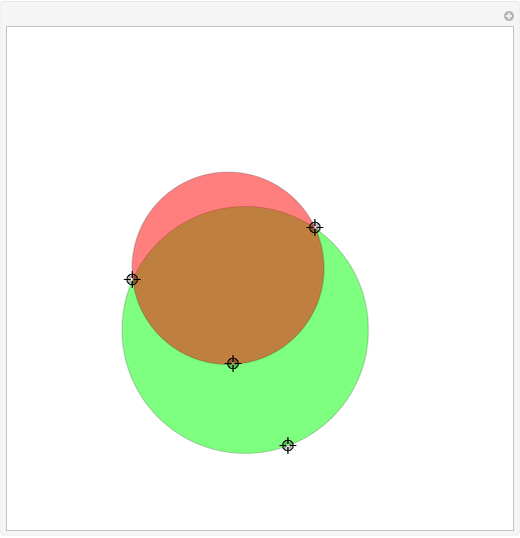
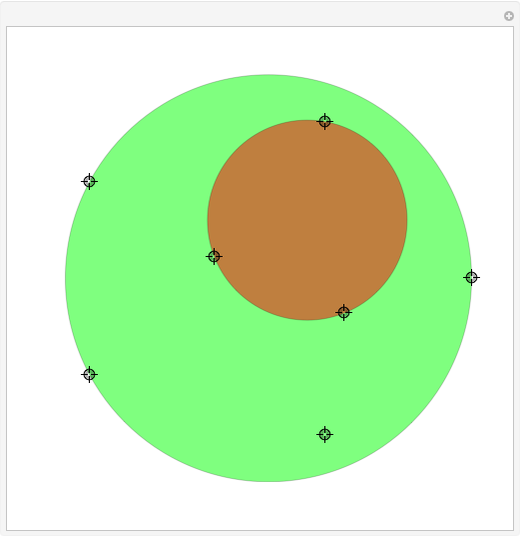
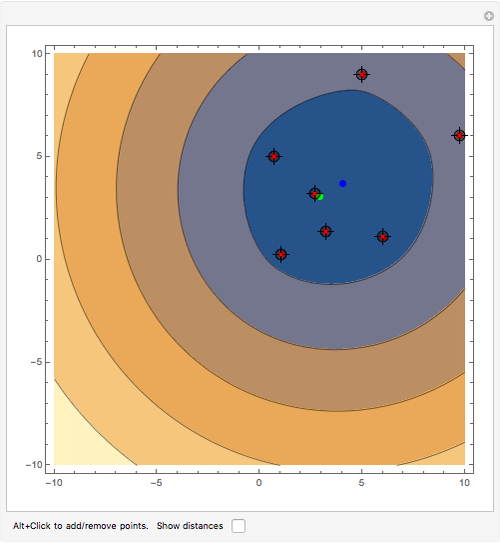
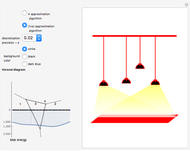
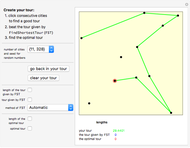
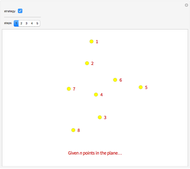
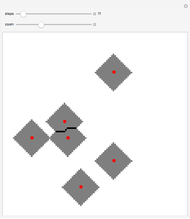
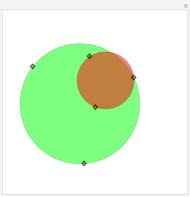
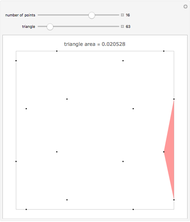
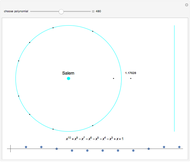
Take a set of random points. In the "Texas sharpshooter problem", the smallest circle that contains three of the points is found. In the "bomb problem", the smallest circle that contains all the points is found.
The Texas sharpshooter is a classic fallacy. Allegorically, poorly aimed shots are made at a barn, and the closest set of three is circled as a bullseye.
The bomb problem comes from military tactics. What is the least powerful bomb that can destroy all targets, and where should it be dropped?
Surprisingly, both of these violent-sounding mathematical problems make use of the same algorithm.
Browse all topics
