The Uranus Puzzle

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
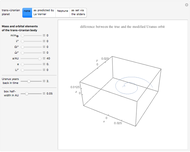
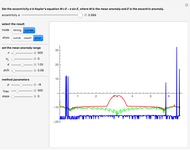
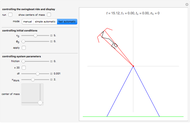
This Demonstration deals with the orbits of the planets Uranus and Neptune. It allows us to reenact a highlight of nineteenth-century astronomy. During the period 1781–1846, when Uranus had been discovered, whereas Neptune was still unknown, astronomers were puzzled by the observed motion of Uranus. It turned out that it was impossible to set up initial conditions for Uranus which together with the forces from the Sun, Jupiter, and Saturn would result in an orbit consistent with observation for more than a few decades (from old stellar catalogs Uranus positions had become available back to 1690). The idea was born that a trans-Uranian planet might cause the anomaly of Uranus' orbit and that a thorough analysis of these anomalies should allow one to calculate the position of the causative planet. As is well known, this program worked, and the computational results of Urbain Le Verrier triggered the discovery of the planet, which finally was baptized Neptune. This Demonstration simulates this Uranus orbit puzzle and lets us solve it by proposing a suitable celestial body. With some trial and error we can do much better than Le Verrier and come much closer to the true properties of Neptune.
[more]
Contributed by: Ulrich Mutze (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
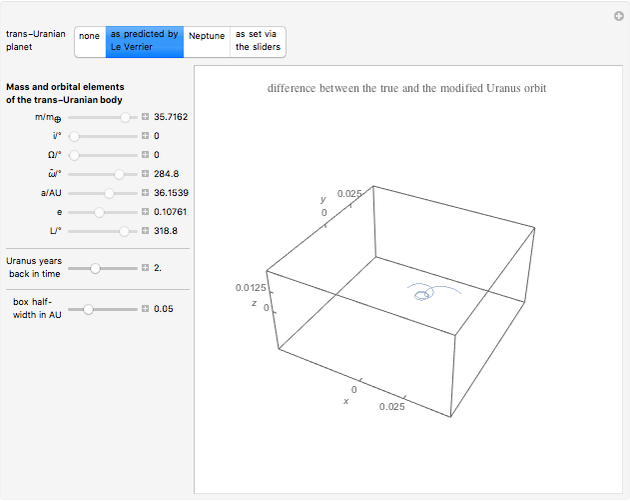
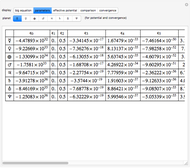
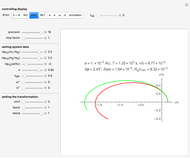
Snapshot 1: This defines the puzzle: an astronomer who determines the Uranus orbit that agrees best with observations and with Newtonian gravitation will always find the difference in orbits, shown here as a function of time.
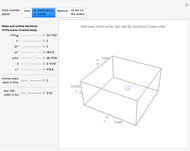
Snapshot 2: This shows to what extent Le Verrier's prediction reduces the size of the orbit difference. We easily see that this is a local minimum. Playing with the sliders we find that the orbit difference grows with all small changes. Although Le Verrier's result was successful in predicting Neptune's spherical position in the years 1846–1847, it gives a rather pure description of Neptune's orbit. This is understandable since for Le Verrier it could not have been natural to assume that the Titius–Bode rule, which predicts a semiaxis 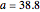 for a planet next to Uranus, would fail completely (the true value being 30.3). So, he overestimated
for a planet next to Uranus, would fail completely (the true value being 30.3). So, he overestimated  and interpreted computational indications for a smaller distance as saying that the Uranus orbit was considerably eccentric (although it is virtually circular). Actually, Le Verrier's Neptune orbit approaches the true one rather closely during the time span 1820–1850.
and interpreted computational indications for a smaller distance as saying that the Uranus orbit was considerably eccentric (although it is virtually circular). Actually, Le Verrier's Neptune orbit approaches the true one rather closely during the time span 1820–1850.
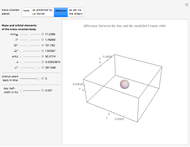
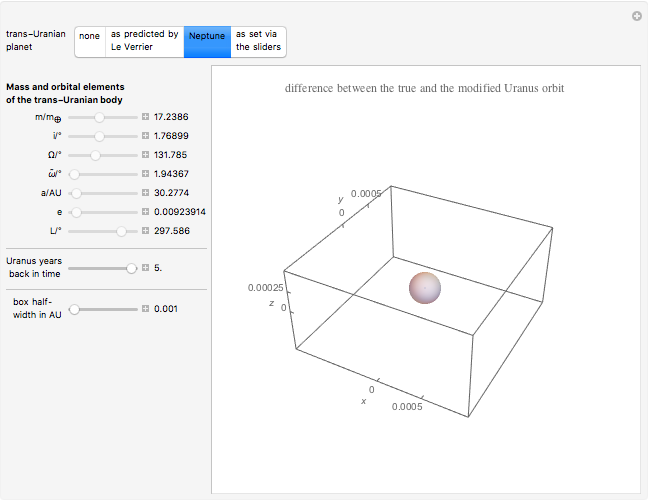
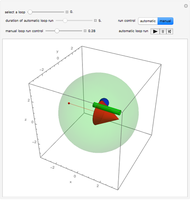
Snapshot 3: Endowing the hypothetical Neptune with its true properties lets the orbit difference disappear completely. This is so even if the scale is set such that the spatial extent of Uranus becomes apparent.
Snapshot 4: This also shows that a Neptune orbit that lies in the ecliptic plane (such as Le Verrier's prediction) leads to a difference orbit that does not exceed Uranus' diameter.
Snapshot 5: This is a fast and simple adaptation starting with the assumption of a circular orbit in the plane of the ecliptic, setting the mass of Neptune equal to that of Uranus, setting 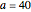 ,
,  , and playing with the controls for
, and playing with the controls for  ,
, 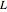 , and
, and 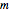 . The orbit representation is orders of magnitude better than the one by Le Verrier's prediction. This, of course, is said with the benefit of hindsight. One should also observe that assuming a circular orbit would be in marked error in predicting the next body—Pluto.
. The orbit representation is orders of magnitude better than the one by Le Verrier's prediction. This, of course, is said with the benefit of hindsight. One should also observe that assuming a circular orbit would be in marked error in predicting the next body—Pluto.
Some simplifications are necessary in order that the Demonstration be of reasonable size and sufficiently responsive: we consider only the planets Jupiter, Saturn, Uranus, and Neptune, and by working in heliocentric coordinates, get rid of the dynamical variables of the Sun. This defines a virtually closed subsystem of the true solar system. Neither the lightweight bodies of the inner solar system, nor those of the surrounding Kuiper belt exert more than a tiny influence on the giant planets. The dynamics of our model system is that of non-relativistic point masses with Newtonian gravitation as the only forces. The corresponding differential equations are solved numerically to produce four orbits over a time span of 100 years quite readily.
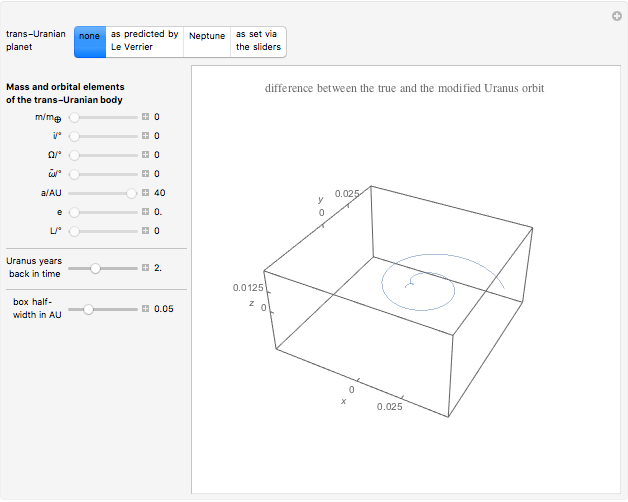
There is a specific point 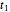 in time (January 1, 1847, for which Le Verrier published orbital elements of his predicted planet) for which the program lets you change all properties of the simulated planet Neptune. Then we compute the orbits of the whole system in two runs: one with Neptune's properties unmodified and the next with the properties modified according to the settings of the controls. The orbits of Jupiter and Saturn are virtually the same in the two runs, but the orbits of Uranus and Neptune are more seriously affected. The deformation of the Uranus orbit, a shift vector which is a function of time, is the quantity to be shown in the graphics box of the Demonstration. Integration is done backward in time from
in time (January 1, 1847, for which Le Verrier published orbital elements of his predicted planet) for which the program lets you change all properties of the simulated planet Neptune. Then we compute the orbits of the whole system in two runs: one with Neptune's properties unmodified and the next with the properties modified according to the settings of the controls. The orbits of Jupiter and Saturn are virtually the same in the two runs, but the orbits of Uranus and Neptune are more seriously affected. The deformation of the Uranus orbit, a shift vector which is a function of time, is the quantity to be shown in the graphics box of the Demonstration. Integration is done backward in time from 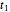 to a point in time which can be set by a slider.
to a point in time which can be set by a slider.
The unmodified solution is a good model for the orbits that were available to the old astronomers for observation, whereas the modified Uranus orbit shows the anomalies mentioned above: especially for the case that the modification gave Neptune a vanishing mass, we get a Uranus orbit as it would be calculated by somebody who does not know about a trans-Uranian planet and who thus observes the full amount of discrepancy that once triggered the idea of a trans-Uranian planet. By choosing less radical modifications we observe how the difference between the two Uranus orbits grows or shrinks.
One should be aware that comparing orbits in 3D space as enabled by this simulation transcends what an Earth-bound astronomer can accomplish. Deducing a spacetime path for a planet from the spherical positions (directions) measured by means of a telescope needs a hypothesis on the gravitational field near the planet. This makes fixing initial conditions for planets an iterative process involving the mass-values of the bodies under consideration. So, given the observational data which an Earth-bound astronomer would get from the exact orbits of our simulation, this astronomer would compute for the 3D-velocity of Uranus a velocity that would deviate from the exact velocity of the simulation by a small correction that depends on the modified properties of a simulated Neptune. It would be too complicated, and would add little to the understanding of the situation if we did include this aspect into the program.
Permanent Citation