Line Jaggedness Visualization with the Mandelbrot-Weierstrass Function

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
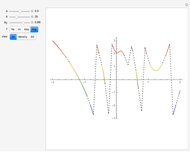
This Demonstration helps visualize the effect of resolution and the apparent fractal dimension on the jaggedness of an irregular line.
Contributed by: Mark D. Normand and Micha Peleg (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
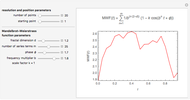
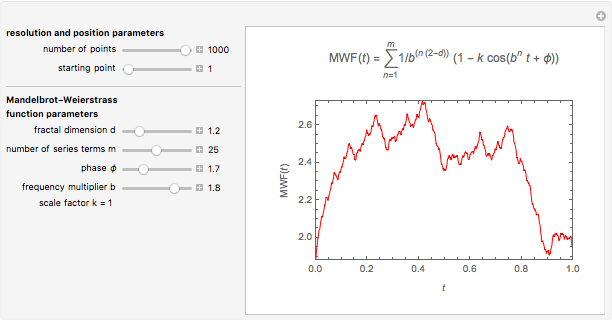
Snapshot 1: moderately jagged line at low resolution
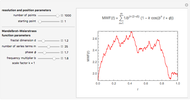
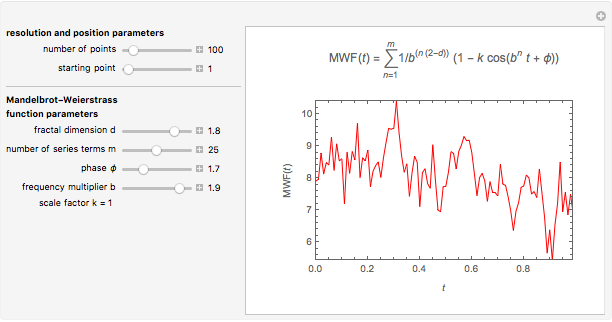
Snapshot 2: moderately jagged line at high resolution
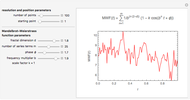
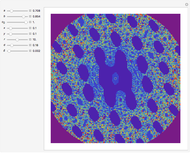
Snapshot 3: highly jagged line at low resolution
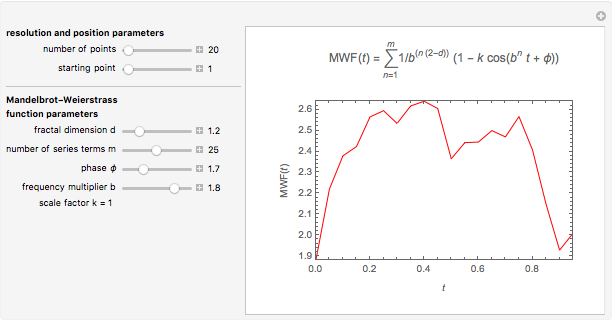
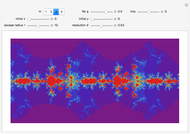
Snapshot 4: highly jagged line at high resolution
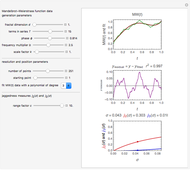
This Demonstration helps the user to visualize the jaggedness of an irregular line, time series, or signature by allowing its generation at different apparent fractal dimensions (in the range 1 to 2) using the Mandelbrot–Weierstrass function as the model. The function is displayed above the plot. The values of four of the function's five parameters  ,
, 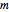 ,
,  , and
, and  are set using sliders while
are set using sliders while  is fixed at 1;
is fixed at 1;  is the apparent fractal dimension,
is the apparent fractal dimension,  is the number of terms computed from the infinite series,
is the number of terms computed from the infinite series,  is the phase,
is the phase,  is the frequency multiplier, and
is the frequency multiplier, and  is the scale factor. The number of generated points, which determines the resolution and the starting point in the plot, is also entered with sliders. You can create plots of controlled jaggedness and resolution whose morphology is different in detail. This highlights the roles of the mathematical jaggedness as represented by the fractal dimension and the resolution that in real plots is determined by practical considerations.
is the scale factor. The number of generated points, which determines the resolution and the starting point in the plot, is also entered with sliders. You can create plots of controlled jaggedness and resolution whose morphology is different in detail. This highlights the roles of the mathematical jaggedness as represented by the fractal dimension and the resolution that in real plots is determined by practical considerations.
References:
R. S. Pande, A. Jalgaonkar, and R. M. Patrikar, "A 3-D FEM Based Extractor for MEMS Inductor with Monte-Carlo Sampling," in Physics of Semiconductor Devices, IEEE Explore, 2007 pp. 710–713.
J. C. Russ, Fractal Surfaces, New York: Plenum Press, 1994 pp. 27–57.
M. Peleg, "Line Jaggedness Measures and Their Applications in Textural Evaluation of Foods," Critical Reviews in Food Science and Nutrition, 37, 1997 pp. 491–518.