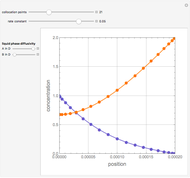
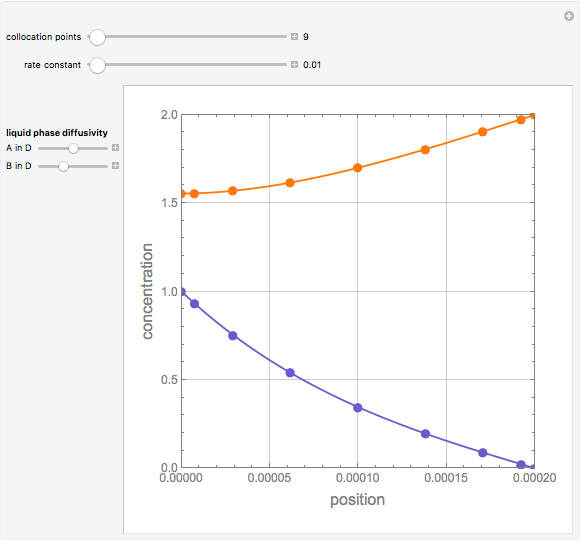
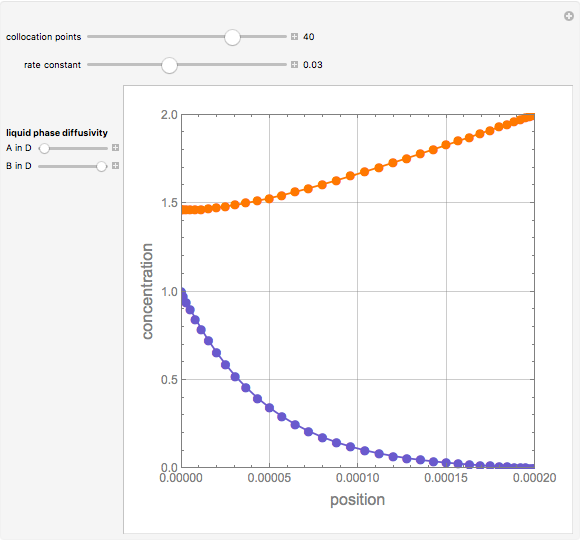
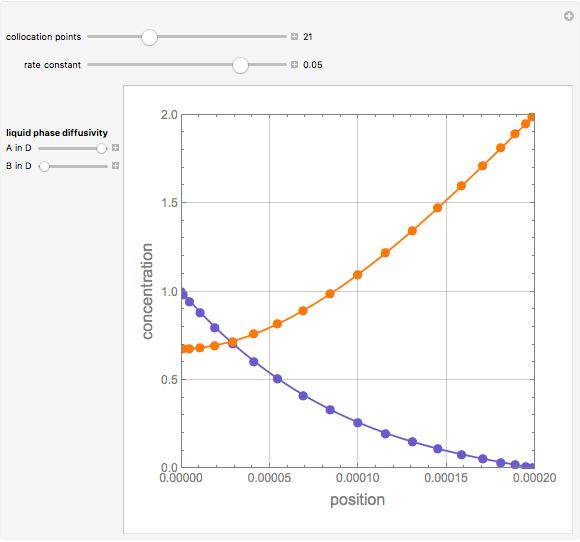
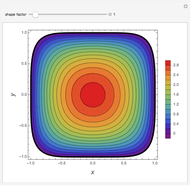
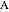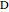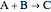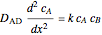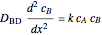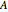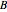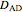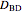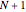Second-Order Reaction with Diffusion in a Liquid Film

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
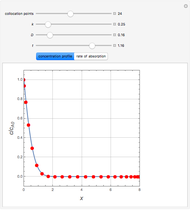
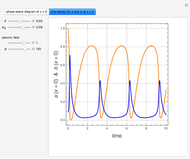
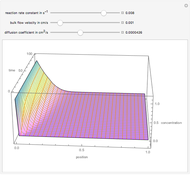
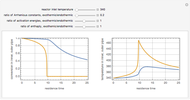
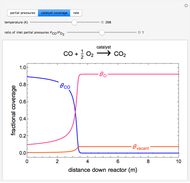
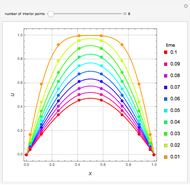
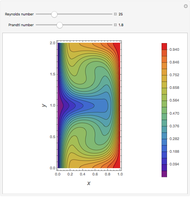
Gas absorption is often enhanced by a chemical reaction. For instance, acid gases (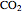 and
and 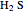 ) are usually eliminated from natural gas by absorption using ethanolamine (
) are usually eliminated from natural gas by absorption using ethanolamine (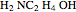 ) as a basic solvent.
) as a basic solvent.
Contributed by: Housam Binousand Brian G. Higgins (July 2013)
Open content licensed under CC BY-NC-SA
Snapshots
Details
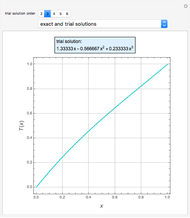
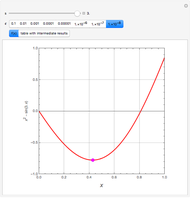
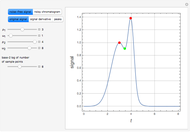
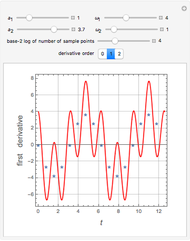
In the discrete Chebyshev–Gauss–Lobatto case, the interior points are given by 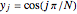 . These points are the extrema of the Chebyshev polynomials of the first kind,
. These points are the extrema of the Chebyshev polynomials of the first kind, 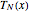 .
.
The 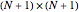 Chebyshev derivative matrix at the quadrature points is an
Chebyshev derivative matrix at the quadrature points is an 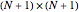 matrix
matrix 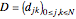 given by
given by
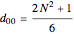 ,
, 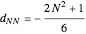 ,
, 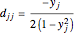 for
for 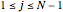 , and
, and 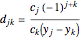 for
for 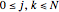 and
and 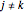 ,
,
where 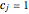 for
for 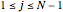 and
and 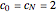 .
.
The matrix 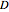 is then used as follows:
is then used as follows: 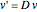 and
and 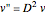 , where
, where  is a vector formed by evaluating
is a vector formed by evaluating  at
at 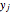 ,
, 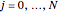 , and
, and 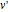 and
and 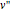 are the approximations of
are the approximations of 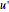 and
and 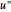 at the
at the 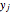 .
.
References
[1] P. Moin, Fundamentals of Engineering Numerical Analysis, Cambridge, UK: Cambridge University Press, 2001.
[2] L. N. Trefethen, Spectral Methods in MATLAB, Philadelphia: SIAM, 2000.
[3] M. B. Cutlip and M. Shacham, Problem Solving in Chemical Engineering with Numerical Methods, Upper Saddle River, NJ: Prentice Hall, 1999.
Permanent Citation