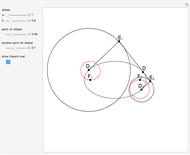
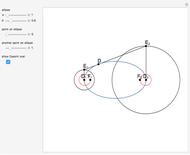
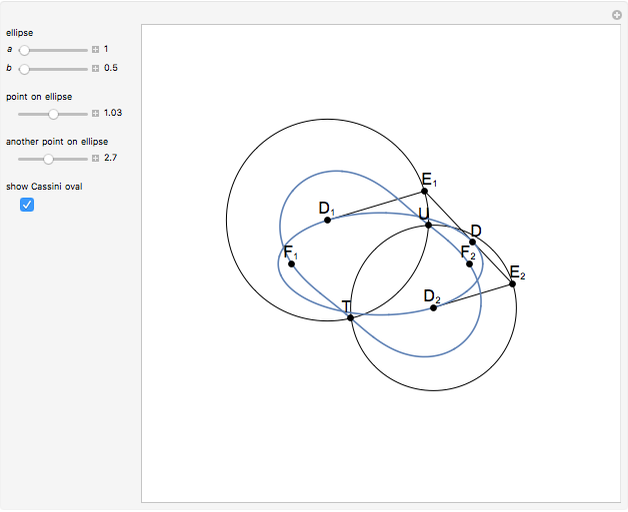
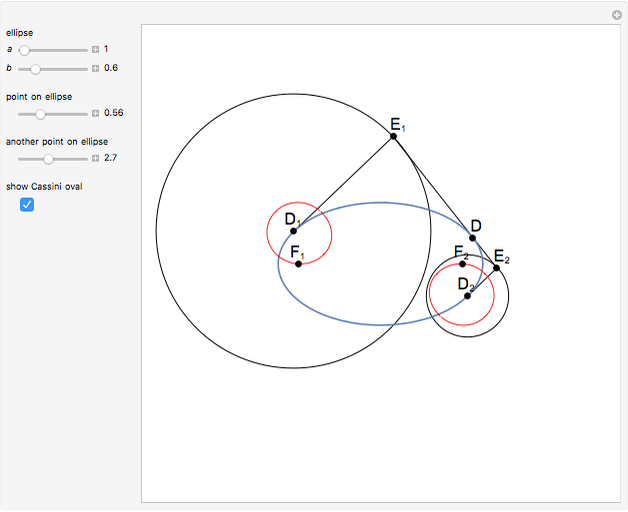
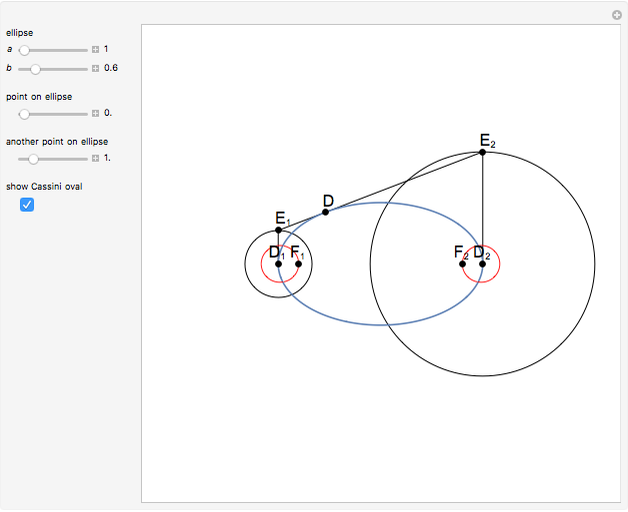
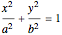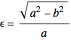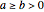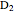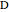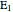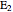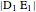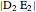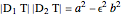3. Constructing a Point on a Cassini Oval

Initializing live version

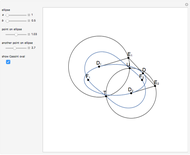
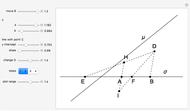
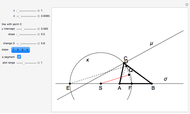
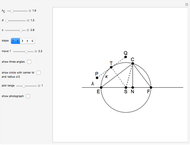
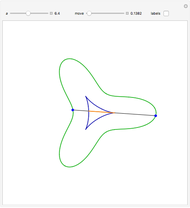
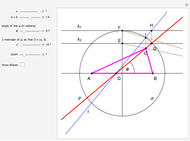
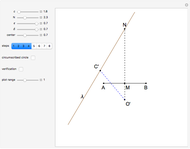
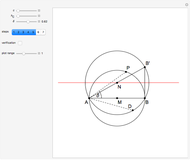
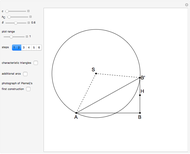
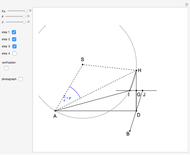
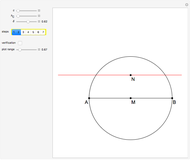
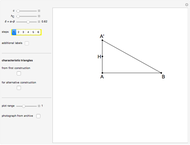
This Demonstration shows another ruler-and-compass construction of a point on a Cassini oval.

Contributed by: Marko Razpet and Izidor Hafner (July 2018)
Open content licensed under CC BY-NC-SA

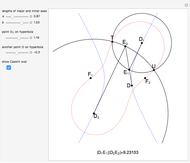
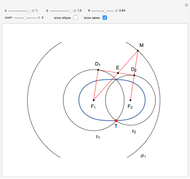
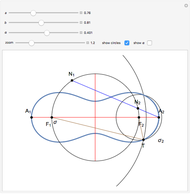
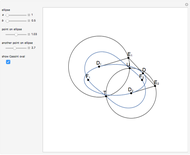
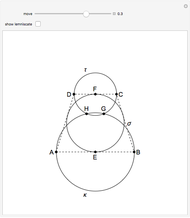
Snapshots

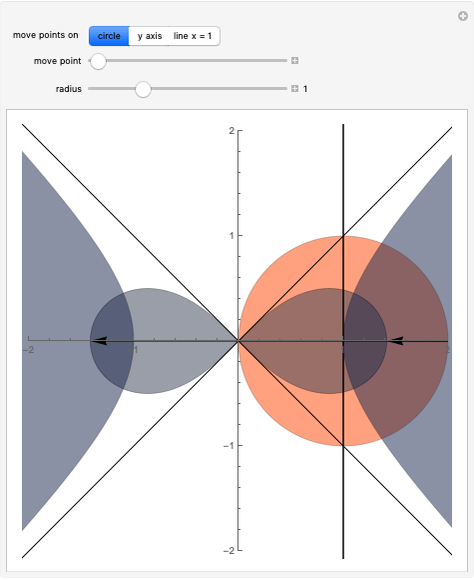
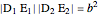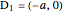In Conics, Book III, theorem 42, Apollonius showed that for and .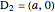

References

[1] T. Heath, A History of Greek Mathematics, Volume II: From Aristarchus to Diophantus, New York: Dover Publications, 1981 p. 155.

[2] A. Ostermann and G. Wanner, Geometry by Its History, New York: Springer, 2012 pp. 76–78.


Feedback (field required)

Email (field required)	Name

Occupation	Organization

Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback. Send