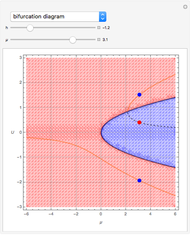
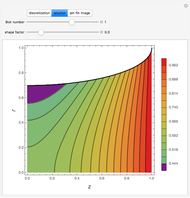
Bifurcation Diagram for the Three-Variable Autocatalator

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
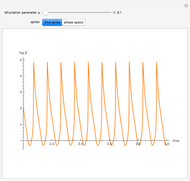
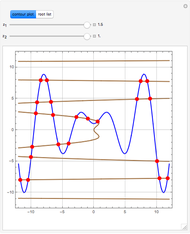
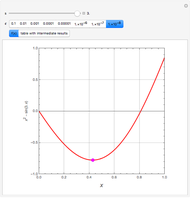
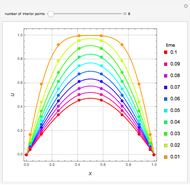
This Demonstration shows how one can compute the bifurcation diagram for a nonlinear chemical system such as the three-variable autocatalator (see Details).
[more]
Contributed by: Housam Binous, Zakia Nasri, and Brian G. Higgins (April 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Consider a chemical reaction such as the shift conversion:  . To study its chemical kinetics, it is written in abstract form as
. To study its chemical kinetics, it is written in abstract form as  . The rate of this reaction is given by
. The rate of this reaction is given by  , where
, where  is the rate constant and
is the rate constant and  ,
,  , and
, and  are the concentrations of the species
are the concentrations of the species  ,
,  , and
, and  .
.
Hypothetically, the autocatalator's steps are the following:
 Here
Here  is a precursor with constant concentration,
is a precursor with constant concentration,  is the final product,
is the final product,  ,
,  , and
, and  are intermediate chemical species,
are intermediate chemical species,  ,
,  ,
,  ,
,  ,
,  , and
, and  are rate constants for the individual reactions, and
are rate constants for the individual reactions, and  ,
,  ,
,  ,
,  , and
, and  are the concentrations of the hypothetical chemical species
are the concentrations of the hypothetical chemical species  ,
,  ,
,  ,
,  , and
, and  .
.
This hypothetical reaction scheme is a model for a three-variable autocatalator. The autocatalytic reaction occurs in the step  , with
, with  catalyzing its own formation. This step introduces the nonlinear term
catalyzing its own formation. This step introduces the nonlinear term  in the governing equations, necessary in order to obtain sufficient complexity for chaos to occur.
in the governing equations, necessary in order to obtain sufficient complexity for chaos to occur.
The rate equations for the three intermediate species can be written in the form:
 ,
,
 ,
,
 .
.
The corresponding dimensionless rate equations are:
 ,
,
 ,
,
 ,
where
,
where  ,
,  , and
, and  are the dimensionless concentrations of
are the dimensionless concentrations of  ,
,  , and
, and  , and the four parameters
, and the four parameters  ,
,  ,
,  , and
, and  depend on the rates of the individual reactions and the concentration of the precursor.
depend on the rates of the individual reactions and the concentration of the precursor.
This is the bifurcation diagram:

Reference
[1] B. Peng, S. K. Scott, and K. Showalter, "Period Doubling and Chaos in a Three-Variable Autocatalator," The Journal of Physical Chemistry, 94(13), 1990 pp. 5243–5246.
Permanent Citation