Gershgorin Circle Theorem

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
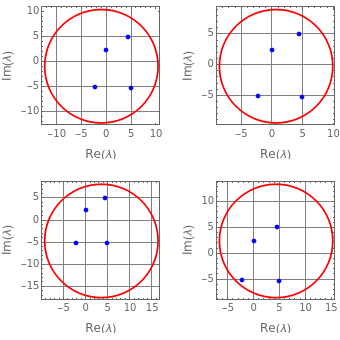
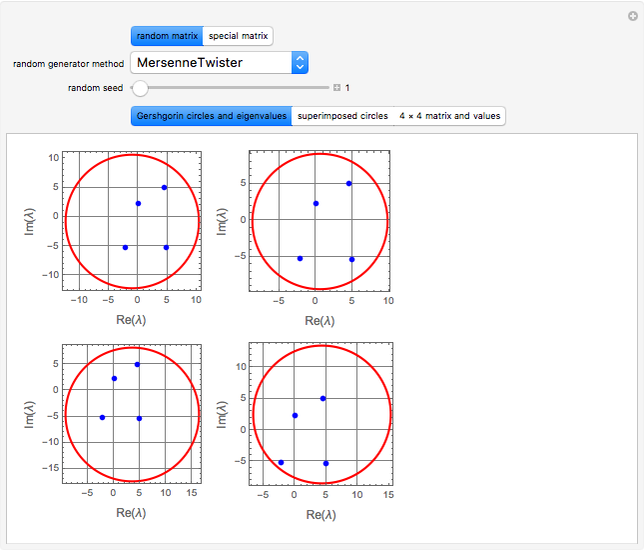
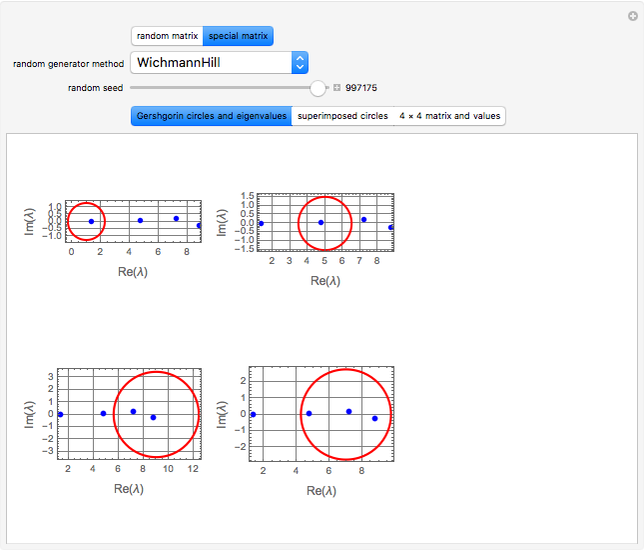
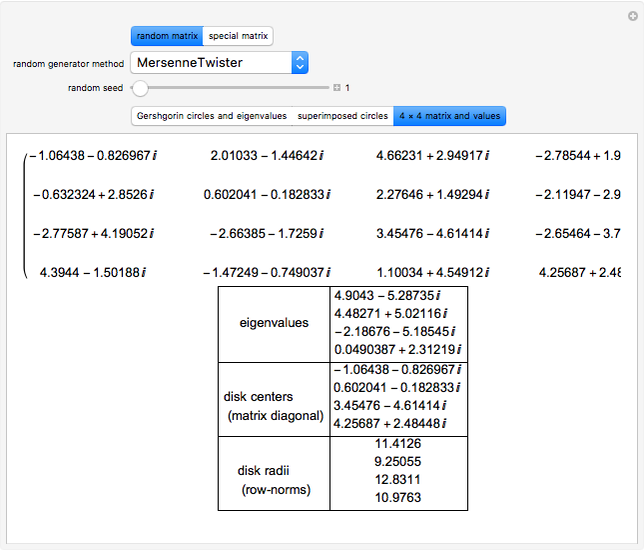
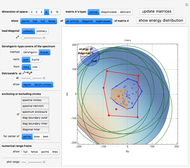
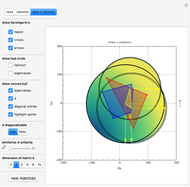
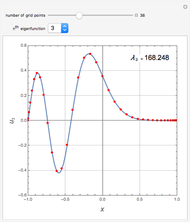
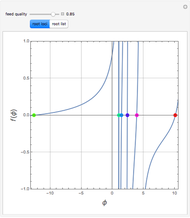
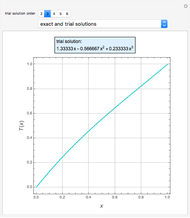
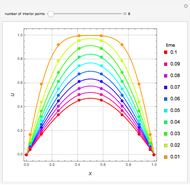
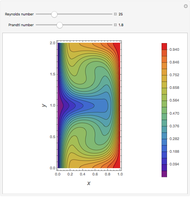
This Demonstration illustrates how the Gershgorin circle theorem can be used to bound the eigenvalues of an  matrix
matrix  . The theorem states that the eigenvalues of
. The theorem states that the eigenvalues of  must lie in circles
must lie in circles  defined in the complex plane
defined in the complex plane  that are centered on the diagonal elements of
that are centered on the diagonal elements of  with radii
with radii  determined by the row-norms of
determined by the row-norms of  , that is,
, that is,  and
and  . If
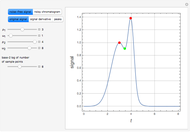
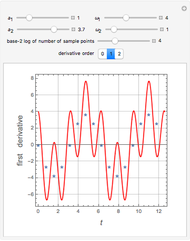
. If  of the circles
of the circles  form a connected region
form a connected region  disjoint from the remaining
disjoint from the remaining  circles, then the region
circles, then the region  contains exactly
contains exactly  eigenvalues.
eigenvalues.
Contributed by: Housam Binous and Brian G. Higgins (April 2012)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Reference
[1] B. Noble, Applied Linear Algebra, New Jersey: Prentice–Hall, 1969.
Permanent Citation