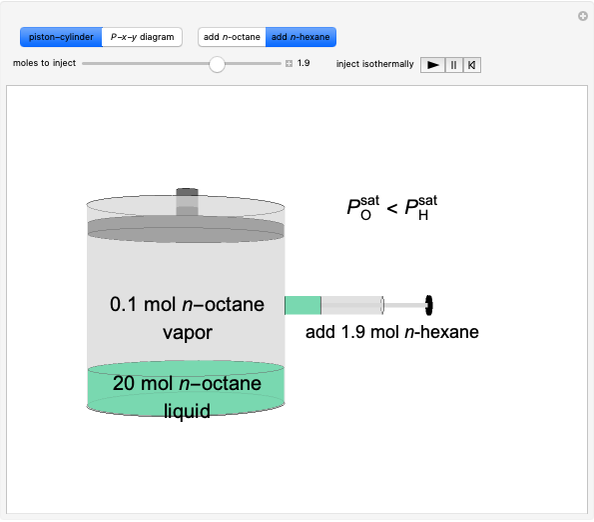
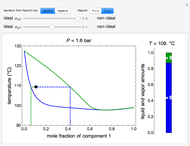
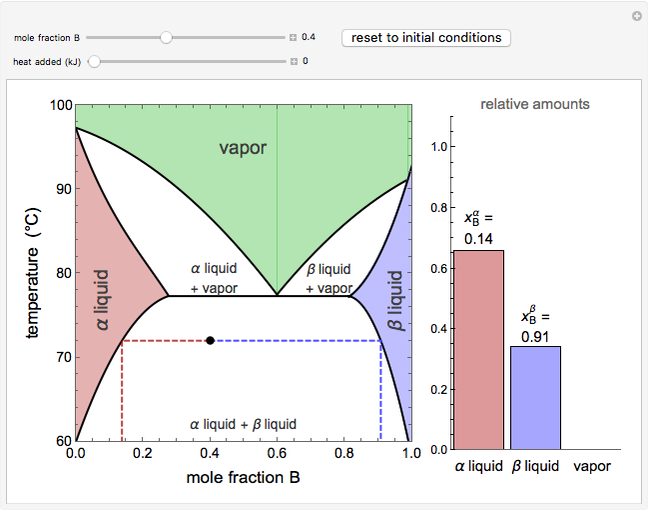
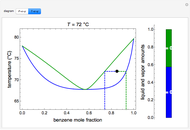
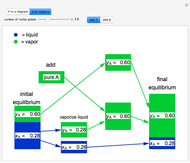
This Demonstration illustrates what happens to a binary mixture, initially in vapor-liquid equilibrium, when a pure component is added at constant temperature and pressure. If both phases remain after the addition, it illustrates how the phase mole fractions remain constant ( is the mole fraction of in the liquid, is the mole fraction of in the vapor). If enough of either pure component is added, the mixture is transformed to a single phase. The initial vapor-liquid mixture contains 2 mol of and 2 mol of at a pressure of 3 bar. 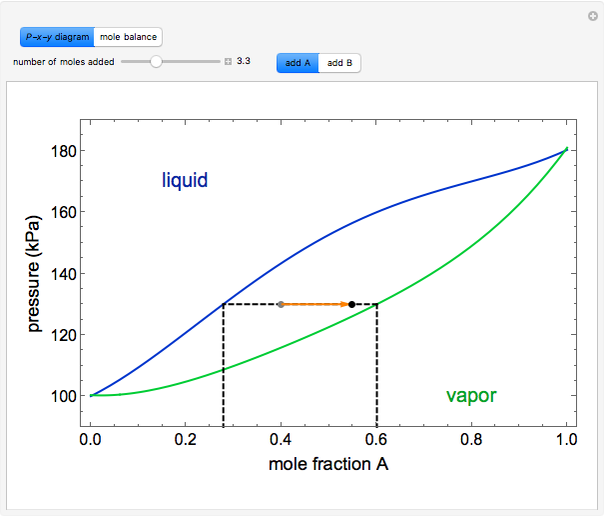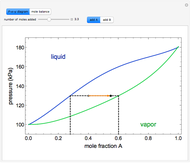Select the button "add " or "add " and use the slider to set the amount added. Adding a pure component changes the overall mole fraction of in the mixture, as represented by the black point on the -- diagram. 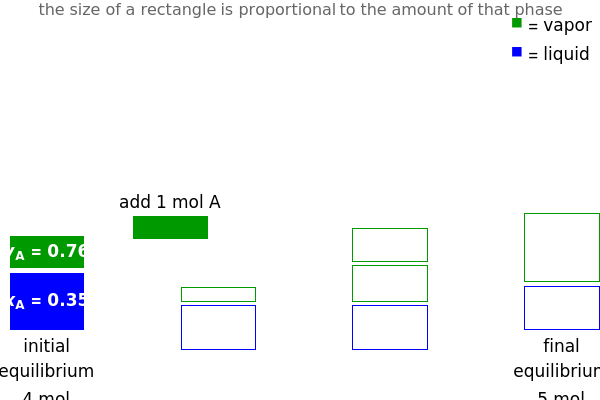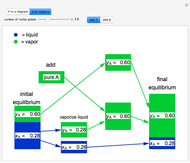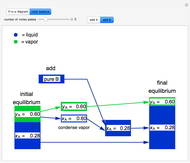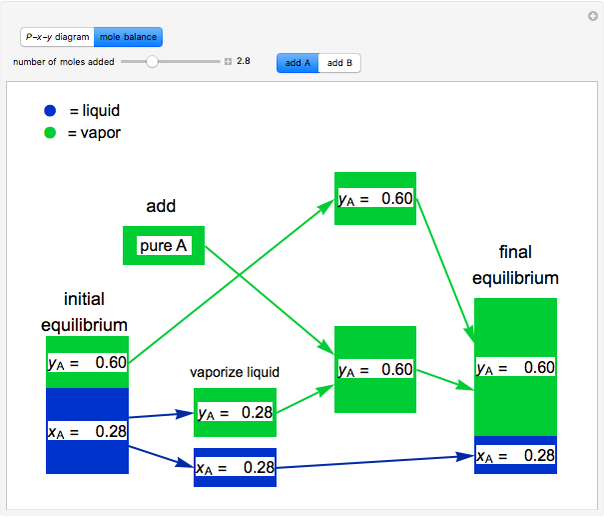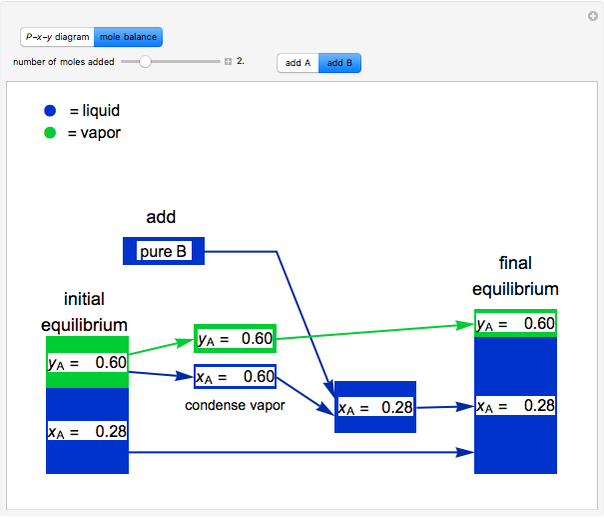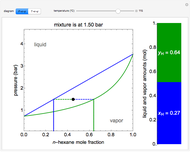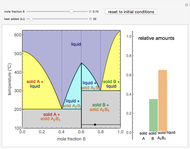If the overall mole fraction of stays within the phase envelope, then and do not change. Select "mole balance" to see how this is possible. The size of a rectangle is proportional to the amount of that phase (blue is liquid, green is vapor). For example, adding increases the vapor-to-liquid ratio, so some of the initial liquid vaporizes, and this amount is determined by a mass balance. For ease of visualization, is shown being added as a vapor and as a liquid, but the final equilibrium is the same if is added as a liquid and as a vapor because the system is isothermal. For example, if pure were added as a liquid, it would vaporize to satisfy the mass balances.