The Return Distribution of the Variance Gamma Process
Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
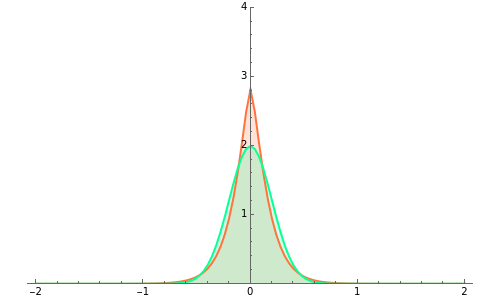
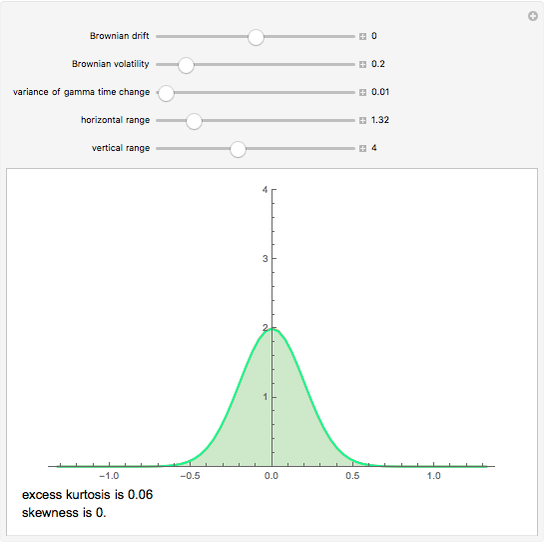
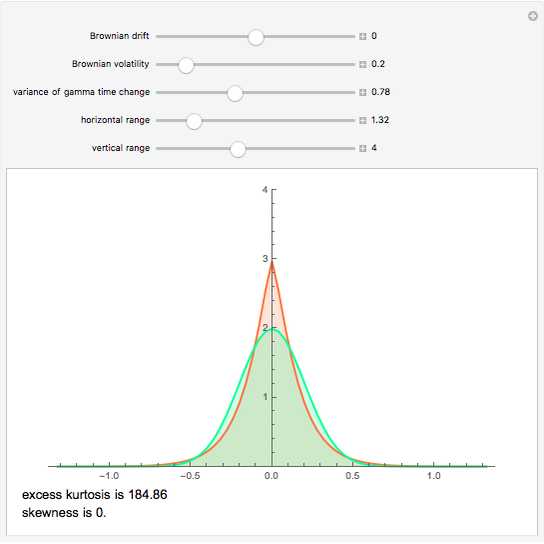
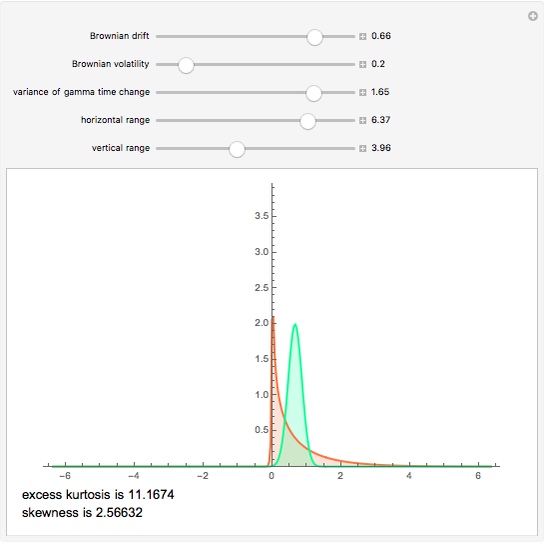
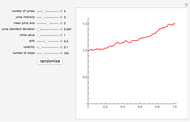
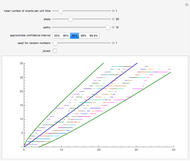
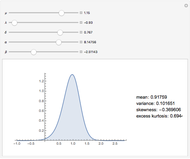
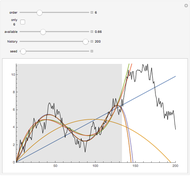
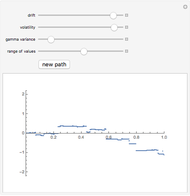
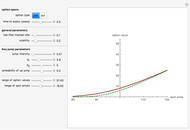
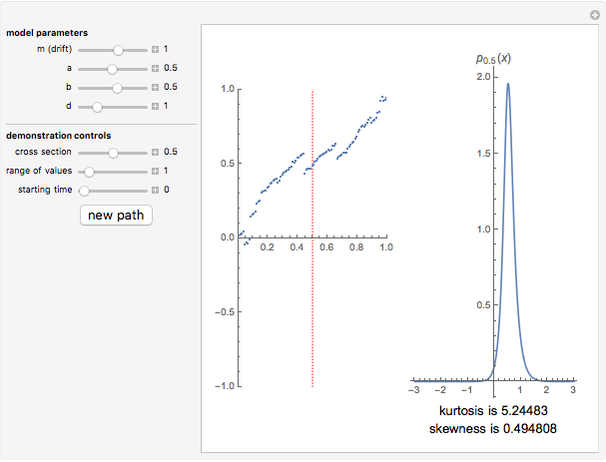
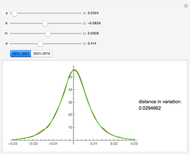
This Demonstration shows the graphs of the density function of the unit period of a variance gamma process (red) and a Brownian motion process with drift (green). The variance gamma process is a three-parameter stochastic process that generalizes Brownian motion and was developed as a model for the dynamics of log stock prices. The process can be constructed as a Brownian motion with drift evaluated at random times given by a gamma process. The process thus has three parameters: the drift and volatility of the Brownian motion and the volatility of the gamma time change. Together they make it possible to control the skewness and kurtosis of the return distribution, which makes it possible to correct for the well-known biases of the Black–Scholes model (based on Brownian motion).
Contributed by: Andrzej Kozlowski (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
The variance gamma process is a high-activity pure-jump Lévy process—that is, unlike for example the Merton Jump Diffusion Process—it does not contain a continuous martingale component. It has an infinite number of jumps in any finite interval of time, but only finitely many of them are larger than any specified positive real number. The exponential variance gamma model has been shown to perform better in modelling stock returns than the Black–Scholes model.
[1] D. B. Madan and E. Seneta, "The Variance Gamma Process (V.G.) Model for Share Market Returns," Journal of Business, 63(4), 1990 pp. 511–524.
[2] D. B. Madan, P. P. Carr, and E. C. Chang, "The Variance Gamma Model and Option Pricing," European Finance Review, 2(1), 1998 pp. 79–105.
[3] K. Lam, E. Chang, and M. C. Lee, "An Empirical Test of the Variance Gamma Option Pricing Model," Pacific Basin Finance Journal, 10(3), 2002 pp. 267–285.
Permanent Citation
"The Return Distribution of the Variance Gamma Process"
http://demonstrations.wolfram.com/TheReturnDistributionOfTheVarianceGammaProcess/
Wolfram Demonstrations Project
Published: March 7 2011
Correlated Wiener Processes
Andrzej Kozlowski
Two Jump Diffusion Processes
Andrzej Kozlowski
The Poisson Process
Andrzej Kozlowski
Merton's Jump Diffusion Model
Andrzej Kozlowski
Brownian Bridge
Andrzej Kozlowski
Correlated Gamma Variance Processes with Common Subordinator
Andrzej Kozlowski
Simulating the Poisson Process
Heikki Ruskeepää
Generalized Hyperbolic Distribution
Andrzej Kozlowski
Polynomial Fits of Random Walks
Michael Schreiber
The Variance Gamma Process
Andrzej Kozlowski
-
The Black-Scholes European Call Option Formula Corrected Using the Gram-Charlier Expansion
Andrzej Kozlowski -
Option Prices in the Kou Jump Diffusion Model
Andrzej Kozlowski -
The Polar and Bipolar of a Convex Polytope
Andrzej Kozlowski -
Two Jump Diffusion Processes
Andrzej Kozlowski -
The Vieta Mapping for the Coxeter Group A_2
Andrzej Kozlowski -
The Bifurcation Set of the Space of Smooth Real Functions
Andrzej Kozlowski -
The Swallowtail Singularity
Andrzej Kozlowski -
Singularities of an Ellipsoidal Wave Front
Andrzej Kozlowski -
Nonuniqueness of Option Pricing Under the Meixner Model
Andrzej Kozlowski -
Coin Machine
Andrzej Kozlowski -
A Mean-Reverting Jump Diffusion Process
Andrzej Kozlowski -
Standard American and European Options
Andrzej Kozlowski -
Density of the Kou Jump Diffusion Process
Andrzej Kozlowski -
The Meixner Process
Andrzej Kozlowski -
Fitting the Meixner Distribution to S&P 500 Returns
Andrzej Kozlowski -
Simple Graphs and Their Binomial Edge Ideals
Andrzej Kozlowski -
The Eneström-Kakeya Bounds for Roots of a Polynomial with Positive Coefficients
Andrzej Kozlowski -
Newton Polygon and Branching of Algebraic Curves
Andrzej Kozlowski -
Counting the Number of Roots of Transcendental Functions in Bounded Regions Using Winding Numbers
Andrzej Kozlowski -
The Space of Inner Products
Andrzej Kozlowski