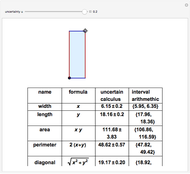
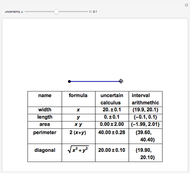
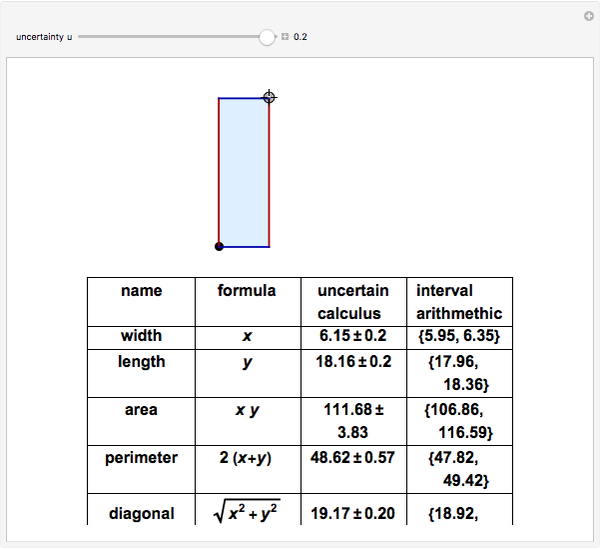
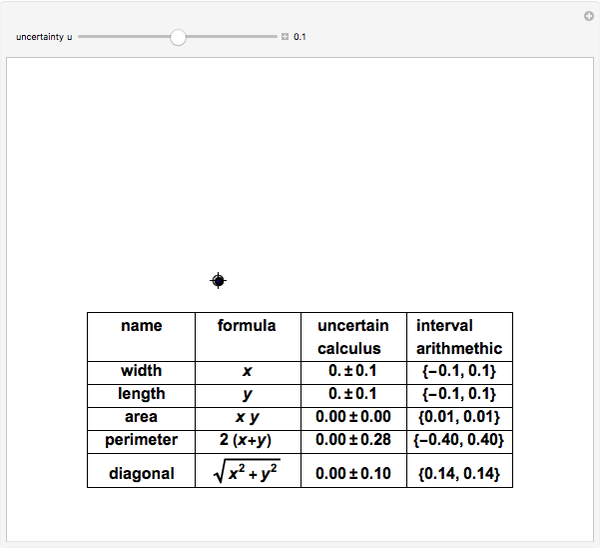
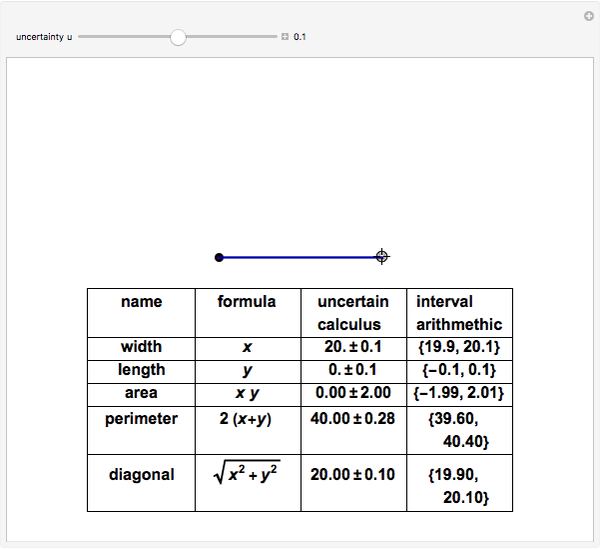
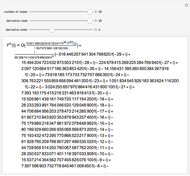
Area, Perimeter, and Diagonal of a Rectangle with Uncertain Sides

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
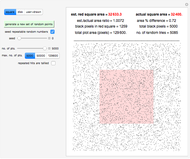
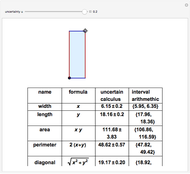
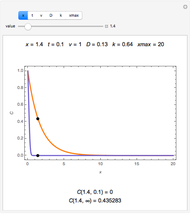
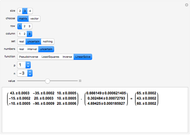
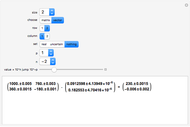
Using the uncertain calculus [1] and interval arithmetic, simple analytical solutions are derived and used to calculate the area, perimeter, and diagonal of a rectangle with the bottom left corner at the origin and the top right corner 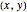 defined by dragging the locator. The slider value
defined by dragging the locator. The slider value  is added as an uncertain part to
is added as an uncertain part to 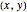 .
.
Contributed by: Valter Yoshihiko Aibe and Mikhail Dimitrov Mikhailov (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
There is international consensus on the evaluation of standard uncertainty and combined standard uncertainty in measurement and computations [1, 2]. Just as the International System of Units (SI) has brought coherence to all scientific and technological measurements, the worldwide consensus on uncertainty permits a vast spectrum of results to be readily understood and properly interpreted. The needs of numerous testing laboratories to calculate the measurement uncertainty on a large scale is supported by numerous software tools. Rasmussen [3] refers to 10 different software packages.
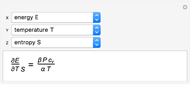
To facilitate the calculation of the combined standard uncertainty, Aibe and Mikhailov introduced a new object 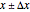 called an uncertain number and developed in Mathematica an Uncertain Calculus package [4]. It is based on two assumptions: the functions manipulated have attribute NumericFunction and the relations Greater, GreaterEqual, Less, LessEqual, SameQ, Unequal, UnsameQ are independent of the uncertainties. The package transforms any functional relationship of uncertain numbers into an uncertain number, by using the general law of uncertainty propagation:
called an uncertain number and developed in Mathematica an Uncertain Calculus package [4]. It is based on two assumptions: the functions manipulated have attribute NumericFunction and the relations Greater, GreaterEqual, Less, LessEqual, SameQ, Unequal, UnsameQ are independent of the uncertainties. The package transforms any functional relationship of uncertain numbers into an uncertain number, by using the general law of uncertainty propagation:
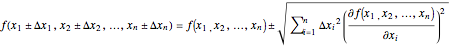 .
.
The traditional uncertainty concept focuses on unknowable error and true value using the so-called general law of error propagation. The new concept is based on the estimated value and uncertainty using the same law of uncertainty propagation. Only the interpretation is different.
The rules extracted from the Uncertain Calculus package [5] and lists have been used to derive analytical solutions for uncertain and interval area, perimeter, and diagonal. A version of this Demonstration using uncertain calculus rules, interval arithmetic, and the derived analytical solutions has been explored to verify that both approaches give the same results. The present version only uses the analytical solutions.
References:
[1] European Cooperation for Accreditation of Laboratories, Expression of the Uncertainty of Measurement in Calibration, EAL-R2 and EAL-R2-S1, 1997.
[2] International Organization for Standardization, Guide to the Expression of Uncertainty in Measurement, Geneva, Switzerland: ISO, 1995.
[3] S. N. Rasmussen, "Software Tools for the Expression of Uncertainty in Measurement," MetroTrade Workshop on Traceability and Measurement Uncertainty in Testing, Berlin, January 30–31, 2003.
[4] V. Y. Aibe and M. D. Mikhailov, "Uncertainty Calculus in Metrology," Proceedings of ENCIT 2008, 12th Brazilian Congress of Thermal Engineering and Sciences, Belo Horizonte, MG, Brazil, November 10–14, 2008.
[5] "Solving Matrix Systems with Real, Interval, or Uncertain Elements" from The Wolfram Demonstrations Project
Permanent Citation