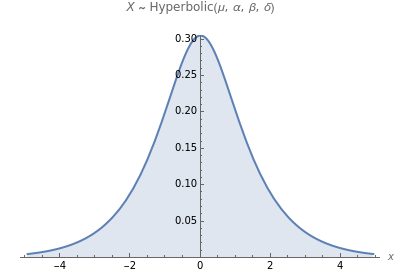
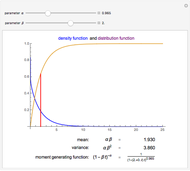
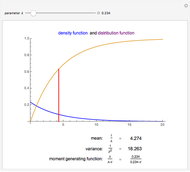
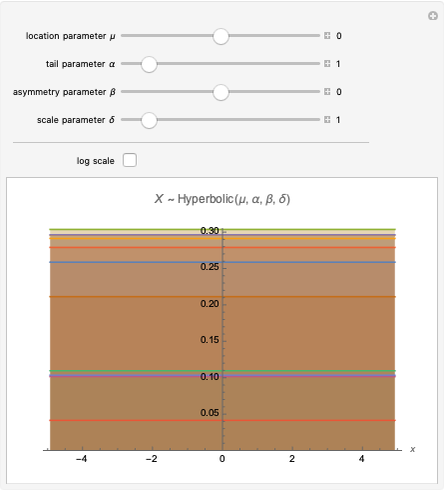
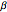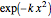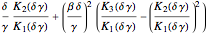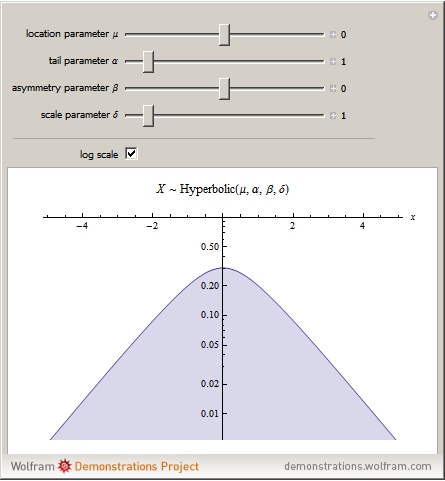
In this Demonstration we visualize the probability density function of the hyperbolic distribution, which has parameters (location), (tail), (asymmetry), and (scale). These are all real-valued, with the additional constraint that 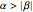. This distribution has "semi-heavy" tails and has appeared in a diverse range of applications, including models of asset returns in financial markets and sand pile formation. The word "hyperbolic" is used because the log of its probability density function is a hyperbola; this can be seen by clicking the "log scale" checkbox.
The probability density function for the hyperbolic distribution is given by
,
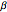where 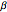and is the modified Bessel function of the second kind. It has mean 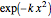and variance .
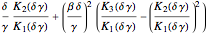As 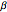, the probability density decays exponentially like . This is intermediate between the behavior of the normal distribution, which decays more rapidly (like 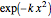), and the more extreme "fat tail" behavior of power-law distributions. For this reason, it is sometimes referred to as a "semi-heavy tailed" distribution.
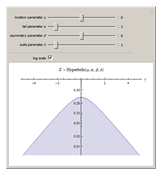
Snapshot 1: on a vertical log scale the distribution has a hyperbolic shape
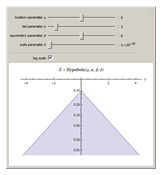
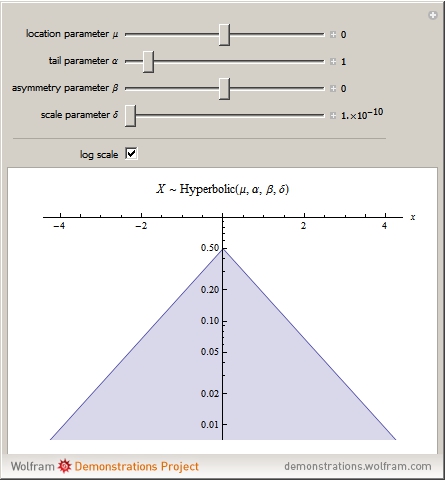
Snapshot 2: as 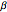, the hyperbola degenerates into a piecewise linear form
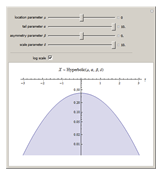
Snapshot 3: as 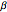, the hyperbola becomes a parabola
Snapshot 4: for non-zero 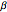, the distribution is asymmetric
![[Snapshot]](HTMLImages/index.en/thumbnail_1.gif)
![[Snapshot]](HTMLImages/index.en/thumbnail_2.gif)
![[Snapshot]](HTMLImages/index.en/thumbnail_3.gif)
![[Snapshot]](HTMLImages/index.en/thumbnail_4.gif)
Embed Interactive Demonstration 
Browse all topics
