Haar Functions
Initializing live version

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
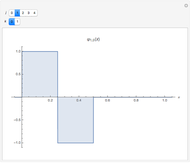
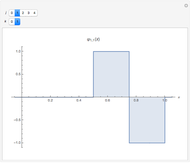
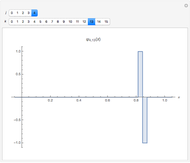
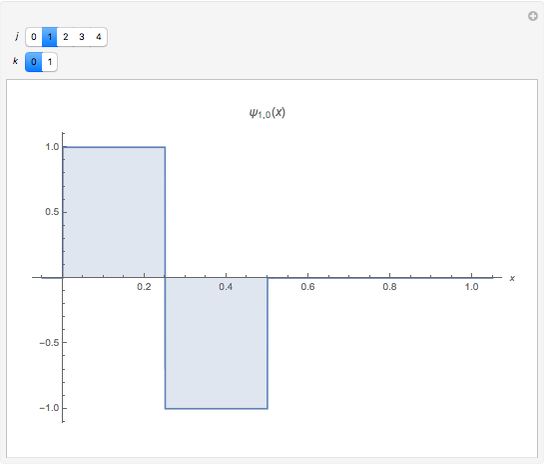
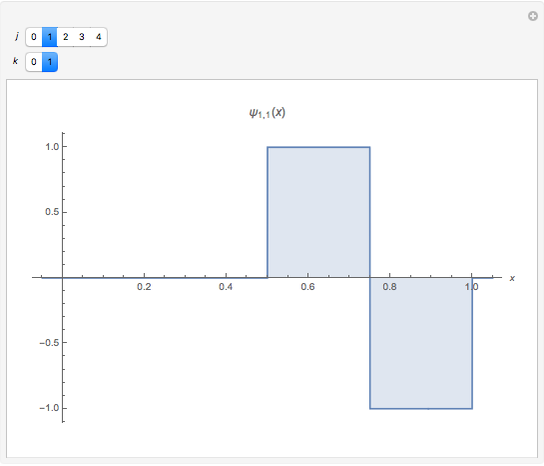
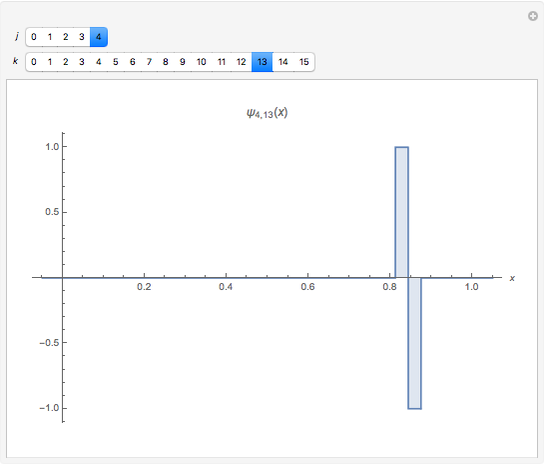
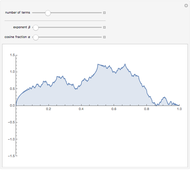
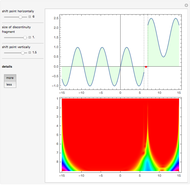
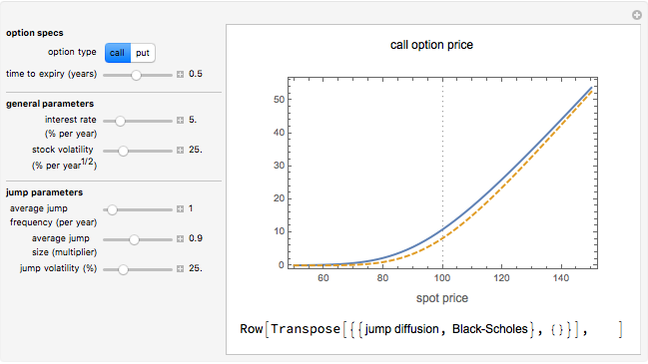
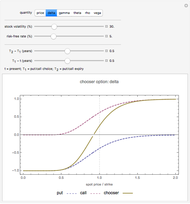
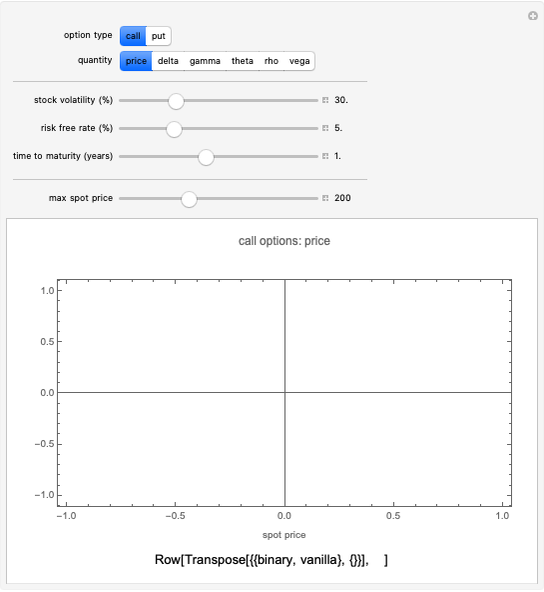
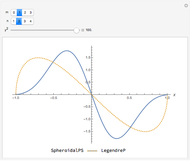
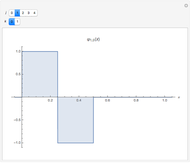
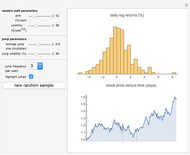
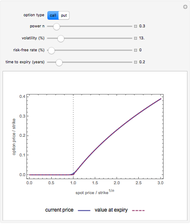
This Demonstration shows the Haar functions 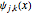 , defined on the unit interval
, defined on the unit interval 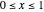 for integers
for integers 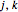 , where
, where 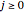 and
and 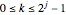 . They form a complete orthogonal set on
. They form a complete orthogonal set on 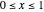 and constitute the simplest type of wavelet.
and constitute the simplest type of wavelet.
Contributed by: Peter Falloon (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
The Haar functions may be defined with respect to the "mother wavelet" 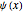 , defined by
, defined by
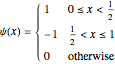
For integers 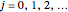 and
and 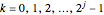 , the Haar functions are then defined as
, the Haar functions are then defined as 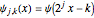 .
.
Permanent Citation