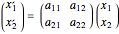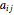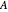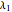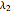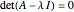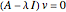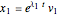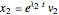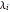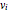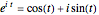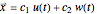Using Eigenvalues to Solve a First-Order System of Two Coupled Differential Equations
Initializing live version

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
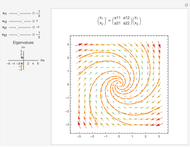
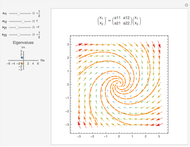
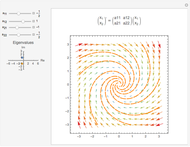
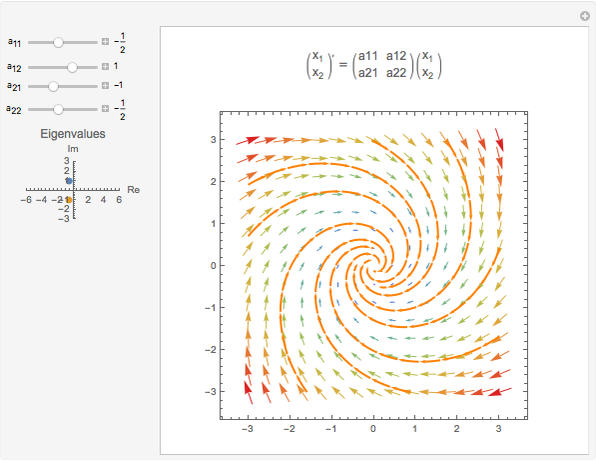
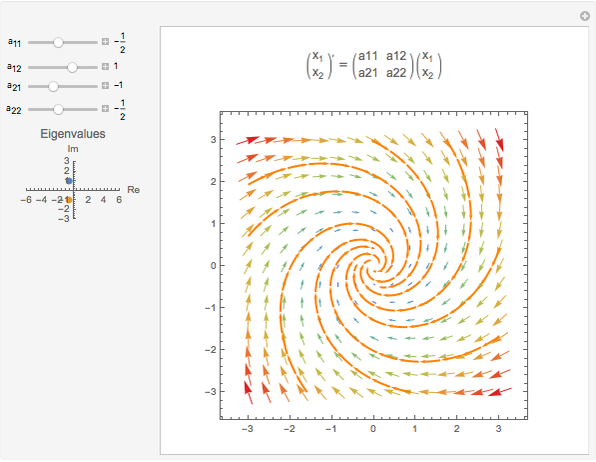
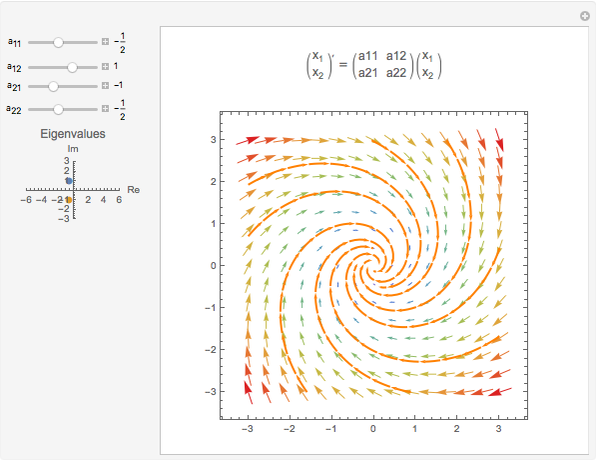
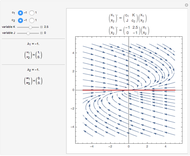
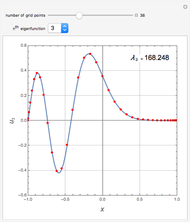
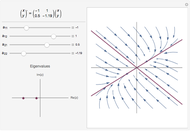
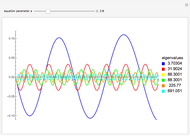
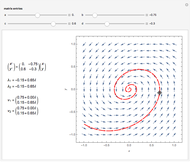
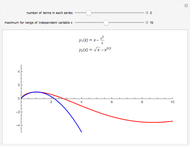
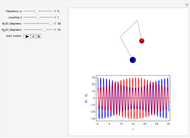
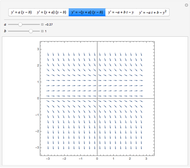
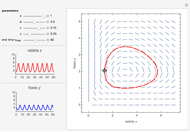
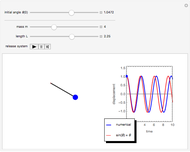
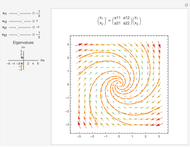
Consider the coupled system of differential equations
[more]
Contributed by: Stephen Wilkerson (March 2011)
(United States Military Academy West Point, Department of Mathematics)
Open content licensed under CC BY-NC-SA
Snapshots
Details
This is the complex eigenvalue example 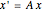 from [1], Section 3.4, Modeling with First Order Equations.
from [1], Section 3.4, Modeling with First Order Equations.
Reference
[1] J. R. Brannan and W. E. Boyce, Differential Equations with Boundary Value Problems: An Introduction to Modern Methods and Applications, New York: John Wiley and Sons, 2010.
Permanent Citation