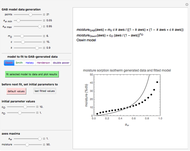
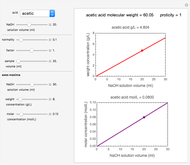
Dehydration by a Desiccant

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
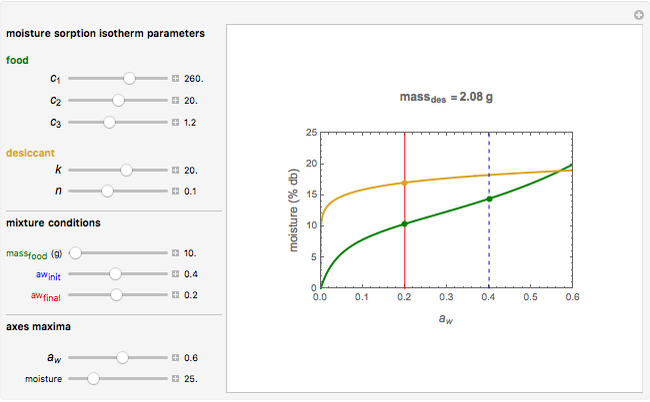
This Demonstration estimates the amount of desiccant needed to lower the water activity, 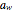 , of a food or pharmaceutical powder. The powder's moisture sorption isotherm is described by a three-parameter generic BET/GAB model, and that of the desiccant by a two-parameter power model. These equations' parameters are determined by fitting experimental sorption data. The Demonstration solves a mass balance equation, based on the powder's and desiccant's moisture sorption isotherms, the powder's initial wet mass, initial
, of a food or pharmaceutical powder. The powder's moisture sorption isotherm is described by a three-parameter generic BET/GAB model, and that of the desiccant by a two-parameter power model. These equations' parameters are determined by fitting experimental sorption data. The Demonstration solves a mass balance equation, based on the powder's and desiccant's moisture sorption isotherms, the powder's initial wet mass, initial 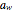 , and the desired final
, and the desired final 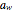 . It is assumed that the system is closed, the temperature is constant, the desiccant is added bone dry, and any moisture in the free space is negligible.
. It is assumed that the system is closed, the temperature is constant, the desiccant is added bone dry, and any moisture in the free space is negligible.
Contributed by: Mark D. Normand and Micha Peleg (July 2012)
Open content licensed under CC BY-NC-SA
Snapshots
Details
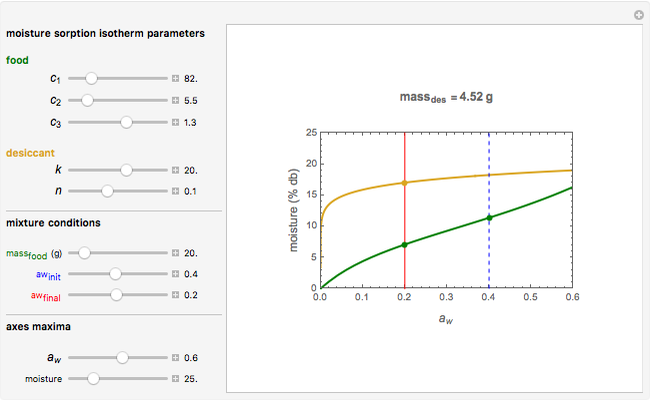
Snapshot 1: desiccation of 10 g of a protein
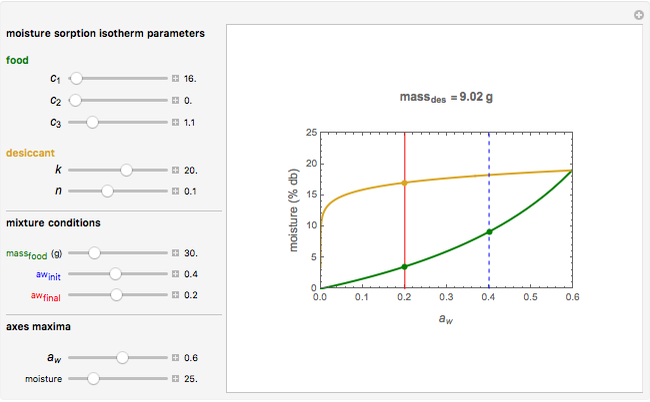
Snapshot 2: desiccation of 20 g of a starch with the same desiccant
Snapshot 3: desiccation of 30 g of dried fruit with the same desiccant
Low water activity, 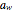 , is a key to the physical, chemical, and biological stability of food and pharmaceutical powders. Given sufficient time, when two powders having different water activities are part of a closed system, such as a sealed container, moisture will be exchanged until both components reach an equilibrium
, is a key to the physical, chemical, and biological stability of food and pharmaceutical powders. Given sufficient time, when two powders having different water activities are part of a closed system, such as a sealed container, moisture will be exchanged until both components reach an equilibrium 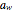 . The presence of a dry desiccant can therefore reduce the water activity of powders by absorbing part of their moisture. The process is governed by the mass balance equation
. The presence of a dry desiccant can therefore reduce the water activity of powders by absorbing part of their moisture. The process is governed by the mass balance equation 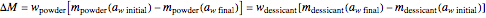 , where
, where 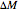 is the mass of water exchanged;
is the mass of water exchanged;  is the powder's dry mass and
is the powder's dry mass and 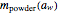 its moisture sorption isotherm equation; and
its moisture sorption isotherm equation; and 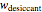 is the desiccant's dry mass and
is the desiccant's dry mass and 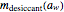 its moisture sorption isotherm equation. When the desiccant is added bone dry
its moisture sorption isotherm equation. When the desiccant is added bone dry  ),
), 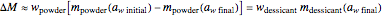 . The dry mass
. The dry mass  at the initial water activity,
at the initial water activity,  , can be calculated by the formula
, can be calculated by the formula 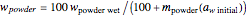 . The amount of desiccant needed to lower a powder's water activity from
. The amount of desiccant needed to lower a powder's water activity from  to
to 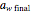 can be calculated by solving the mass balance equation for
can be calculated by solving the mass balance equation for 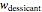 .
.
In this Demonstration, the powder's moisture sorption isotherm is in the form 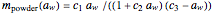 , where the
, where the 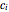 , which can be found by fitting experimental sorption data, are entered with sliders. The desiccant's moisture sorption isotherm is in the form
, which can be found by fitting experimental sorption data, are entered with sliders. The desiccant's moisture sorption isotherm is in the form 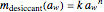 , where
, where  and
and  are similarly determined and entered.
are similarly determined and entered.
Upon entering the powder's and desiccant's moisture sorption equation's coefficients, the powder's mass, and the initial and desirable final 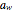 , the Demonstration displays the two moisture sorption isotherm curves and calculates and displays the amount of desiccant needed,
, the Demonstration displays the two moisture sorption isotherm curves and calculates and displays the amount of desiccant needed, 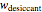 , assuming that it is added bone dry. The calculation is based on the assumptions that the system is closed, the temperature is practically constant, and any moisture present in the interparticle space is negligible relative to the amount exchanged. Notice that the mass balance equation on which the calculation is based provides no information about how long it will take for the process to approach equilibrium.
, assuming that it is added bone dry. The calculation is based on the assumptions that the system is closed, the temperature is practically constant, and any moisture present in the interparticle space is negligible relative to the amount exchanged. Notice that the mass balance equation on which the calculation is based provides no information about how long it will take for the process to approach equilibrium.
References
[1] H. A. Iglesias and J. Chirife, Handbook of Food Isotherms: Water Sorption Parameters for Food and Food Components, New York: Academic Press, 1982.
[2] M. Peleg and M. D. Normand, "Estimation of the Equilibrium Water Activity of Multicomponent Mixtures," Trends in Food Science & Technology, 3, 1992 pp. 157–160. www.sciencedirect.com/science/article/pii/092422449290177X.
[3] W. Wolf, W. E. L. Spiess, and G. Jung, Sorption Isotherms and Water Activity of Food Materials, New York: Elsevier, 1985.
[4] M. Peleg. Calculating the Weight of Desiccant or Moistener to Adjust a Dry Mixture's Water Activity. (Jul 9, 2012) people.umass.edu/aew2000/WaterAct/DesMoi.html.
[5] M. Peleg. Calculating Water Activity of Dry Food Mixtures Using Mathematica or Excel. (Jul 9, 2012) people.umass.edu/aew2000/WaterAct/wateractivity.html.
[6] M. Peleg."Calculating Water Activity of Dry Food Mixtures." (Jul 9, 2012) people.umass.edu/aew2000/WaterAct/Mathematica/mathwateract.html.
Permanent Citation