Weibullian Chemical Degradation

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
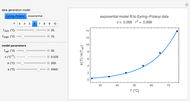
This Demonstration shows isothermal and selected dynamic chemical degradation patterns that follow the Weibullian ("stretched exponential") model with a constant shape factor (exponent). The temperature dependence of the rate parameter is described by a simple exponential model which, as has been previously shown, can be used interchangeably with the Arrhenius equation.
Contributed by: Mark D. Normandand Micha Peleg (September 2016)
Open content licensed under CC BY-NC-SA
Snapshots
Details
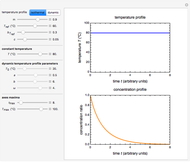
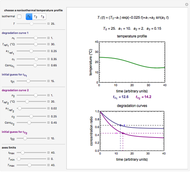
Snapshot 1: isothermal degradation curve following the Weibullian model
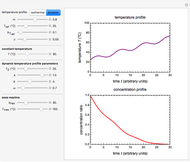
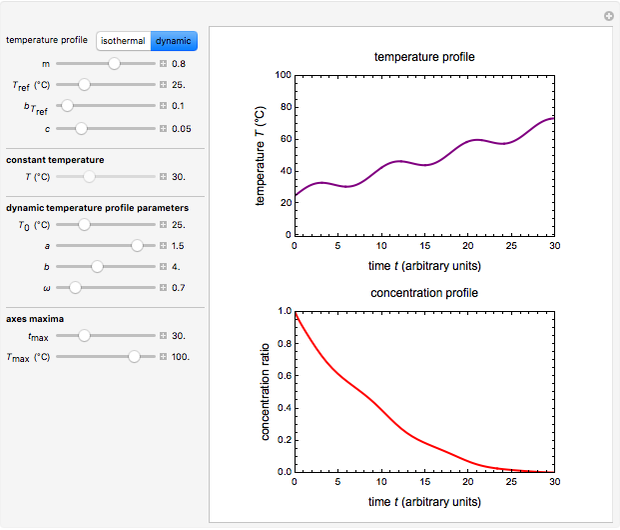
Snapshot 2: nonisothermal degradation curve following the Weibullian model (rising oscillating temperature)
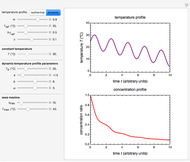
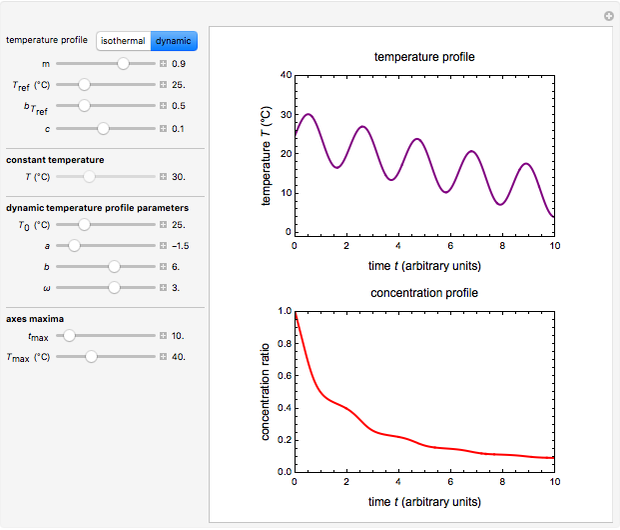
Snapshot 3: nonisothermal degradation curve following the Weibullian model (falling oscillating temperature)
There is evidence in the literature that the isothermal degradation of certain nutrients can sometimes be better described by the Weibullian model  than by fixed-order kinetics [1, 2].
than by fixed-order kinetics [1, 2]. 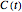 in this model is the time-dependent concentration ratio,
in this model is the time-dependent concentration ratio, 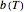 is a temperature-dependent rate constant and
is a temperature-dependent rate constant and 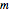 is the shape factor, assumed to be constant or a very weak function of temperature.
is the shape factor, assumed to be constant or a very weak function of temperature.
The temperature dependence of 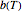 is assumed to follow the Arrhenius equation, which can be replaced by the simpler exponential model
is assumed to follow the Arrhenius equation, which can be replaced by the simpler exponential model 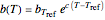 without sacrificing the fit [3]. In this model,
without sacrificing the fit [3]. In this model, 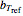 is the rate constant at an arbitrary reference temperature
is the rate constant at an arbitrary reference temperature 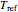 , both
, both 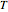 and
and 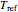 are in °C, and
are in °C, and  is a characteristic constant having units of
is a characteristic constant having units of 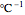 . Incorporating
. Incorporating 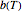 , thus defined, into the isothermal degradation equation leads to the general isothermal model
, thus defined, into the isothermal degradation equation leads to the general isothermal model 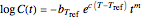 .
.
Assume that when the temperature varies, the degradation rate is the isothermal rate at temperature 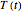 , at time
, at time 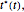 that corresponds to the concentration ratio
that corresponds to the concentration ratio 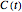 [2, 4]. Implementing this assumption renders the general dynamic degradation model in the form
[2, 4]. Implementing this assumption renders the general dynamic degradation model in the form 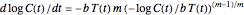 , with the boundary condition
, with the boundary condition 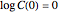 , from which
, from which 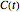 is determined.
is determined.
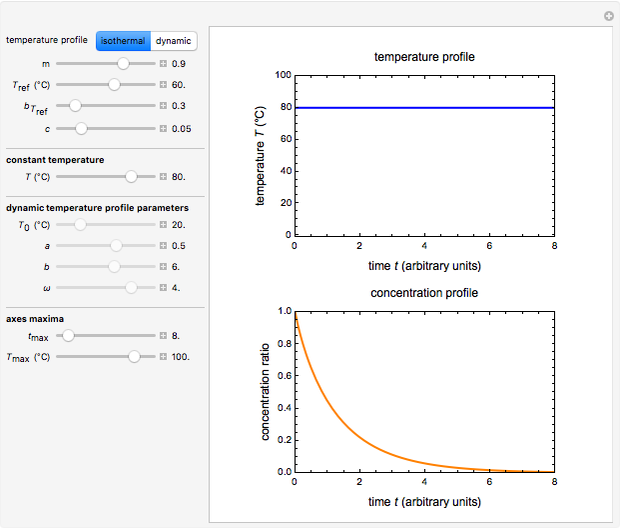
In this Demonstration you can choose between simulating isothermal degradation at chosen constant temperatures or, as examples, dynamic degradation at linearly rising and falling oscillating temperatures. You can vary the degradation kinetic parameters, namely, 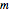 ,
, 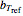 and
and  with sliders. You can also vary the constant temperature
with sliders. You can also vary the constant temperature 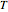 . For dynamic degradation, you can vary the temperature profile parameters
. For dynamic degradation, you can vary the temperature profile parameters 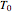 , the initial temperature
, the initial temperature  in °C, the slope of the temperature rise
in °C, the slope of the temperature rise 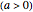 or fall
or fall 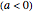 in
in  units, the temperature oscillation amplitude
units, the temperature oscillation amplitude  in °C, and the frequency
in °C, and the frequency  in units of
in units of  .
.
Not all combinations of the temperature profile parameters 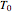 ,
,  ,
,  and
and  in the dynamic temperature profile equation and the kinetic parameters
in the dynamic temperature profile equation and the kinetic parameters 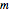 ,
, 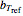 and
and  lead to realistic degradation curves.
lead to realistic degradation curves.
References
[1] M. G. Corradini and M. Peleg, "A Model of Non-isothermal Degradation of Nutrients, Pigments and Enzymes," Journal of the Science of Food and Agriculture, 84(3), 2004 pp. 217–226. doi:10.1002/jsfa.1647.
[2] M. G. Corradini and M. Peleg, "Prediction of Vitamins Loss During Non-isothermal Heat Processes and Storage with Non-linear Kinetic Models," Trends in Food Science and Technology, 17(1), 2006 pp. 24–34. doi:10.1016/j.tifs.2005.09.004.
[3] M. Peleg, M. D. Normand and M. G. Corradini, "The Arrhenius Equation Revisited," Critical Reviews in Food Science and Nutrition, 52(9), 2012 pp. 830–851. doi:10.1080/10408398.2012.667460.
[4] M. Peleg, A. D. Kim and M. D. Normand, "Predicting Anthocyanins' Isothermal and Non-isothermal Degradation with the Endpoints Method," Food Chemistry, 187(15), 2015 pp. 537–544. doi:10.1016/j.foodchem.2015.04.091.
Permanent Citation