Electromagnetic Fields For Hertzian Dipoles

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
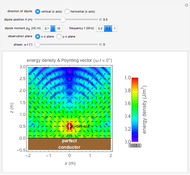
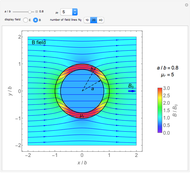
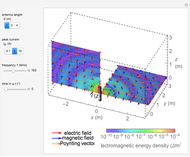
This Demonstration shows the electromagnetic fields for an electric dipole or a Hertzian dipole, the electric and magnetic fields, the associated energy densities, and the Poynting vector distributions. You can vary the dipole moment, frequency, and time for either a DC or static dipole field.
Contributed by: Y. Shibuya (September 2012)
Open content licensed under CC BY-NC-SA
Snapshots
Details
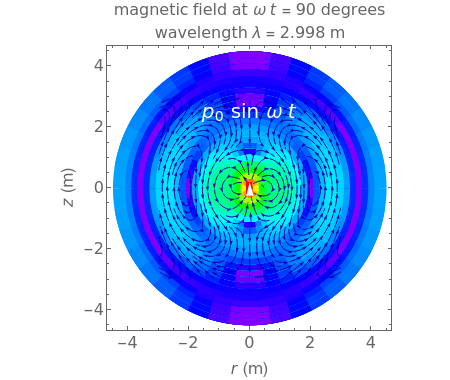
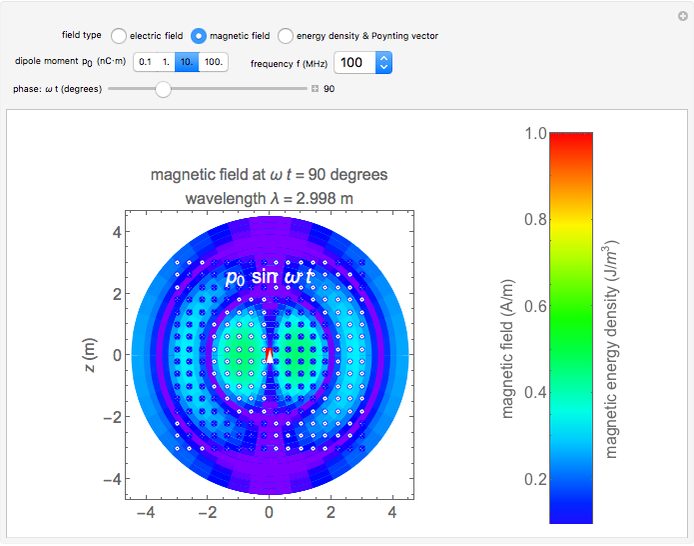
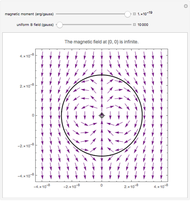
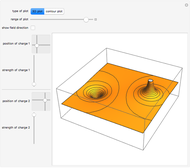
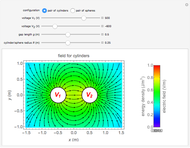
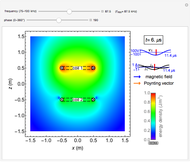
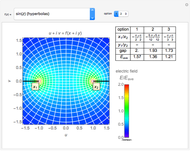
Snapshot 1: a magnetic field distribution
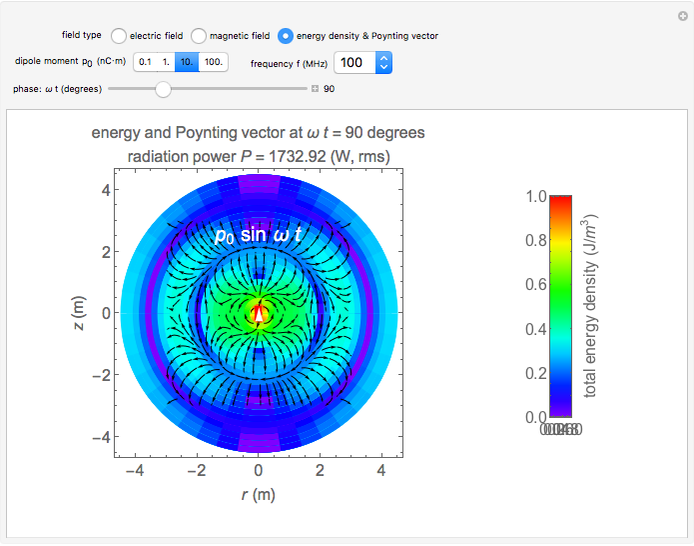
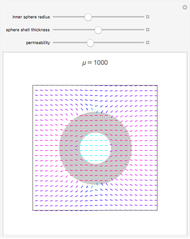
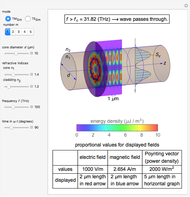
Snapshot 2: an energy density and Poynting vector distributions
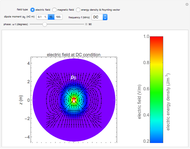
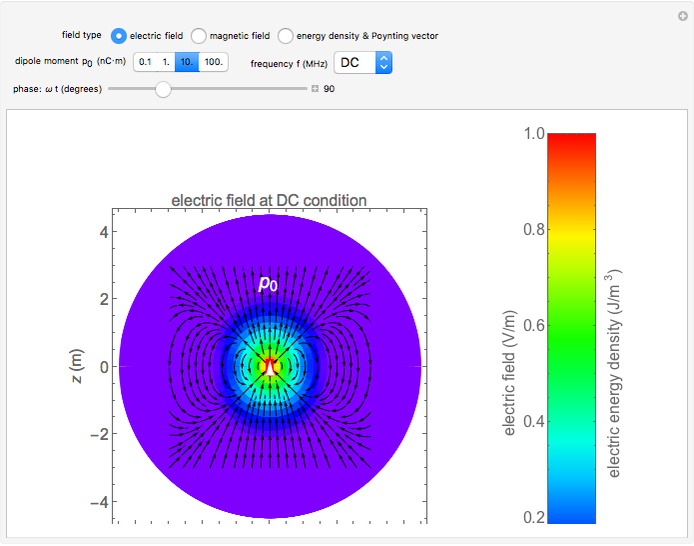
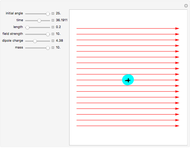
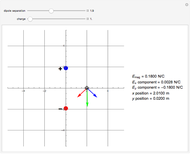
Snapshot 3: a DC electric field
The electromagnetic fields of a Hertzian dipole can be analyzed using the electrical Hertz vector:
 ,
,
where 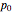 is the dipole moment (a vector in the
is the dipole moment (a vector in the  direction in this analysis), and
direction in this analysis), and  is the distance to the observation point. The energy density
is the distance to the observation point. The energy density  is
is
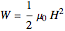 .
.
The electric and magnetic fields can be calculated as follows:
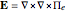 ,
,
 (in phasor,
(in phasor, 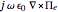 ,
,  ).
).
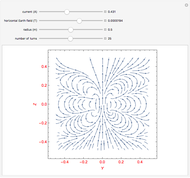
In the sinusoidally oscillating dipole of 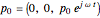 in cylindrical coordinates
in cylindrical coordinates  , the field vectors in the
, the field vectors in the  -
- plane are
plane are
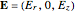 ,
,  .
.
The total energy density  and Poynting vector
and Poynting vector  are given by
are given by
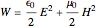 ,
, 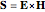 .
.
In the graphics, the field strengths are shown by color and the directions are shown by arrows.
The DC fields are obtained from the above equation, with the magnetic field equal to zero.
Reference
[1] J. A. Stratton, Electromagnetic Theory, New York: McGraw-Hill, 1941.
Permanent Citation