Enthalpy and Entropy Departure Functions for Gases

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
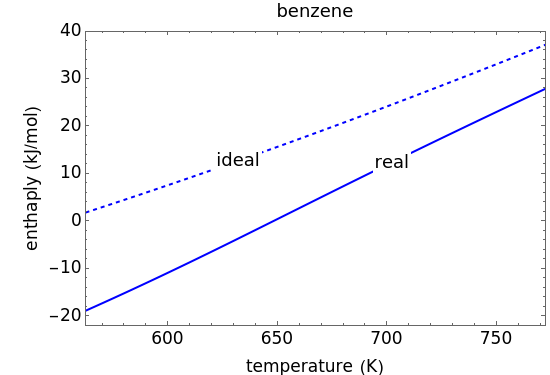
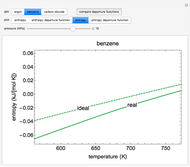
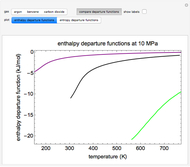
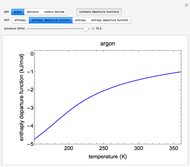
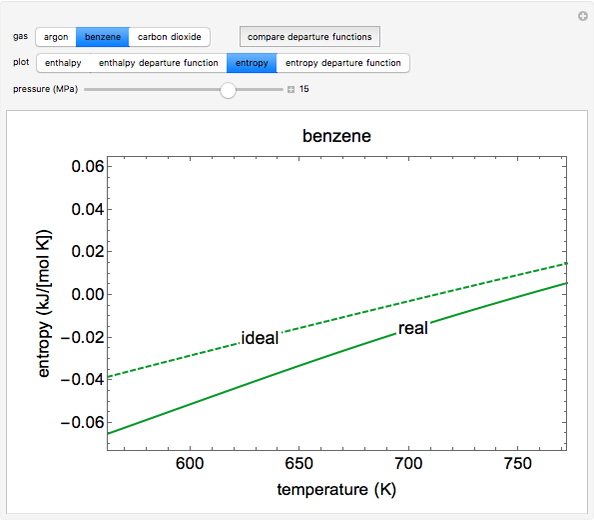
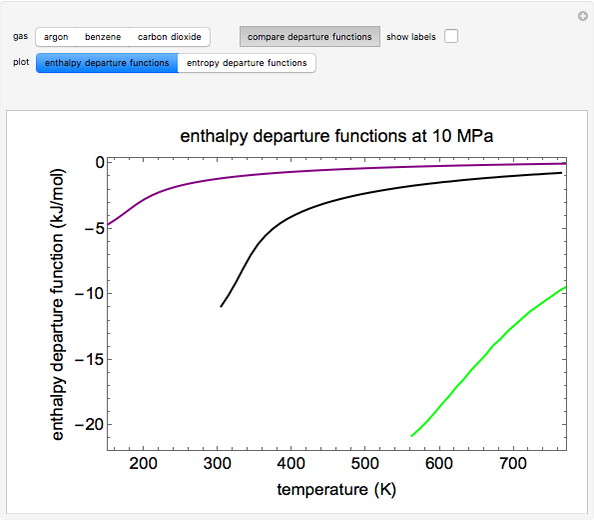
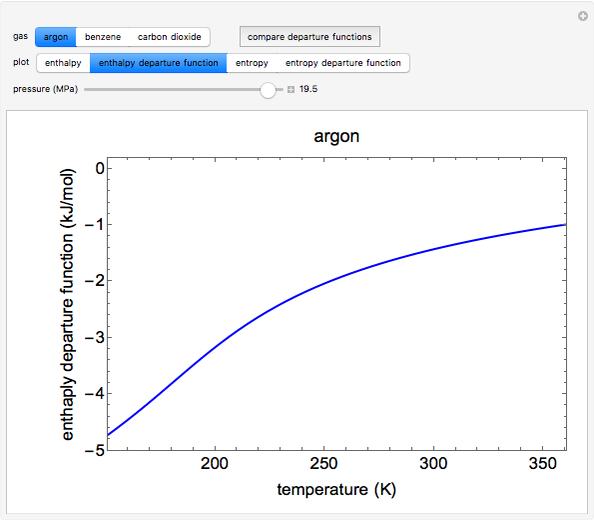
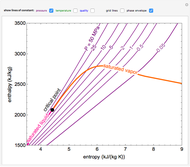
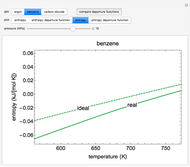
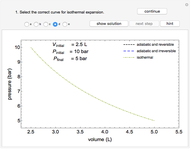
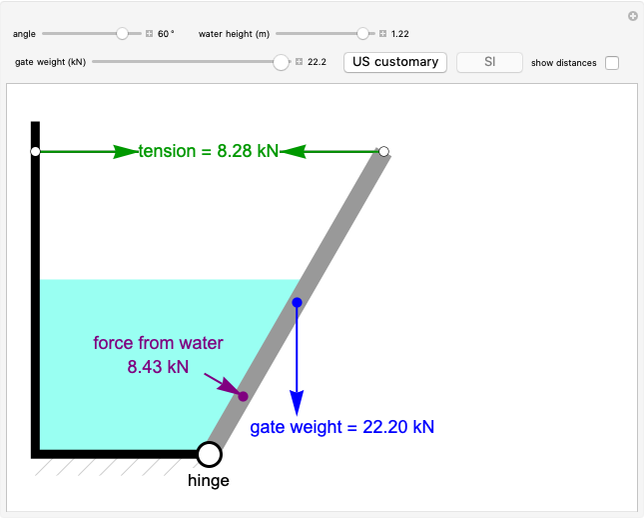
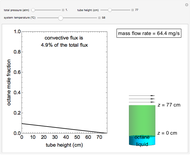
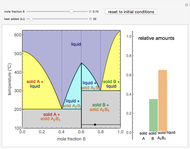
This Demonstration plots the enthalpies and entropies for a real gas and an ideal gas as a function of temperature, relative to a reference state at a selected pressure and temperature, using the Peng–Robinson equation of state and ideal-gas heat capacities. Select argon, benzene, or carbon dioxide with buttons. For each gas, the temperature scale is adjusted so the plots are only shown above the critical temperature. Select enthalpy or entropy departure function, which is the difference between the thermodynamic property (enthalpy, entropy) for a real gas and an ideal gas at the same temperature and pressure. You can vary the pressure with the slider. Select "compare departure functions" to view departure functions for all three gases as a function of temperature at 10 MPa. Click "show labels" to label each departure function curve with the corresponding gas.
Contributed by: Rachael L. Baumann and Neil Hendren (May 2015)
Additional contributions by: John L. Falconer, Derek M. Machalek, Nathan S. Nelson, and Garrison J. Vigil
(University of Colorado Boulder, Department of Chemical and Biological Engineering)
Open content licensed under CC BY-NC-SA
Details
Enthalpy 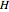 and entropy
and entropy 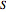 are calculated using the Peng–Robinson equation of state (EOS) for a real gas and the ideal gas law for an ideal gas:
are calculated using the Peng–Robinson equation of state (EOS) for a real gas and the ideal gas law for an ideal gas:
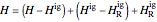 ,
,
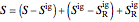 ,
,
where 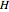 is in kJ/mol and
is in kJ/mol and 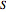 is in kJ/[mol K]; the superscript
is in kJ/[mol K]; the superscript 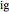 represents an ideal gas, the subscript
represents an ideal gas, the subscript 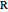 refers to the reference state, and
refers to the reference state, and 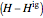 and
and 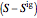 are the enthalpy and entropy departure functions for a real gas calculated from the Peng–Robinson EOS, while
are the enthalpy and entropy departure functions for a real gas calculated from the Peng–Robinson EOS, while 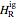 and
and 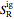 are the ideal gas enthalpy and entropy at the reference state.
are the ideal gas enthalpy and entropy at the reference state.
 ,
,
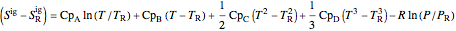 ,
,
where 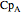 ,
, 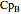 ,
, 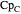 , and
, and 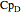 are heat capacity constants (
are heat capacity constants (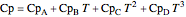 ),
), 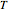 is temperature (K), and
is temperature (K), and 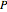 is pressure (MPa).
is pressure (MPa).
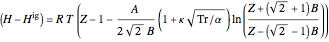 ,
,
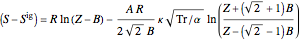 ,
,
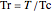 ,
,
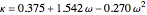 ,
,
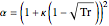 ,
,
where 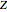 is the compressibility factor,
is the compressibility factor, 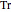 is the reduced temperature (dimensionless, not to be confused with
is the reduced temperature (dimensionless, not to be confused with 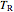 ),
), 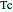 is the critical temperature (K),
is the critical temperature (K),  is the acentric factor, and
is the acentric factor, and  and
and  are constants.
are constants.
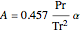 ,
,
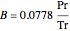 ,
,
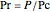 ,
,
where 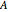 and
and 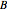 are constants,
are constants, 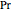 is the reduced pressure (dimensionless, not to be confused with
is the reduced pressure (dimensionless, not to be confused with 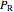 ), and
), and  is the critical pressure (MPa).
is the critical pressure (MPa).
These equations are used to calculate the compressibility factor 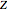 :
:
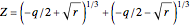 ,
,
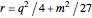 ,
,
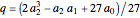 ,
,
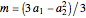 ,
,
where  ,
,  ,
, 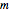 ,
, 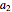 ,
, 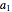 , and
, and 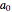 are constants used for simplification.
are constants used for simplification.
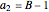 ,
,
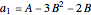 ,
,
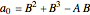 .
.
The screencast video at [1] explains how to use this Demonstration.
Reference
[1] Enthalpy and Entropy Departure Functions for Gases. www.colorado.edu/learncheme/thermodynamics/EntropyEnthalpyDepartureFunctions.html.
Snapshots
Permanent Citation