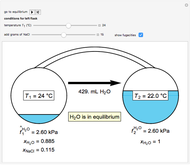
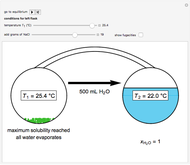
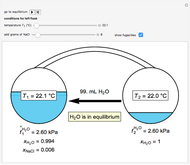
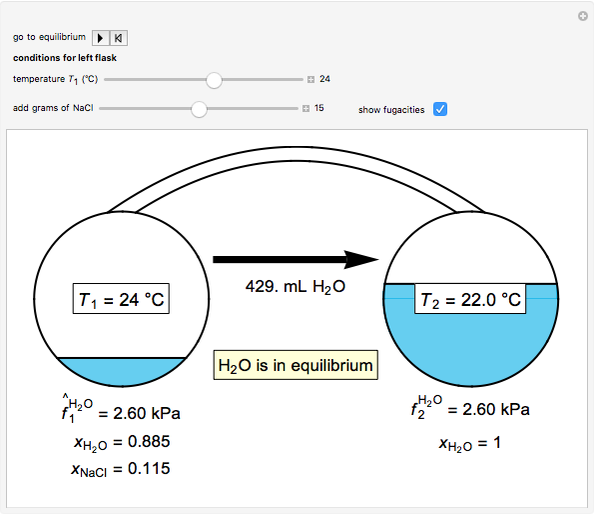
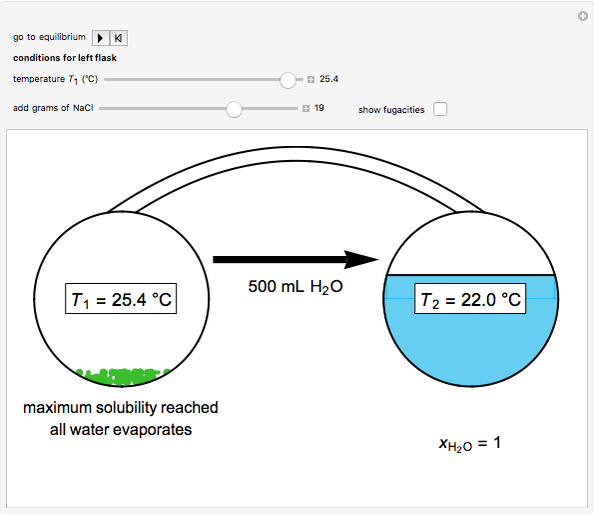
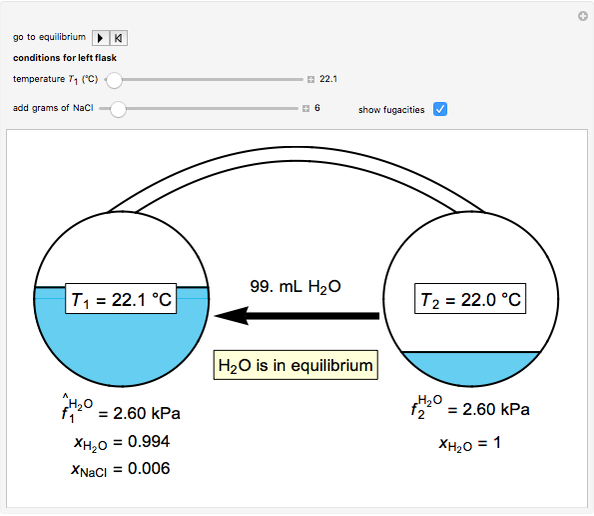
Two 1-liter flasks are at different temperatures; initially the left flask contains 500 mL of water with dissolved salt and the right flask contains 200 mL of pure water. You can control the temperature of the left flask and the amount of salt dissolved with sliders. Click the play button "go to equilibrium" to remove the caps on each flask. Water transfers from one flask to the other to try to make water fugacities equal in each flask. For some conditions, all the water transfers to the left or right flask. Check "show fugacities" to display the water fugacities in each flask.