Illustrating the Central Limit Theorem with Sums of Bernoulli Random Variables

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
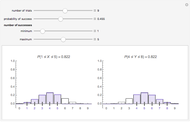
Consider the central limit theorem for independent Bernoulli random variables  , where
, where  and
and  ,
,  . Then the sum
. Then the sum 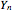 is binomial with parameters
is binomial with parameters  and
and  and
and  converges in distribution to the standard normal. The exact distribution for
converges in distribution to the standard normal. The exact distribution for  may be written
may be written  , where
, where  ,
, 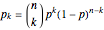 ,
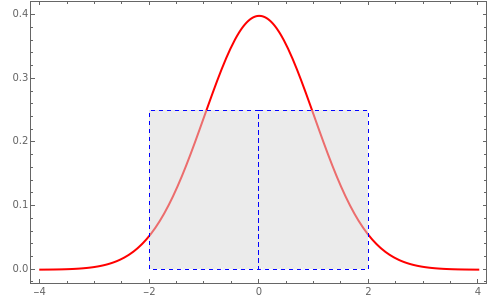
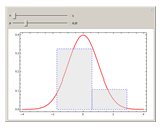
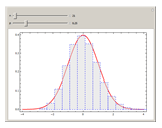
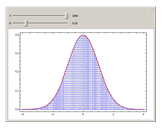
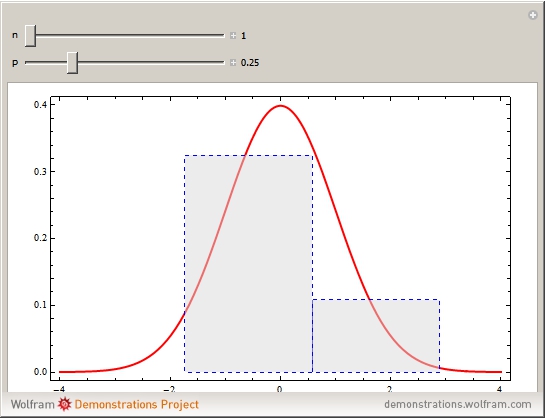
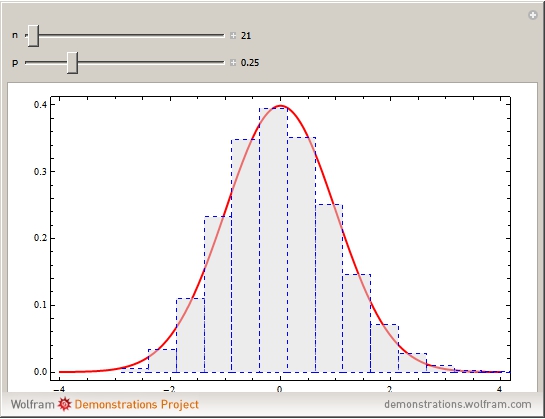
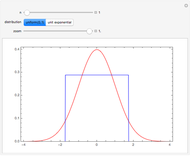
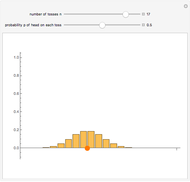
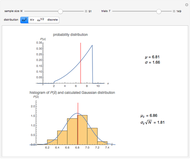
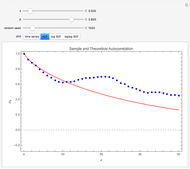
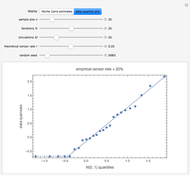
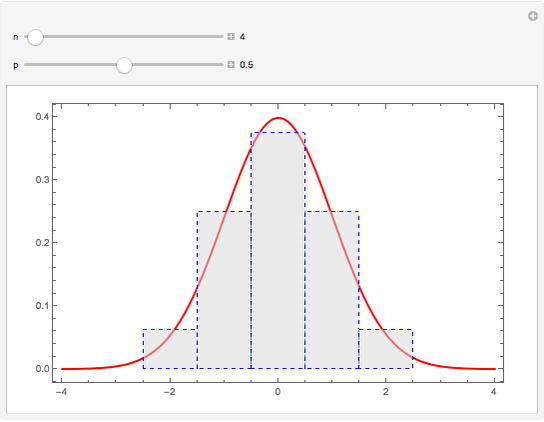
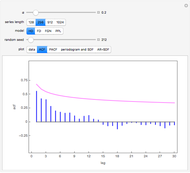
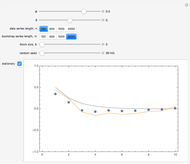
, 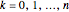 . The convergence may be illustrated using rectangles of width
. The convergence may be illustrated using rectangles of width  and height
and height  centered at
centered at  . As
. As  increases, the rectangles closely approach the standard normal density function. The convergence is faster in the symmetric case when
increases, the rectangles closely approach the standard normal density function. The convergence is faster in the symmetric case when 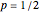 .
.
Contributed by: Ian McLeod (University of Western Ontario) (February 2010)
Open content licensed under CC BY-NC-SA