Simulating Microbial Count Records with an Expanded Fermi Solution Model

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
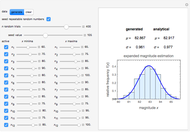
This Demonstration generates simulated microbial count records with and without zero entries. It is based on the assumption that each count is determined by the combination of several factors, specified by their upper and lower limits, which promote or suppress the population size. Where no zero entries are allowed, the counts' distribution is approximately lognormal. Where the organism, such as a pathogen, is not always present, the probability of its presence is also included as a factor. This Demonstration displays the generated counts and their histogram and calculates the risk of encountering a count exceeding a specified level considered hazardous.
Contributed by: Mark D. Normand and Micha Peleg (June 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
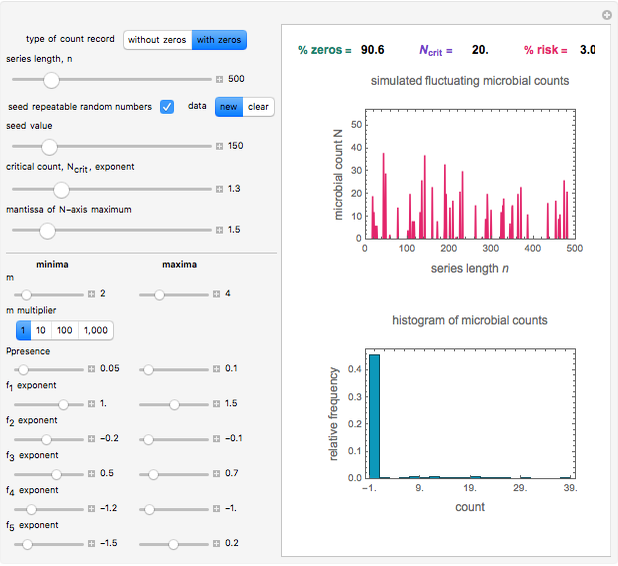
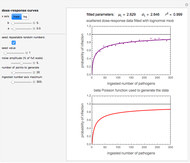
Snapshot 1: simulated record of the total microbial count in a nutrient-rich raw food
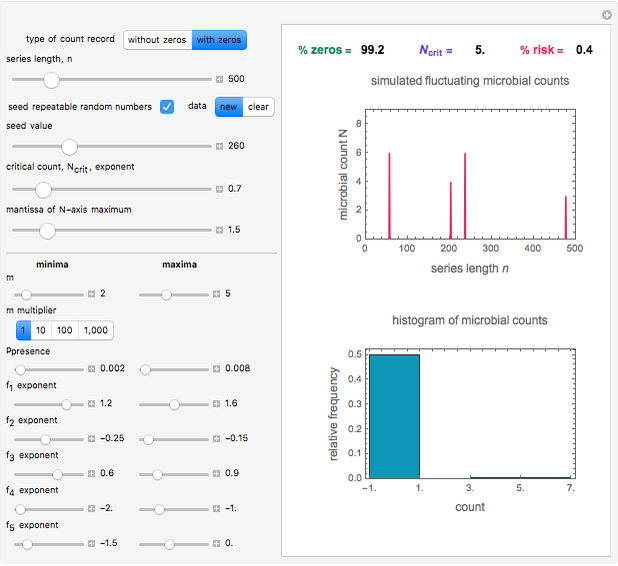
Snapshot 2: simulated record of a common pathogen in a nutrient-rich raw food
Snapshot 3: simulated record of a rare virulent pathogen in fresh produce
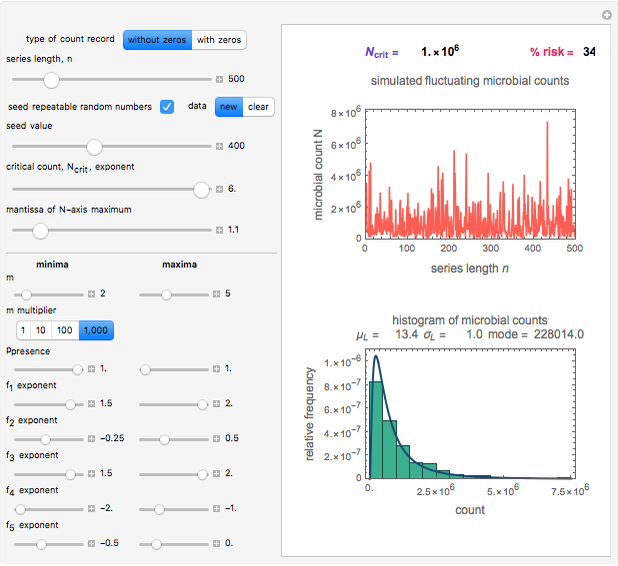
This Demonstration uses the expanded Fermi solution to simulate microbial count records of the kind encountered in quality assurance of raw and processed foods. The record consists of a series of  count values,
count values, 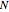 . The generated records are of two kinds; without and with zero entries, which can be selected with a setter. The record length,
. The generated records are of two kinds; without and with zero entries, which can be selected with a setter. The record length,  , is entered with a slider. The upper and lower limits of the initial number of microbes present,
, is entered with a slider. The upper and lower limits of the initial number of microbes present, 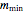 and
and 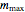 , are also specified with sliders. It is assumed that each individual count is the result of the interplay of up to five random factors,
, are also specified with sliders. It is assumed that each individual count is the result of the interplay of up to five random factors, 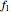 through
through 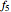 , whose effect is multiplicative. These factors are chosen by the user and their upper and lower limits,
, whose effect is multiplicative. These factors are chosen by the user and their upper and lower limits, 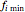 and
and 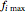 , are all entered with sliders. Because these factors can vary dramatically, and they can be growth promoters or suppressors, the scales of the
, are all entered with sliders. Because these factors can vary dramatically, and they can be growth promoters or suppressors, the scales of the 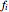 sliders are logarithmic (base 10). Thus, a negative setting produces a fraction of
sliders are logarithmic (base 10). Thus, a negative setting produces a fraction of 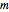 and a positive setting produces a multiplier of
and a positive setting produces a multiplier of 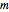 . In the case of records allowing zero entries, as in the sporadic appearance of pathogens, the upper and lower limits of their presence probability,
. In the case of records allowing zero entries, as in the sporadic appearance of pathogens, the upper and lower limits of their presence probability, 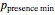 and
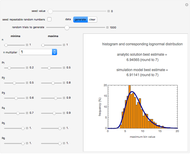
and 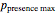 , are also entered as factors. The program then calculates the product of all the relevant factors, each assigned a random value within its respective range. In the absence of zero entries, counts calculated by this procedure are expected to have an approximately lognormal distribution whose logarithmic mean,
, are also entered as factors. The program then calculates the product of all the relevant factors, each assigned a random value within its respective range. In the absence of zero entries, counts calculated by this procedure are expected to have an approximately lognormal distribution whose logarithmic mean, 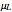 , standard deviation,
, standard deviation, 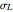 , and mode are calculated and displayed.
, and mode are calculated and displayed.
The program plots the generated record, 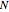 versus
versus  , and below it, its histogram. The display also includes the percent risk of a count exceeding a level,
, and below it, its histogram. The display also includes the percent risk of a count exceeding a level, 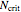 , considered hazardous, entered by the user with a slider. Because
, considered hazardous, entered by the user with a slider. Because 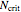 can vary over several orders of magnitude, its slider's scale is logarithmic (base 10). Where the record includes zero entries, their percentage is displayed above the plot. In the case of a record containing no zeros, the lognormal distribution curve having the calculated
can vary over several orders of magnitude, its slider's scale is logarithmic (base 10). Where the record includes zero entries, their percentage is displayed above the plot. In the case of a record containing no zeros, the lognormal distribution curve having the calculated 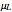 and
and 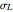 is superimposed on the histogram.
is superimposed on the histogram.
The Demonstration lets you generate repeatable random records from a fixed seed by clicking a checkbox and entering the seed value with a slider. It also allows generation of a new random count record without changing any other settings by clicking the data "clear" and then "new" setters. The overall size of the generated count record can be altered by the "m multiplier" setter bar and the upper limit of the microbial count 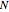 -axis plot scale by a slider.
-axis plot scale by a slider.
This Demonstration was constructed to cover a large range of scenarios. However, not all records generated by the program correspond to a real microbial system.
Although this Demonstration has been primarily intended for microbial records, it can also be used for other randomly fluctuating time series of the kind encountered in quality assurance.
References
[1] M. Peleg, Advanced Quantitative Microbiology for Food and Biosystems: Models for Predicting Growth and Inactivation, Boca Raton, FL: CRC Press, 2006.
[2] M. Peleg, M. D. Normand, J. Horowitz, and M. G. Corradini, "An Expanded Fermi Solution for Microbial Risk Assessment," International Journal of Food Microbiology, 113(1), 2007 pp. 92–101.
[3] M. Peleg, "Estimating the Probability of High Microbial Counts Using Excel." (Feb 4, 2008) http://www-unix.oit.umass.edu/~aew2000/MicCountProb/microbecounts.html.
Permanent Citation