Attenuation of a Sinusoidal Magnetic Field in a Halfspace Conductor

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
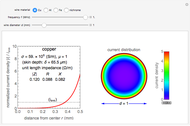
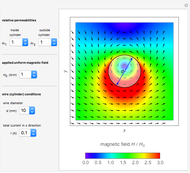
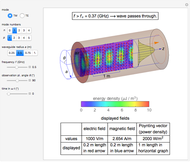
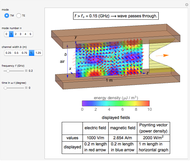
When a block of conductor is subjected to a changing magnetic field, a current is induced inside the conductor. This Demonstration shows this phenomenon when a sinusoidal magnetic field is applied to a halfspace conductor. The magnetic field of moderate frequency (and therefore, regarded as a near field) is assumed to be parallel to the conductor surface. The skin depth is determined by the frequency  as well as the conductor parameters of conductivity
as well as the conductor parameters of conductivity  and relative permeance
and relative permeance  . Taking the direction of the magnetic field as the
. Taking the direction of the magnetic field as the  -axis, the current is directed along the
-axis, the current is directed along the  -axis. The spatial distributions of
-axis. The spatial distributions of  and
and  (to which the electric field is proportional:
(to which the electric field is proportional:  ) are shown graphically.
) are shown graphically.
Contributed by: Y. Shibuya (November 2012)
Open content licensed under CC BY-NC-SA
Snapshots
Details
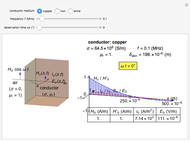
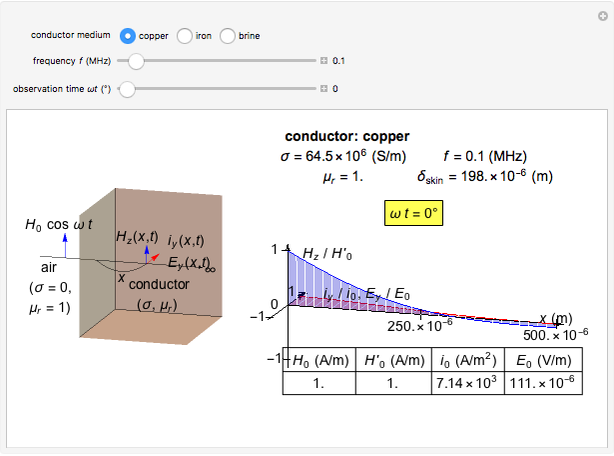
Snapshot 1: the skin depth of copper is about 200 μm in a 0.1 MHz field
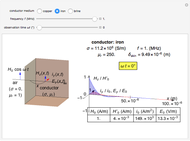
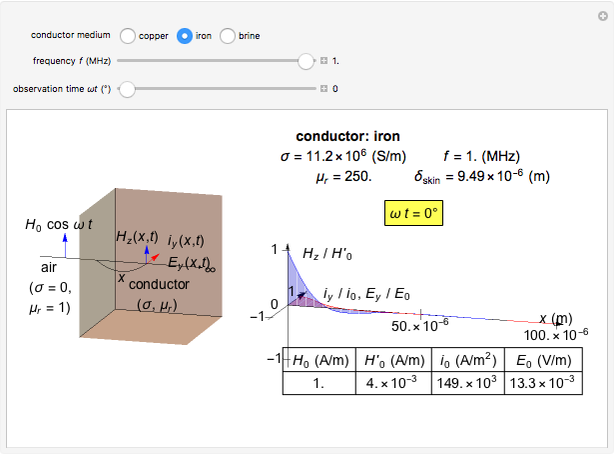
Snapshot 2: the high permeance of iron contributes to decreasing the diffusing distance
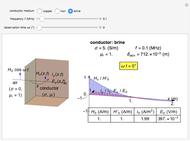
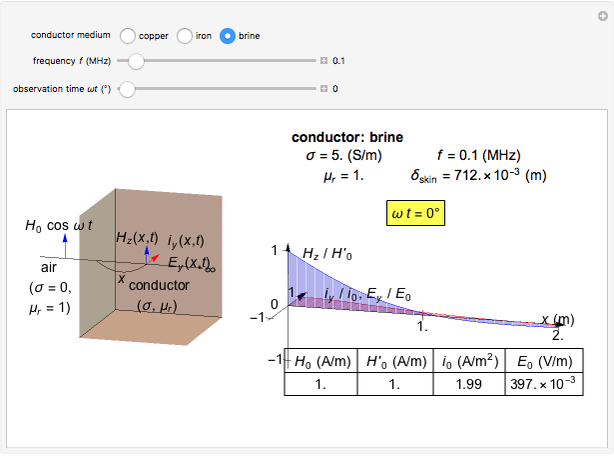
Snapshot 3: brine, a poor conductor, still retains screening effects to lengths of the order of meters
The electromagnetic fields in a conductor are governed by the diffusion equation, in which the skin depth is given by  . The values for copper and iron are much smaller than for brine. The fields, a function of space and time, are expressed as
. The values for copper and iron are much smaller than for brine. The fields, a function of space and time, are expressed as
 ,
,
 ,
,
 .
.
The maximum values are related by  ,
,  ,
,  , where the amplitude of the applied field is set equal to
, where the amplitude of the applied field is set equal to  . Those values are shown in the table at the bottom. For copper and brine, the maximum magnetic field is equal to the applied field (
. Those values are shown in the table at the bottom. For copper and brine, the maximum magnetic field is equal to the applied field ( ); however, the interior field for iron becomes much smaller due to the large permeance.
); however, the interior field for iron becomes much smaller due to the large permeance.
As seen from the equations, the field distribution is quite analogous in all cases. The skin depth is the only parameter to define. The distribution curve may suggest a wave propagation into the interior. However, this phenomenon is not the electromagnetic wave defined by Maxwell's equations, but rather the result of diffusion of the magnetic field, which occurs at a variable speed.
Reference
[1] G. Lehner, Electromagnetic Field Theory for Engineers and Physicists (M. Horrer, trans.), New York: Springer, 2009.
Permanent Citation