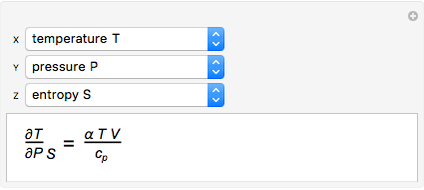
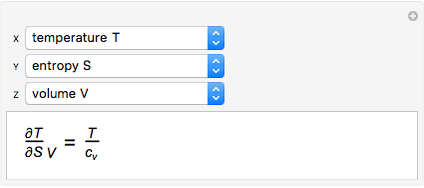
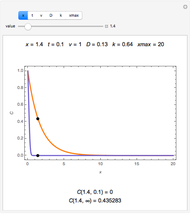
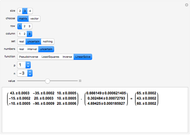
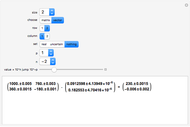
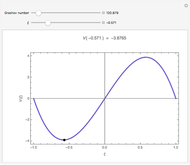
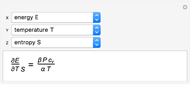
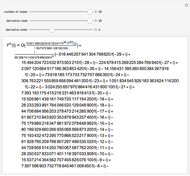
Derivation of Thermodynamic Derivatives Using Jacobians

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
Rules from the book by M. Tribus are explored to deduce specified thermodynamic derivatives of the thermodynamical variables (energy  , enthalpy
, enthalpy  , free energy of Helmholtz
, free energy of Helmholtz  , free energy of Gibbs
, free energy of Gibbs  , entropy
, entropy  , temperature
, temperature  , pressure
, pressure  , and volume
, and volume 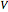 ) as functions of measurable quantities (temperature
) as functions of measurable quantities (temperature  , pressure
, pressure  , volume
, volume 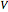 , coefficient of thermal expansion
, coefficient of thermal expansion  , coefficient of compressibility
, coefficient of compressibility  , heat capacity at constant pressure
, heat capacity at constant pressure  , and heat capacity at constant volume
, and heat capacity at constant volume 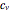 ). Sometimes the entropy
). Sometimes the entropy  will remain outside the derivatives.
will remain outside the derivatives.
Contributed by: Mikhail Dimitrov Mikhailov (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
The main thermodynamical variables are energy  , enthalpy
, enthalpy  , free energy of Helmholtz
, free energy of Helmholtz  , free energy of Gibbs
, free energy of Gibbs  , entropy
, entropy  , temperature
, temperature  , pressure
, pressure  , and volume
, and volume 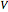 .
.
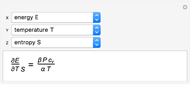
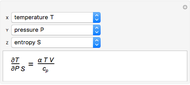
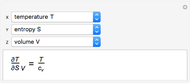
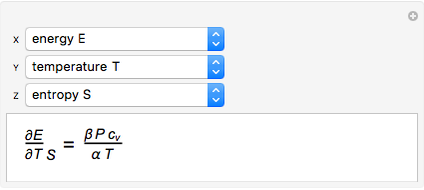
It is possible to form 336 partial derivatives of the type 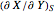 , where
, where  ,
,  , and
, and 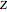 are among these eight variables.
are among these eight variables.
It is possible to express one derivative in terms of three outer derivatives. Among the 336 derivatives there are approximately 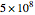 combinations, but only a small fraction of them have practical value.
combinations, but only a small fraction of them have practical value.
The Jacobian notation shows thermodynamic derivatives in an elegant manner. The application of Jacobians in thermodynamics appears to have started with Bryan [1].
A collection of Jacobian expressions is presented in [2] and [3].
Based on a suggestion made by E. Jaynes, Tribus developed rules for the deduction of thermodynamic partial derivatives [4]. For efficiency a new rule is added.
[1] G. H. Bryan, (article title unknown), Encyklopedie der mathematishen Wissenschaften, Bd V, Teil 1, A. Sommerfeld, ed., Leipzig: G. B. Teubner, 1903 p. 113.
[2] P. W. Bridgman, A Condensed Collection of Thermodynamic Formulas, Cambridge: Harvard University Press, 1925.
[3] A. N. Shaw, "The Derivation of Thermodynamical Relations for a Simple System," Phil. Trans. Roy. Soc. A, 234(740), 1935 pp. 299–328.
[4] M. Tribus, Thermostatics and Thermodynamics, Princton, NJ: Van Nostrand, 1961.
Permanent Citation