Wolfram Demonstrations Project

13,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

Exploring Skewness in Box Plots

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

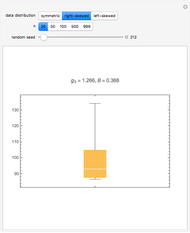
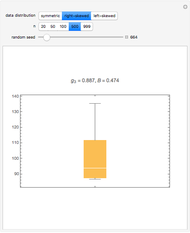
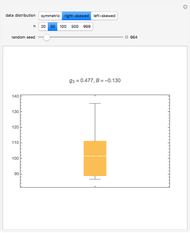
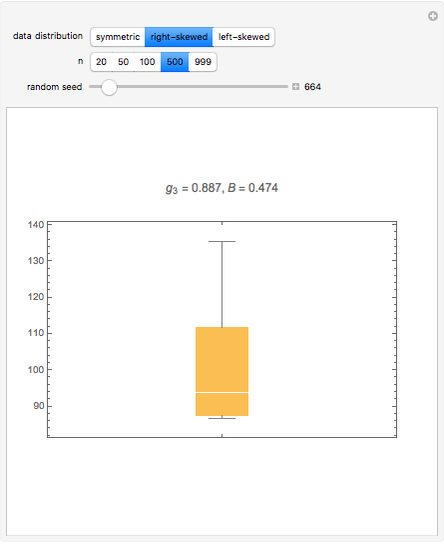
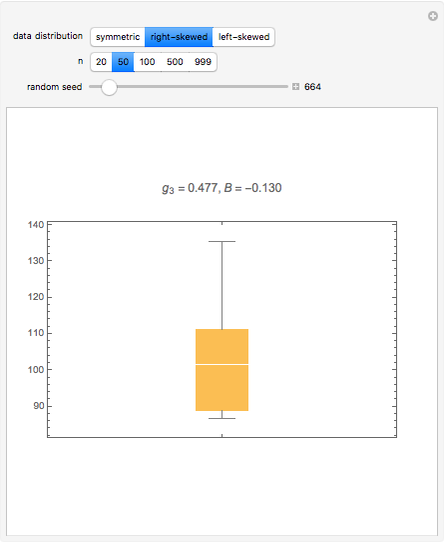
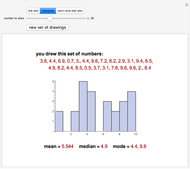
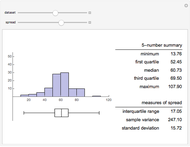
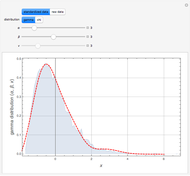
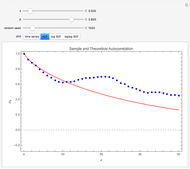
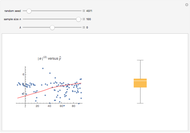
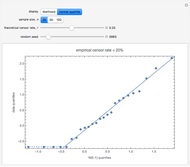
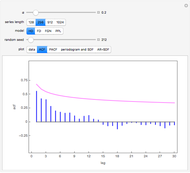
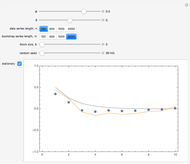
The box-and-whisker plot, also known simply as the box plot, is useful in visualizing skewness or lack thereof in data. The usual form of the box plot, shown in the graphic, shows the 25% and 75% quartiles, and , at the bottom and top of the box, respectively. The median, , is shown by the horizontal line drawn through the box. The whiskers extend out to the extremes. For brevity, the whiskers at the top and bottom are referred to as the positive and negative whiskers.

[more]

In judging skewness, positive skewness (or right-skewed) distributions are often indicated by , which is usually apparent from inspection of the box plot. This condition is equivalent to , where is the quartile skewness coefficient. This Demonstration shows that using , , and in this way is not a reliable way to judge skewness when the sample size is not large, as in or . Skewness, denoted by , is more reliably indicated visually by the relative size of the whiskers. A large positive whisker relative to the negative whisker is a better indication of positive skewness.

Similarly, the whiskers may be used for judging symmetry and negative skewness.

[less]

Contributed by: Ian McLeod (March 2011)
Open content licensed under CC BY-NC-SA

Snapshots

Details

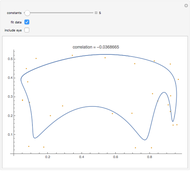
A beta distribution with parameters (0.3, 0.8) is used for the case of positive skewness and (0.8, 0.3) in the negative case. The skewness coefficient is ±0.95. All data is scaled so the distribution mean and standard deviation are 100 and 15, respectively. In the symmetric case, the normal distribution is used.

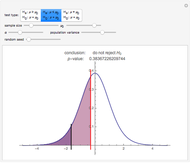
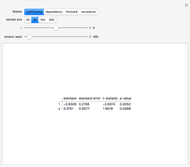
In the present simulations, the quartile skewness is not as reliable as the usual skewness coefficient. This is to be expected when the third moment exists. With financial data, infinite variance distributions are sometimes used [1] and in this case the quartile skewness may be expected to provide a more reliable estimate.

[1] J.-W. Lin and A. I. McLeod, "Portmanteau Tests for ARMA Models with Infinite Variance," Journal of Time Series Analysis, 29(3), 2008 pp. 600–617.

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send