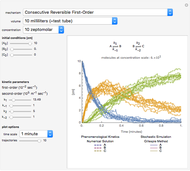
Eyring-Polanyi versus Exponential Model for Chemical Reactions

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
The temperature dependence of the rate of chemical reactions and biological processes is frequently described by the Arrhenius equation. A recently introduced alternative in food research is the Eyring–Polanyi model, adapted from chemical kinetics. It is shown here that there are many conditions in which data generated with a simpler exponential model can be fitted with the Eyring–Polanyi model and vice versa. This suggests that an Eyring–Polanyi model is not sufficient to definitively establish the existence of a temperature-independent "free energy of activation" in food processes and reactions unless the presence of an activated state can be confirmed independently.
Contributed by: Mark D. Normand, Christina S. Barsa, and Micha Peleg (February 2013)
Open content licensed under CC BY-NC-SA
Snapshots
Details
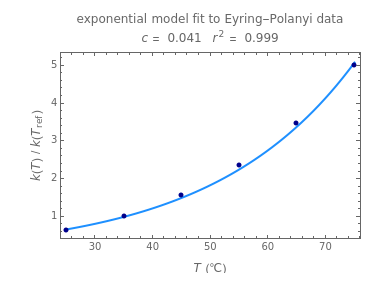
Snapshot 1: data generated with an Eyring–Polanyi-like equation where the absolute temperature in °K is replaced by  in °C and then fitted with the exponential model
in °C and then fitted with the exponential model
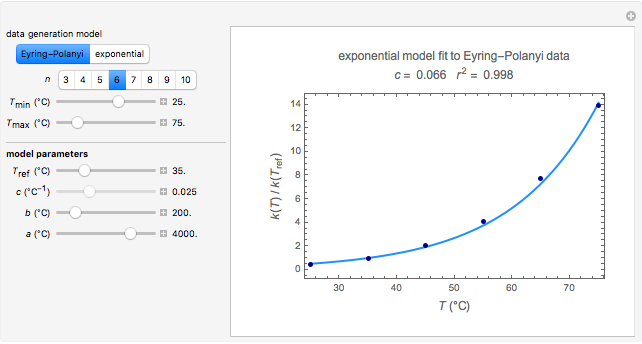
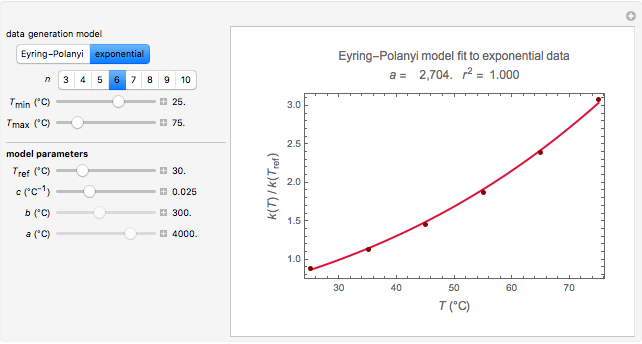
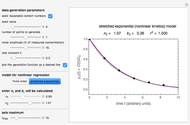
Snapshot 2: data generated with the exponential model and fitted with the Eyring–Polanyi model
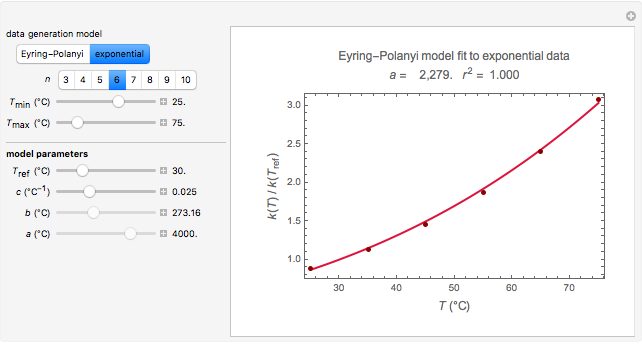
Snapshot 3: data generated with the exponential model and fitted with an Eyring–Polanyi-like equation where the absolute temperature in °K is replaced by  in °C and then fitted with the exponential model
in °C and then fitted with the exponential model
This Demonstration computes 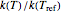 versus
versus 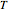 datasets using the Eyring–Polanyi or related equations that are fitted with an exponential model. It also generates such datasets using the exponential model that are fitted by the Eyring–Polanyi model or a related equation.
datasets using the Eyring–Polanyi or related equations that are fitted with an exponential model. It also generates such datasets using the exponential model that are fitted by the Eyring–Polanyi model or a related equation.
The Eyring–Polanyi-like equation is presented as 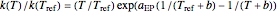 , where
, where 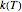 and
and 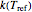 are the rates at temperature
are the rates at temperature 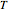 and the reference temperature,
and the reference temperature, 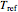 , respectively, both in °C, and
, respectively, both in °C, and  and
and  are constants having temperature dimension. Notice that setting
are constants having temperature dimension. Notice that setting  to 273.16 °C produces the standard Eyring–Polanyi equation. The exponential model equation is
to 273.16 °C produces the standard Eyring–Polanyi equation. The exponential model equation is 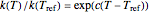 , where
, where  is a constant having temperature reciprocal units
is a constant having temperature reciprocal units 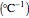 .
.
Choose the data generation model by clicking the setter bar. The data so generated will be fitted by the other model. Use the sliders to enter the number of points  , the plot's temperature range
, the plot's temperature range 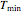 and
and 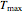 , the reference temperature
, the reference temperature 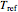 , and the generation or fit parameters
, and the generation or fit parameters  ,
,  , and
, and  . The display shows either the
. The display shows either the 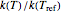 versus
versus 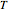 data generated with the original or modified Eyring–Polanyi model and their fit by the exponential model, or the
data generated with the original or modified Eyring–Polanyi model and their fit by the exponential model, or the 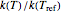 versus
versus 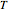 data generated with the exponential model and their fit by the original or modified Eyring–Polanyi model.
data generated with the exponential model and their fit by the original or modified Eyring–Polanyi model.
When the parameter  is large, the modified Eyring–Polanyi plot of the data generated by the exponential model is nearly linear.
is large, the modified Eyring–Polanyi plot of the data generated by the exponential model is nearly linear.
References
[1] M. Peleg, M. D. Normand, and M. G. Corradini, "The Arrhenius Equation Revisited," Critical Reviews in Foods Science and Nutrition, 52(9), 2012 pp. 830–851. doi:10.1080/10408398.2012.667460.
[2] C. S. Barsa, M. D. Normand, and M. Peleg, "On Models of the Temperature Effect on the Rate of Chemical Reactions and Biological Processes in Foods," Food Engineering Reviews, 4(4), 2012 pp. 191–202. doi:10.1007/s12393-012-9056-x.
Permanent Citation