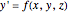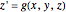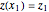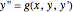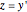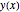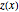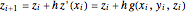Graphic Solution of a Second-Order Differential Equation

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
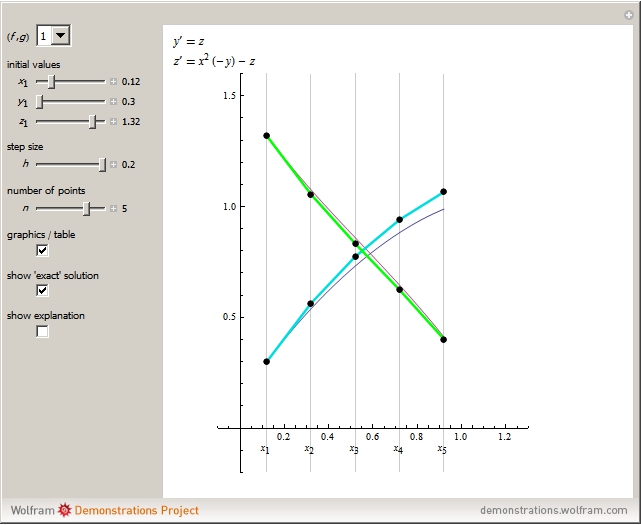
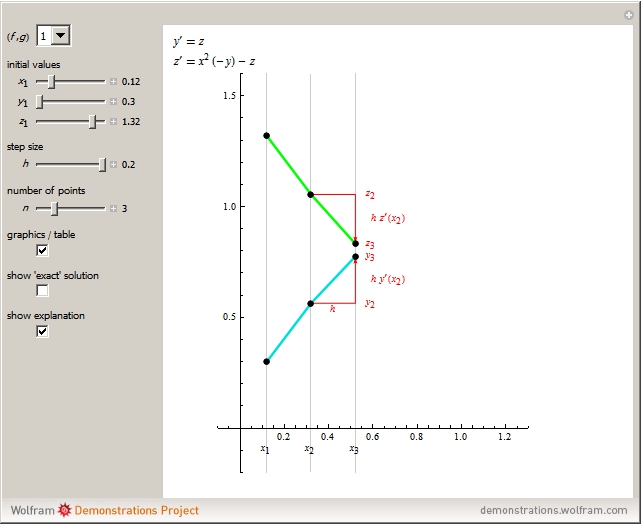
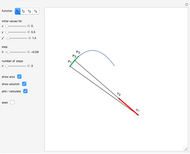
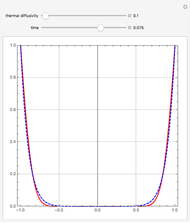
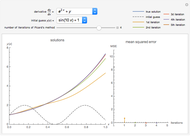
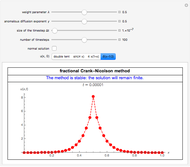
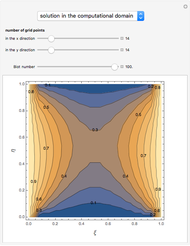
This Demonstration shows the Euler–Cauchy method for approximating the solution of an initial value problem with a second-order differential equation. An example of such an equation is 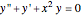 , with derivatives from now on always taken with respect to
, with derivatives from now on always taken with respect to  . This equation can be written as a pair of first-order equations,
. This equation can be written as a pair of first-order equations,  ,
,  .
.
Contributed by: Izidor Hafner (January 2014)
Open content licensed under CC BY-NC-SA
Snapshots
Details
References
[1] V. I. Smirnoff, Lectures in Higher Mathematics (in Russian), vol. 2, Moscow: Nauka, 1967 p. 50.
[2] L. Euler, "De Integratione Aequationum Differentialium Per Approximationem," Institutionum Calculi Integralis Volumen Primum, 1768. www.math.dartmouth.edu/~euler/docs/originals/E342sec2ch7.pdf.
Permanent Citation