Hedging the Black-Scholes Call Option

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
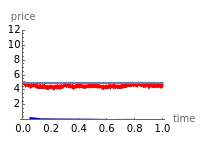
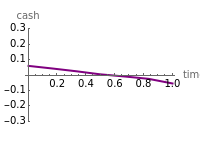
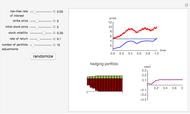
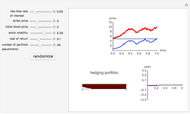
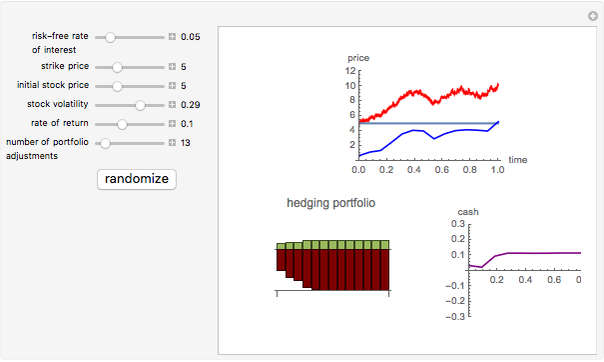
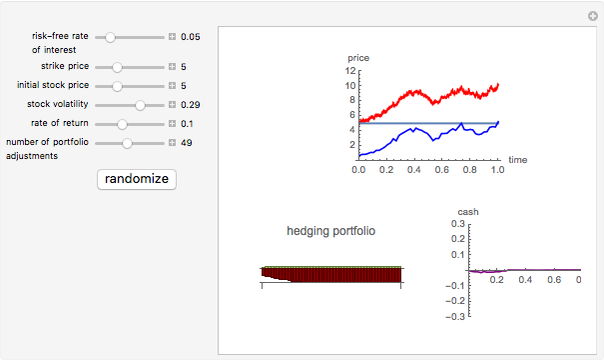
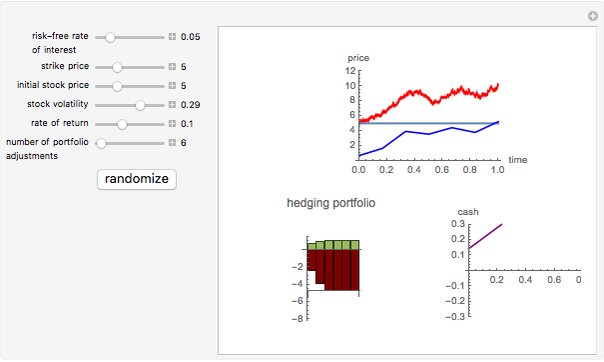
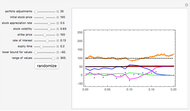
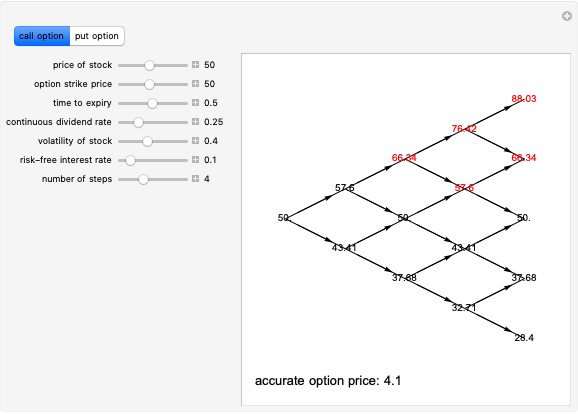
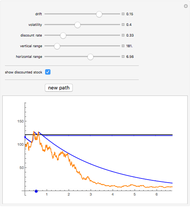
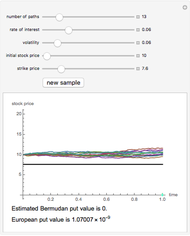
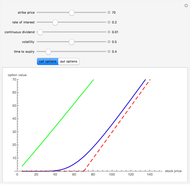
We demonstrate hedging of a vanilla European call option on stock paying no dividend in the Black–Scholes model. The top picture shows the path of an exponential Brownian motion (actually, a random walk approximation with a very small time step) representing the price of stock (colored red), the strike price of a European call option (colored dark blue), and the values of a hedging portfolio. The hedging portfolio itself, consisting of some stock (colored green) and a short position in a riskless bond (colored red) is shown below. The graph at the lower right shows the "cash flow" from the transactions performed in holding the portfolio. For a perfectly self-financing portfolio this graph ought to be perfectly flat and coincide with the horizontal axis.
[more]
Contributed by: Andrzej Kozlowski (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Hedging is both a theoretical device that makes it possible to calculate the "fair value" of derivative instruments such as options and a practical technique of risk management. Here we illustrate it on one of the simplest examples: a so-called vanilla European call option on stock whose price is assumed to follow an exponential Brownian motion (the Black–Scholes model). We assume the stock pays no dividend and that the rate of interest is constant (neither of these are essential assumptions but they make the details simpler). Since we cannot use a genuine Brownian motion, we approximate it by a random walk consisting of a large number of independent and normally distributed jumps over equally spaced time periods ("steps"). We hold a portfolio consisting of a certain number of shares of stock and a short position in a risk-free bond, which means that the value of the bond is subtracted when computing the value of the portfolio. We purchase a starting portfolio consisting of some stock and a certain quantity of interest-paying bond, which we "short" (financial jargon for selling without owning). We then observe the movements of the stock at a certain number of points in time and adjust the portfolio we hold, by selling the old one and purchasing a new one at each of these points.
The cash flow of these transactions is shown in the picture on the rightâ€Âhand side. The portfolio is almost "self-financing", that is, the proceeds from the transactions almost exactly balance the costs of purchasing new portfolios. To obtain a genuinely self-financing portfolio we would need to adjust the portfolio at every moment in time, which in the case of a genuine continuous exponential Brownian motion is, of course, impossible. However, we can see that increasing the number of portfolio adjustments will tend to make the portfolio closer to a "self-financing" one (the cash flow curve on the lower right will lie closer to the horizontal axis). In practice, one needs a certain amount of cash to finance hedging operations, which, in the real world, also includes transaction costs.
Note that the final payoff from the option, which is equal to the greater of (a) the stock price at expiration minus the strike price and (b) zero, is almost equal to the final value of the hedging portfolio—as it should be. Thus the portfolio really "hedges" the European call option, in the sense that it always produces a payoff (almost) equal to that of the option. It follows that the initial value of the hedging portfolio must be (approximately) equal to the "fair value" of the option.
The entire argument can be viewed as a visual proof of the Black–Scholes formula, which is actually the main tool used in constructing the hedging portfolio.
Permanent Citation
"Hedging the Black-Scholes Call Option"
http://demonstrations.wolfram.com/HedgingTheBlackScholesCallOption/
Wolfram Demonstrations Project
Published: March 7 2011