The Price of a Call Option on Electrical Power

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
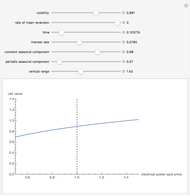
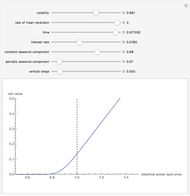
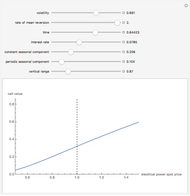
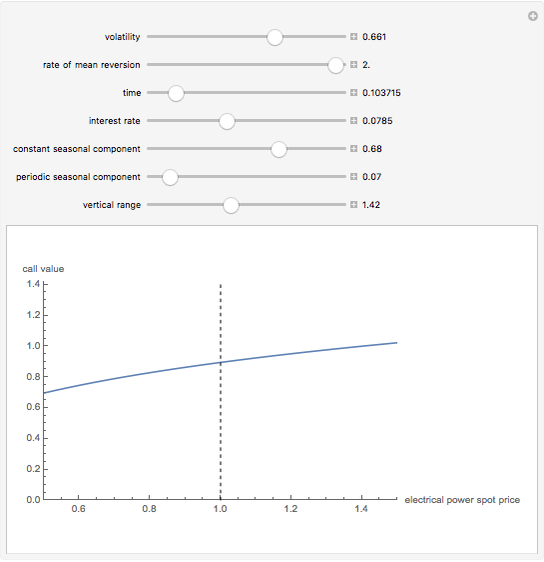
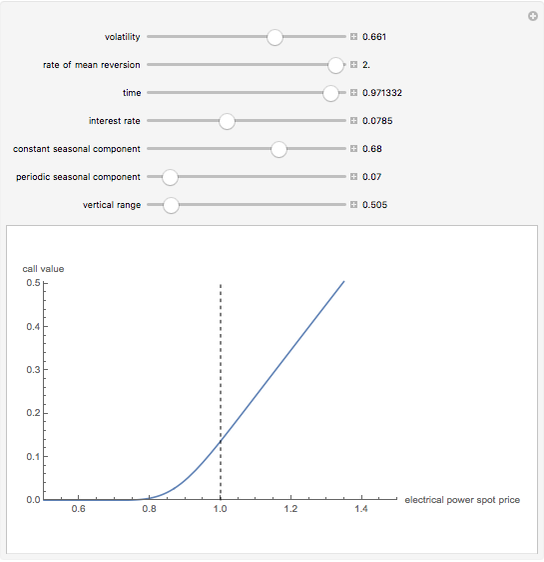
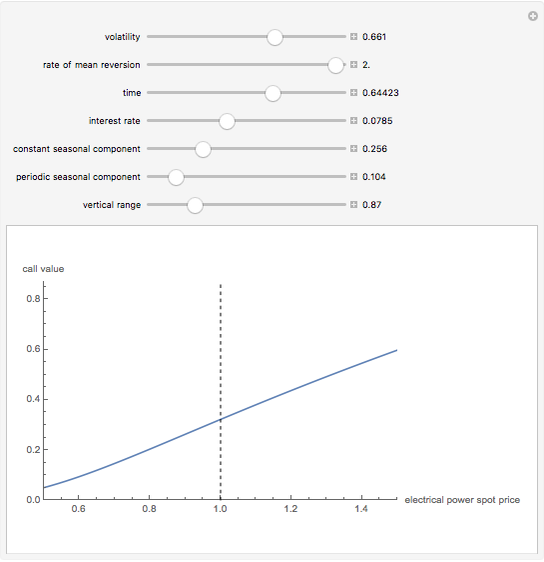
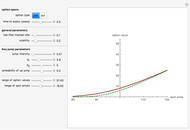
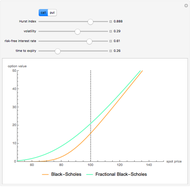
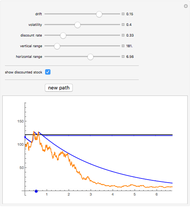
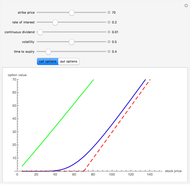
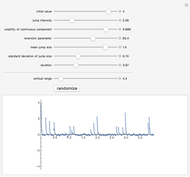
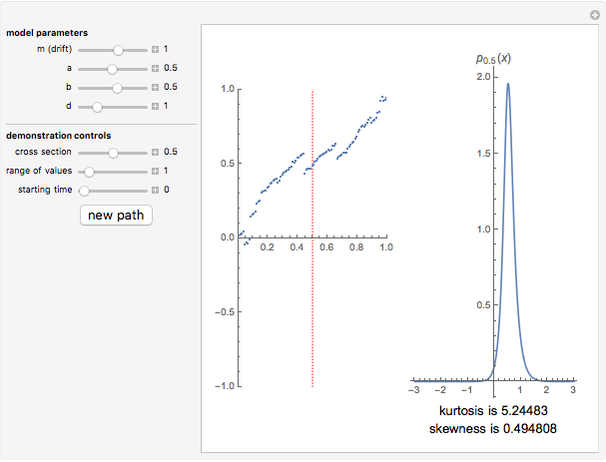
We demonstrate the formula for the value of a call option on electrical power obtained by Tino Kluge, which is analogous to the classical Black–Scholes formula. The model of spot price movements is described under a risk-neutral measure by  , where
, where  is a mean-reverting process satisfying
is a mean-reverting process satisfying  , and
, and  is Brownian motion. The deterministic seasonality function
is Brownian motion. The deterministic seasonality function  is supposed to capture all relevant components of the market that vary predictably with time. Here we use an oversimplified model,
is supposed to capture all relevant components of the market that vary predictably with time. Here we use an oversimplified model,  , where the trigonometric component reflects weekly periodicity (time
, where the trigonometric component reflects weekly periodicity (time  is measured in years, with 365 days per year and 7 days per week).
is measured in years, with 365 days per year and 7 days per week).
Contributed by: Andrzej Kozlowski (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
In many markets in various parts of the world deregulation of electric power has led to more competitive prices, but at the same time to higher uncertainty about future development. This in turn led to the introduction of derivative contracts such as options, intended to protect energy users from unexpected price spikes due to various seasonal and random factors. Models borrowed from financial markets, such as the Black–Scholes model, are not suitable for valuing options on electrical energy, as they lack the most important property such a model should have: mean reversion. The price of electrical energy (and other commodities) reflects the marginal cost of production and departs from this value due to various random and seasonal factors. When the influence of these temporary factors ceases, the price tends to revert to the mean. There is one additional important aspect of electricity prices: discontinuous random spikes in price due to unpredictable changes in weather or supply conditions. We ignore this aspect in this Demonstration for the sake of simplicity and particularly because we can then use a closed-form solution analogous to the one for Black–Scholes, given in [1] (where a much more sophisticated model, including spikes, is studied). The model used in here is a simplified version of Model 2 of [2], with the deterministic seasonal component reflecting only weekly seasonality and the difference between holidays and "peak" days disregarded.
[1] T. Kluge, Pricing Swing Options And Other Electricity Derivatives, Univ. Of Oxford D. Phil. thesis, 2006.
[2] J. Lucia and E. Schwartz, "Electricity Prices and Power Derivatives: Evidence from the Nordic Power Exchange," Review of Derivatives Research, 5(1), 2002 pp. 5–50.
Permanent Citation
"The Price of a Call Option on Electrical Power"
http://demonstrations.wolfram.com/ThePriceOfACallOptionOnElectricalPower/
Wolfram Demonstrations Project
Published: March 7 2011