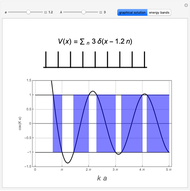
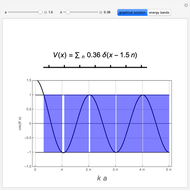
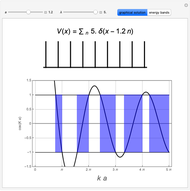
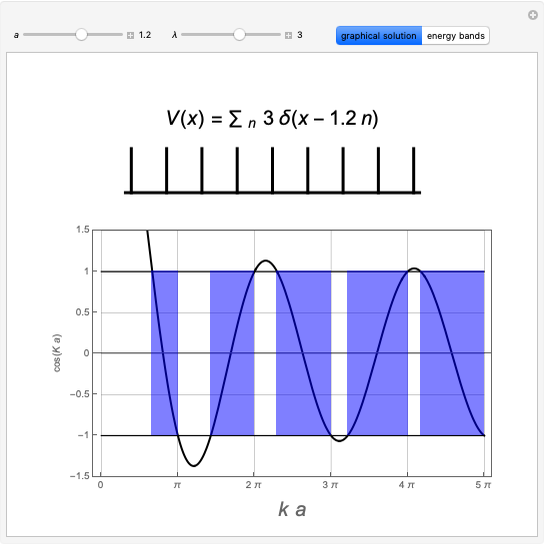
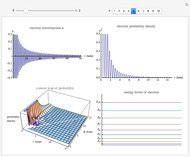
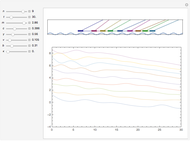
Kronig-Penney Model with Dirac Comb

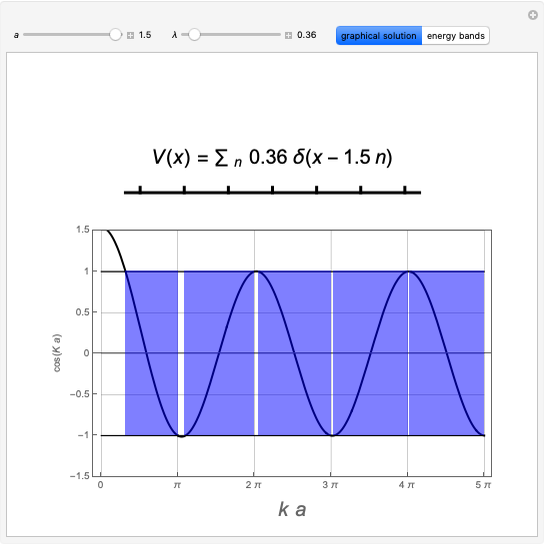
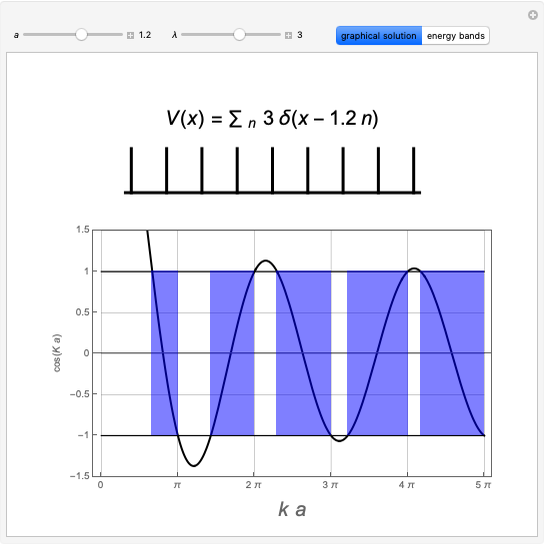
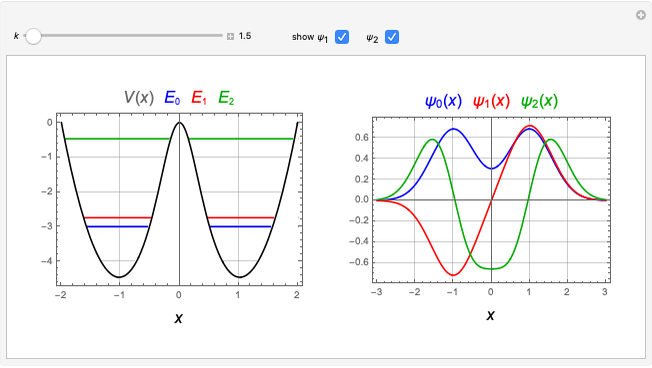
The simplest modification of the Kronig–Penney model for electrons in a one-dimensional periodic lattice can be based on a Dirac-comb potential approximating the positive cores:

.

Here, is the lattice spacing for an infinite row of delta functions. We consider the case of a repulsive potential, with . The Schrödinger equation (in atomic units ),

, with ,

can be solved exactly by applying Bloch's theorem, such that

,

where is the crystal momentum. As derived in the Details section, the allowed energies are then determined by the transcendental equation

.

Since , only values of fulfilling this condition are allowed. Selecting "graphical solution" shows these values of occupying a sequence of bands, shown in blue. This simple model thus simulates the electronic band structure of solids, which is essential for the understanding of insulators, conductors and semiconductors.

Select "energy bands" to see an idealized representation of the band structure, showing the first few energy bands separated by gaps. Completely filled bands generally make the material an insulator, since it takes considerable energy to excite an electron across a gap. However, if at least one band is partially filled, it takes less energy to excite an electron, and this material is typically a conductor. An insulator selectively doped with a few atoms can possibly create extra electrons or holes in a filled band, which results in a semiconductor, through which weak currents can flow.