DC and AC Josephson Effects

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
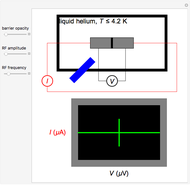
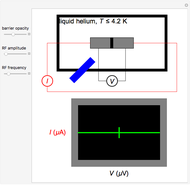
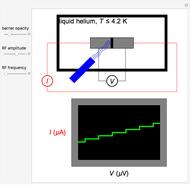
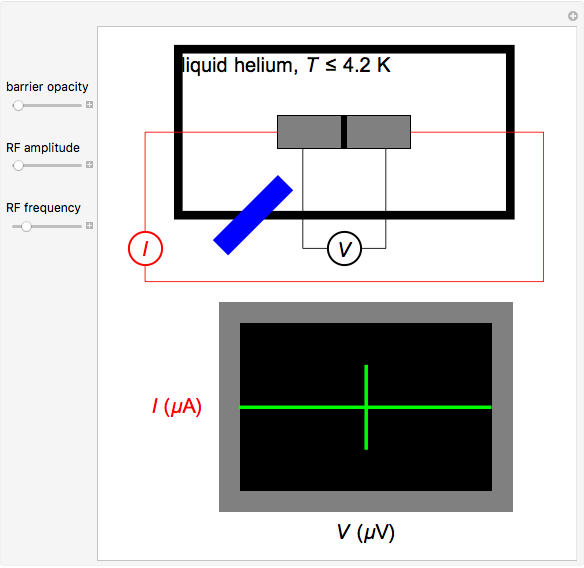
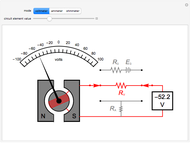
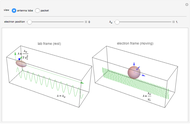
The upper graphic shows a Josephson junction: two superconductors, shown in gray, separated by a thin insulating barrier, all immersed in a liquid-helium cryostat. The junction is subjected to a DC voltage 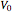 as well as a much lower-amplitude high-frequency AC voltage
as well as a much lower-amplitude high-frequency AC voltage 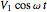 , produced by microwave radiation. The current
, produced by microwave radiation. The current 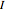 through the junction is monitored. By varying the radiation amplitude and frequency, as well as the barrier opacity, both the DC and the AC Josephson effects can be exhibited on the oscilloscope in the lower graphic. The voltage
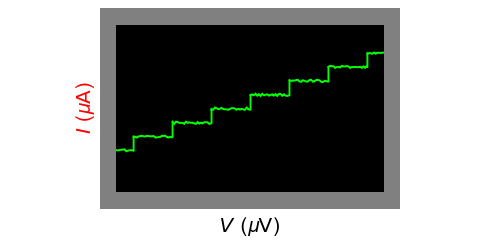
through the junction is monitored. By varying the radiation amplitude and frequency, as well as the barrier opacity, both the DC and the AC Josephson effects can be exhibited on the oscilloscope in the lower graphic. The voltage 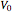 is swept across a range of several μV. The step structure shown is somewhat idealized, and will usually be much more irregular.
is swept across a range of several μV. The step structure shown is somewhat idealized, and will usually be much more irregular.
Contributed by: S. M. Blinder (January 2013)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Brian Josephson discovered in 1962 (and was awarded the Physics Nobel Prize in 1973) that Cooper pairs of superconducting electrons could tunnel through an insulating barrier, of the order of 10 Å wide, in the absence of any external voltage. This is to be distinguished from the tunneling of single electrons, which does require a finite voltage. This phenomenon is known as the DC Josephson effect.
A superconductor can be described by an order parameter, which is, in effect, the wavefunction describing the collective state of all the Cooper pairs. On the two sides of the barrier the wavefunctions can be represented by 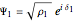 and
and 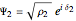 , respectively, in which
, respectively, in which 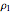 and
and 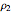 are Cooper-pair densities and
are Cooper-pair densities and 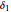 and
and 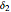 are the phases of the wavefunctions. The wavefunctions obey the coupled time-dependent Schrödinger equations
are the phases of the wavefunctions. The wavefunctions obey the coupled time-dependent Schrödinger equations
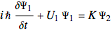 and
and 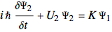 ,
,
where 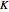 is a barrier-opacity constant that depends on the width and composition of the insulating barrier and the temperature. For superconductivity to be operative, the entire system is immersed in a liquid helium cryostat, so
is a barrier-opacity constant that depends on the width and composition of the insulating barrier and the temperature. For superconductivity to be operative, the entire system is immersed in a liquid helium cryostat, so 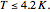 For simplicity, the two superconductors are assumed to have the same composition; niobium (Nb) is a popular choice. The energy difference
For simplicity, the two superconductors are assumed to have the same composition; niobium (Nb) is a popular choice. The energy difference  is
is 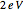 , where
, where 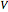 is the voltage across the junction and
is the voltage across the junction and 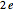 is the charge of a Cooper pair. The phase difference is given by
is the charge of a Cooper pair. The phase difference is given by 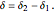 The current
The current 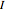 tunneling across the barrier is proportional to the time rate of change of the Cooper-pair densities
tunneling across the barrier is proportional to the time rate of change of the Cooper-pair densities 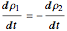 .
.
The preceding can be solved for the two fundamental equations for the Josephson effect:
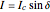 and
and 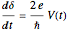 , where
, where 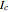 is the critical current, above which superconductivity is lost. The critical current depends on
is the critical current, above which superconductivity is lost. The critical current depends on 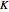 and also on any external magnetic field (which we assume absent here).
and also on any external magnetic field (which we assume absent here).
Suppose first that we apply a DC voltage 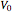 . The current is then given by
. The current is then given by 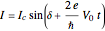 . Since
. Since  in the second term is so small, the argument of the sine is immensely large and time-averages to zero. Thus the tunneling supercurrent is zero if
in the second term is so small, the argument of the sine is immensely large and time-averages to zero. Thus the tunneling supercurrent is zero if 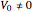 . Only when
. Only when 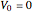 do we observe a current
do we observe a current 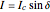 , with a maximum amplitude of
, with a maximum amplitude of 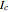 . The lower graphic shows an oscilloscope trace as
. The lower graphic shows an oscilloscope trace as 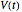 is swept over a range of the order of
is swept over a range of the order of 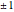 or
or 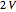 . This is, in essence, the DC Josephson effect, in which current flows only if
. This is, in essence, the DC Josephson effect, in which current flows only if 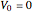 , but drops to zero if a DC voltage is applied. The "cross" at
, but drops to zero if a DC voltage is applied. The "cross" at 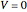 comes from superposed views of the supercurrent flowing in either direction in the course of each oscilloscope cycle.
comes from superposed views of the supercurrent flowing in either direction in the course of each oscilloscope cycle.
With a DC voltage 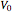 across the junction, the energy difference for a Cooper pair crossing the junction equals
across the junction, the energy difference for a Cooper pair crossing the junction equals 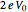 , which would correspond to a photon of frequency
, which would correspond to a photon of frequency 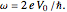 Such radiation has been measured with highly sensitive detectors.
Such radiation has been measured with highly sensitive detectors.
The AC Josephson effect is observed if the DC voltage is augmented by a small-amplitude, high-frequency AC contribution, such that 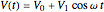 . When RF amplitude is zero, the system reverts to the DC effect. This is most readily accomplished by irradiating the junction with low-intensity microwave radiation in the range of 10–20 GHz. The junction current then given by
. When RF amplitude is zero, the system reverts to the DC effect. This is most readily accomplished by irradiating the junction with low-intensity microwave radiation in the range of 10–20 GHz. The junction current then given by
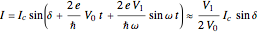 , for
, for 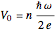 ,
,  , again neglecting sinusoidal voltages of unobservably high frequencies.
, again neglecting sinusoidal voltages of unobservably high frequencies.
As the voltage  is swept, those points at which the radiation frequency obeys the resonance condition
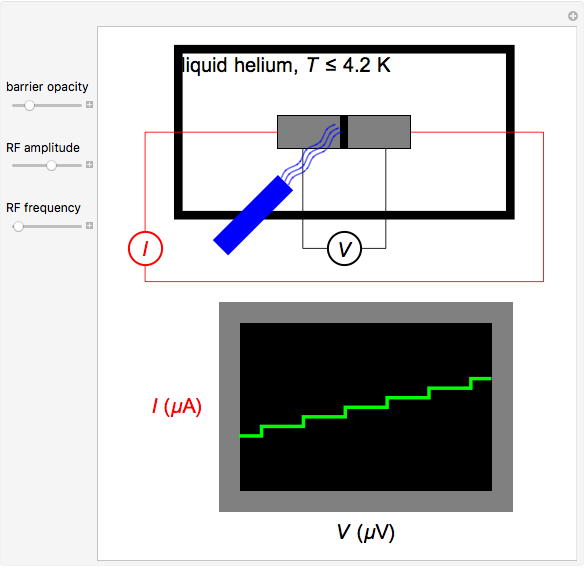
is swept, those points at which the radiation frequency obeys the resonance condition 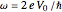 exhibit a series of stepwise increases in current, equal to
exhibit a series of stepwise increases in current, equal to 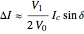 . These steps were first observed by S. Shapiro in 1963 and can be considered a definitive validation of the AC Josephson effect. The width of each voltage step is very precisely equal to
. These steps were first observed by S. Shapiro in 1963 and can be considered a definitive validation of the AC Josephson effect. The width of each voltage step is very precisely equal to 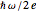 .
.
All plots are shown without numerical axes labels, since these magnitudes depend on the specific individual characteristics of instruments and materials. Thus, a qualitative descriptions of these phenomena suffices.
Josephson junctions have important applications in SQUIDs (superconducting quantum interference devices), which can be very sensitive magnetometers, and for RSFQ (rapid single flux quantum) digital electronic devices. They have been suggested in several proposed designs for quantum computers. The Josephson effect provides a highly accurate frequency to voltage conversion, as expressed by the Josephson constant, 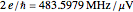 . Since frequency can be very precisely defined by the cesium atomic clock, the Josephson effect is now used as the basis of a practical high-precision definition of the volt.
. Since frequency can be very precisely defined by the cesium atomic clock, the Josephson effect is now used as the basis of a practical high-precision definition of the volt.
Snapshot 1: DC Josephson effect, with zero external voltage
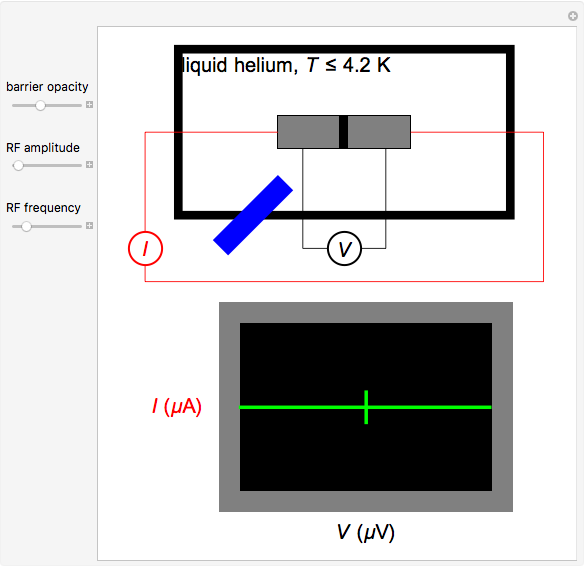
Snapshot 2: no tunneling when barrier is sufficiently opaque
Snapshot 3: oscilloscope trace for typical AC Josephson effect, showing Shapiro steps
References
[1] R. P. Feynman, R. B. Leighton, and M. Sands, The Feynman Lectures on Physics, Volume III, Reading, MA: Addison-Wesley, 1965 pp. 21–14 ff.
[2] Wikipedia: Josephson effect
Permanent Citation