Newton's Integrability Proof

Initializing live version

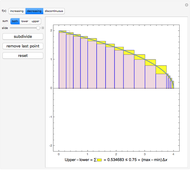
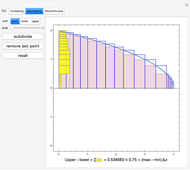
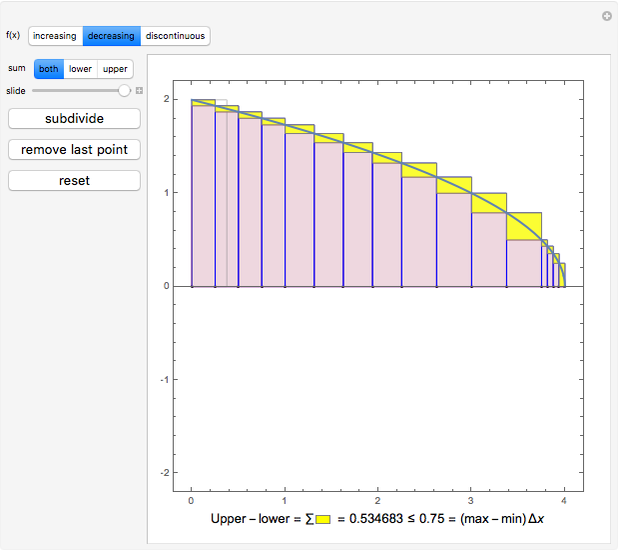
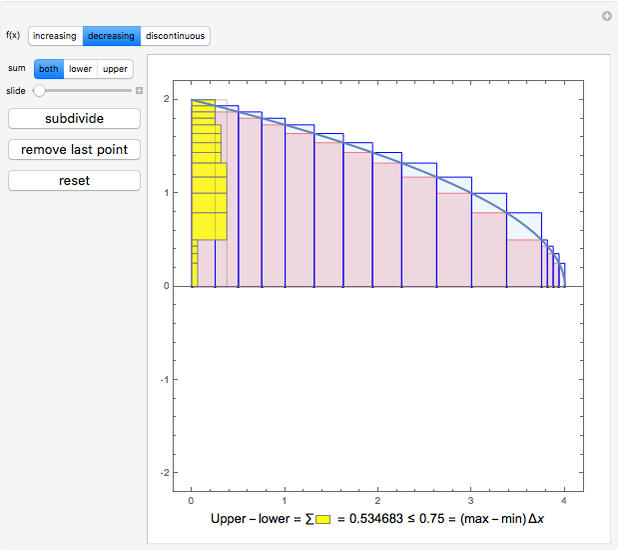
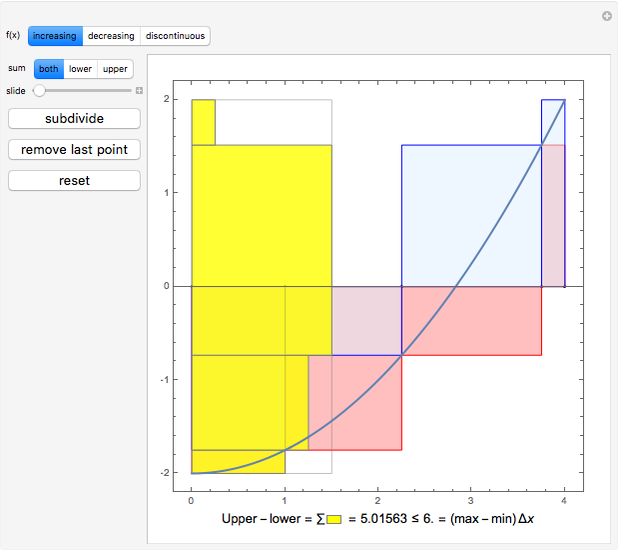
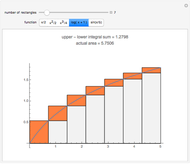
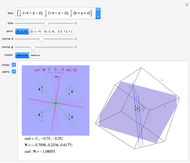
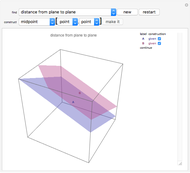
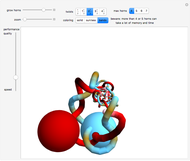
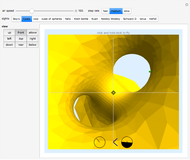
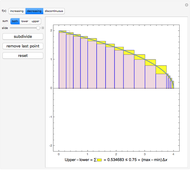
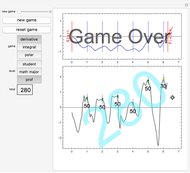
This figure is based on Newton's proof of the integrability of monotonic functions found in his Principia Mathematica (Book I, Lemma III). The error between the lower and upper sums, represented by the yellow rectangles, slides over and fits in a rectangle whose height is the height of the graph and width is that of the broadest yellow rectangle. As the partition is subdivided, the error approaches zero. In other words, the upper and lower sums approach the same value, the value of the integral of the function.

Contributed by: Michael Rogers (Oxford College/Emory University) (March 2011)
Open content licensed under CC BY-NC-SA

Snapshots

Details

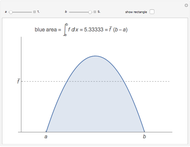
Click to add a point to the partition or click the subdivide button to divide each interval in half. The "slide" slider shows that the difference between the upper and lower sums fits inside a rectangle whose height is the difference in the values of at the ends of the interval and whose base is the width of the widest interval of the partition.

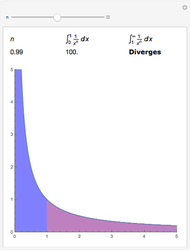
Snapshots 1 and 2: the decreasing function with the partition subdivided a couple of times, with the (yellow) difference rectangles in their original position and slid over inside the outlined rectangle, respectively

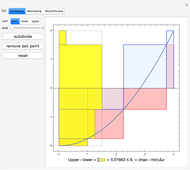
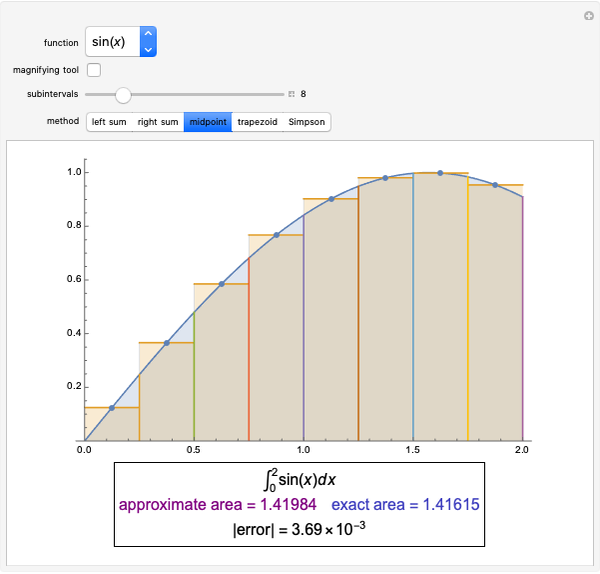
Snapshot 3: the increasing function with the (yellow) difference rectangles slid over inside the outlined rectangle

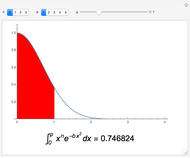
Snapshot 4: the discontinuous function with the (yellow) difference rectangles slid over inside the outlined rectangle

Permanent Citation

Michael Rogers (Oxford College/Emory University) "Newton's Integrability Proof"
http://demonstrations.wolfram.com/NewtonsIntegrabilityProof/
Wolfram Demonstrations Project
Published: March 7 2011


Feedback (field required)

Email (field required)	Name

Occupation	Organization

Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback. Send

Newton's Integrability Proof

Snapshots

Details

Related Links

Permanent Citation