Playing with Stellations of the Icosahedron

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
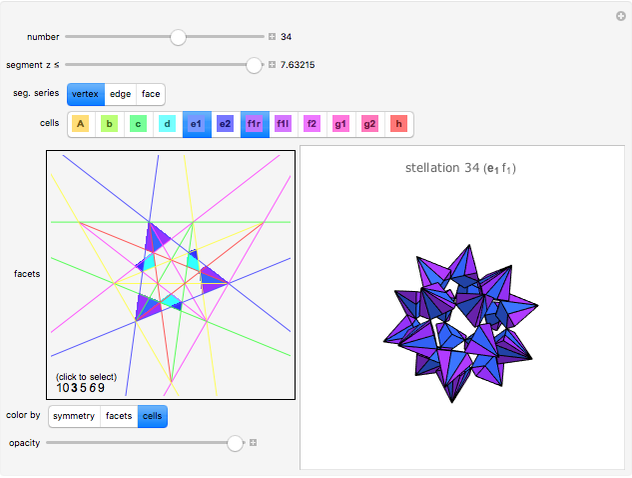
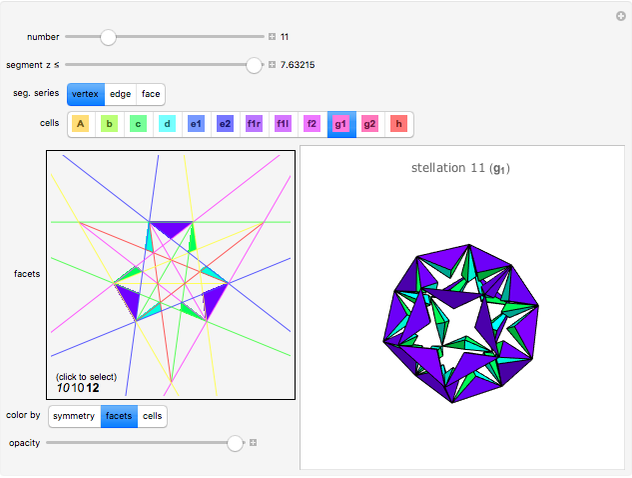
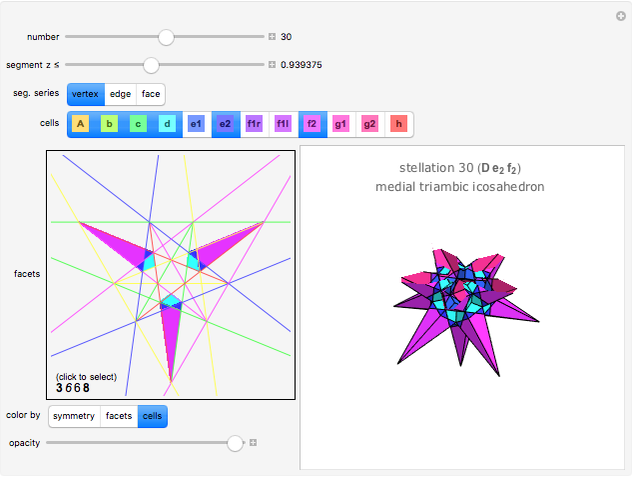
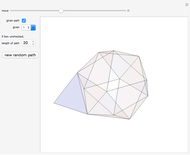
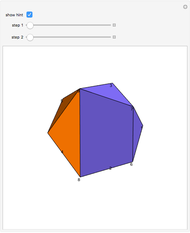
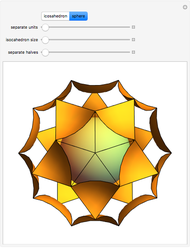
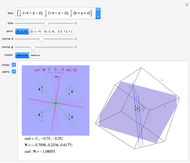
Play with the facets and cells of stellations of the icosahedron. Cut off a segment (along an axis through a vertex, edge, or face) and see inside. Different color schemes help identify the symmetry, facets, and cells of icosahedral stellations.
Contributed by: Michael Rogers (Oxford College/Emory University) (March 2011)
Open content licensed under CC BY-NC-SA
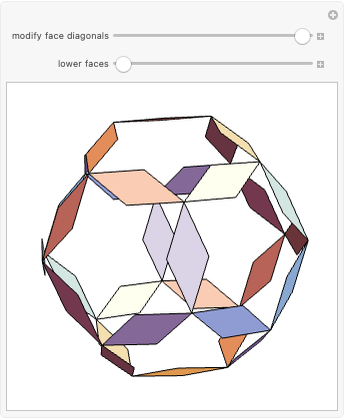
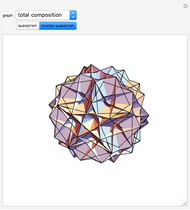
Snapshots
Details
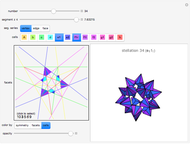
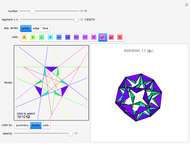
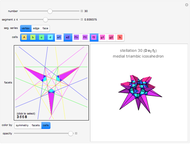
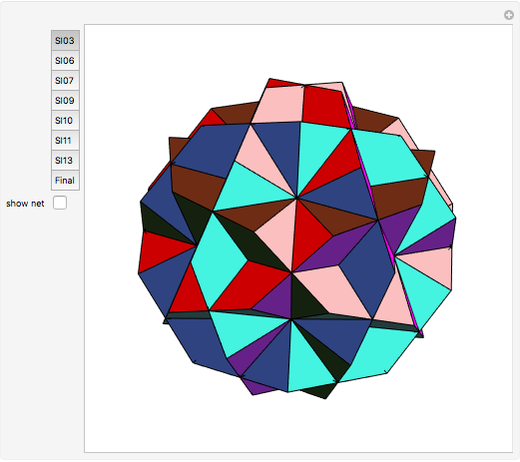
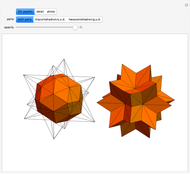
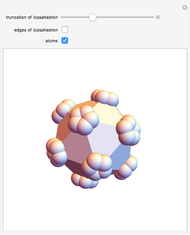
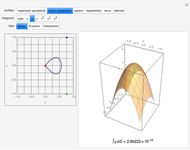
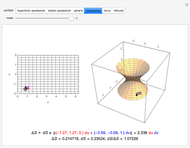
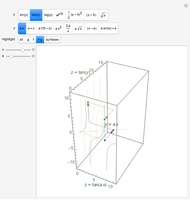
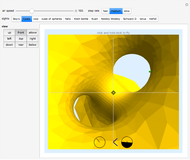
What is a stellation? Roughly, it's a bit of space contained by the face planes of the icosahedron that has the same rotational symmetry as the icosahedron. But that's not all. The planes containing the faces divide space into regions and they divide other face planes into polygons. The bounded regions are called "cells" and the bounded polygons "facets". But whether any symmetric selection of cells or any symmetric configuration of facets deserves to be called a stellation is, to some extent, a matter of taste. See [1] and [2] and use this Demonstration to get started on your own definition. Snapshots 1 and 2 show two stellations colored according to the cells and facets. Snapshots 3 and 4 show cut-away segments, the 4th revealing the faceting of the stellation.
This Demonstration was adapted from Mathematica code originally developed by Michael Rogers for a talk at Colby College in 1993.
[1] H. S. M. Coxeter, P. Du Val, H. T. Flather, and J. F. Petrie, The Fifty-Nine Icosahedra, Toronto: University of Toronto Press, 1938. Reprint, New York: Springer-Verlag, 1982.
[2] G. Inchbald, "In Search of the Lost Icosahedra," The Mathematical Gazette 86(506), July 2002 pp. 208-215.
Permanent Citation
"Playing with Stellations of the Icosahedron"
http://demonstrations.wolfram.com/PlayingWithStellationsOfTheIcosahedron/
Wolfram Demonstrations Project
Published: March 7 2011