WKB Computations on Morse Potential

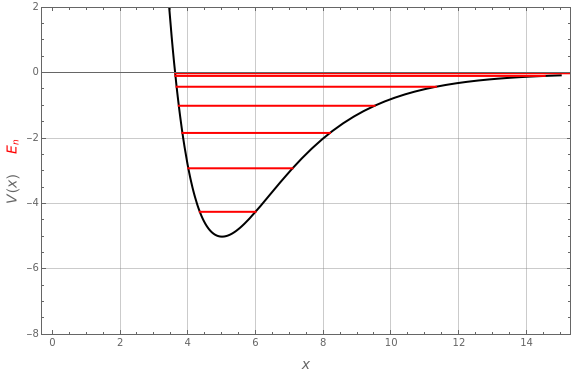
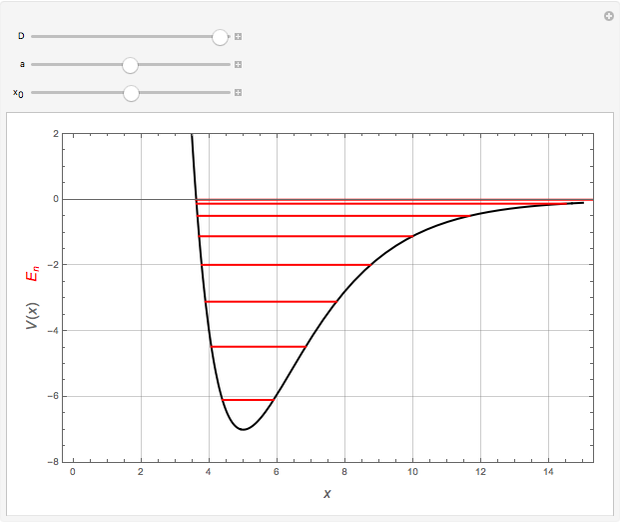
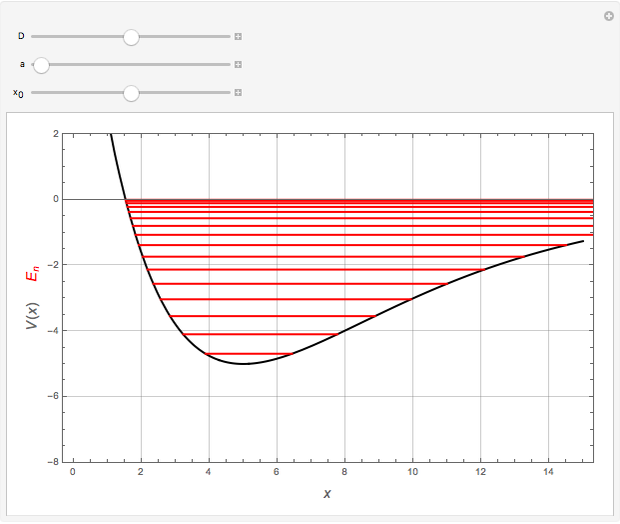
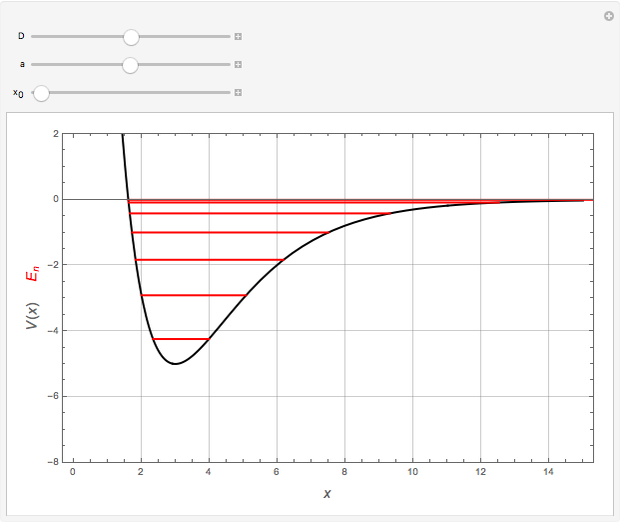
The semiclassical Wentzel–Kramers–Brillouin (WKB) method applied to one-dimensional problems with bound states often reduces to the Sommerfeld–Wilson quantization conditions, the cyclic phase-space integrals . It turns out that this formula gives the exact bound-state energies for the Morse oscillator with . The requisite integral can be reduced to , in which and are the classical turning points . The integral can be done "by hand", using the transformation followed by a contour integration in the complex plane, but Mathematica can evaluate the integral explicitly, needing only the additional fact that The result reads
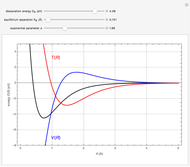
, which can be solved for to give , , in units with . The highest bound state is given by , where represents the floor, which for positive numbers is simply the integer part. The values of , , and (expressed in atomic units) used in this Demonstration are for illustrative purposes only and are not necessarily representative of any actual diatomic molecule.