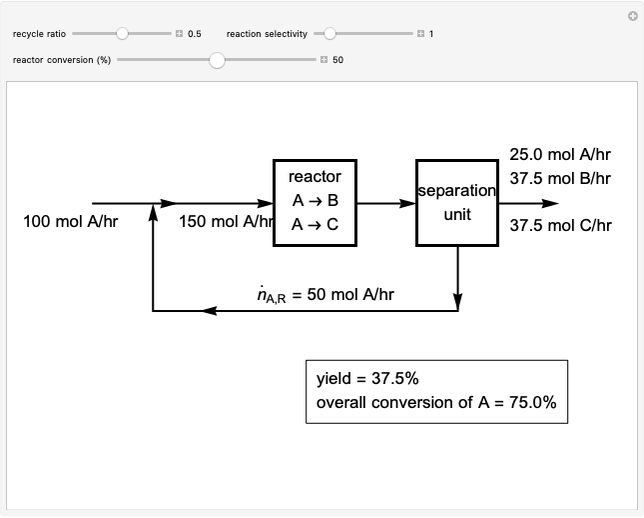
Fugacities in a Can of Soda

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
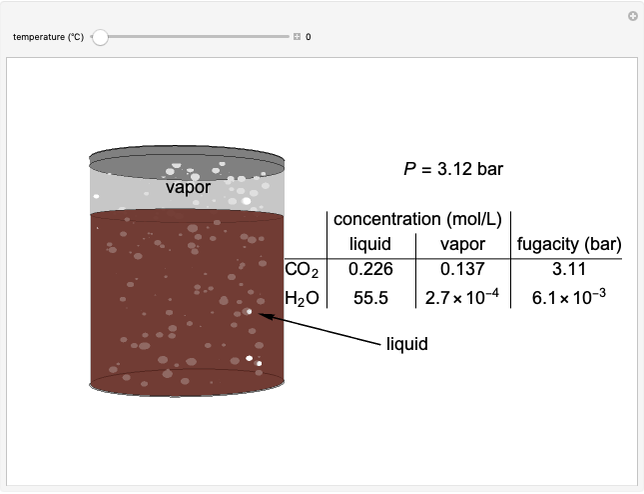
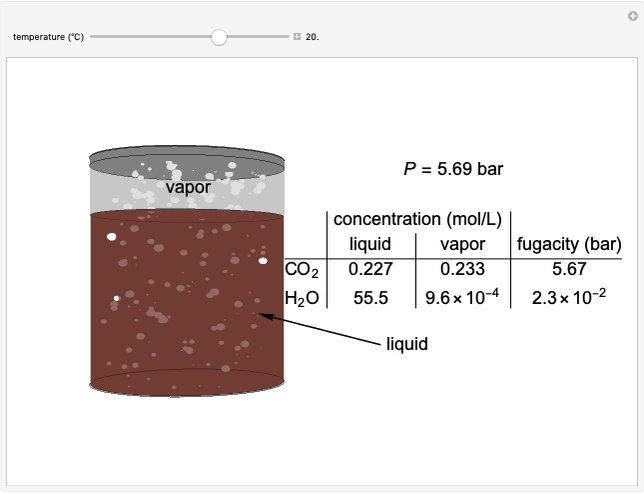
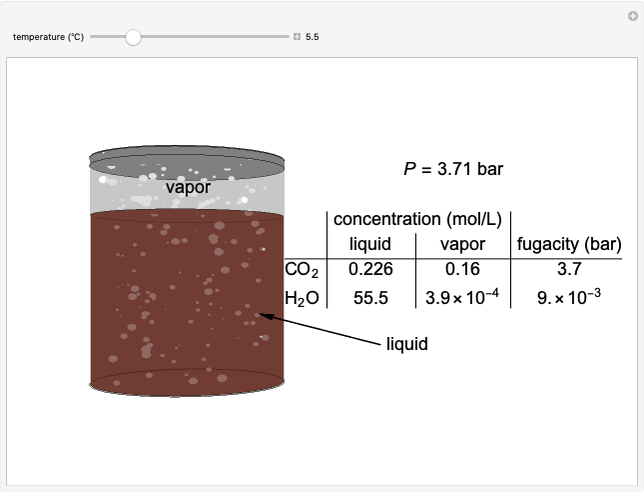
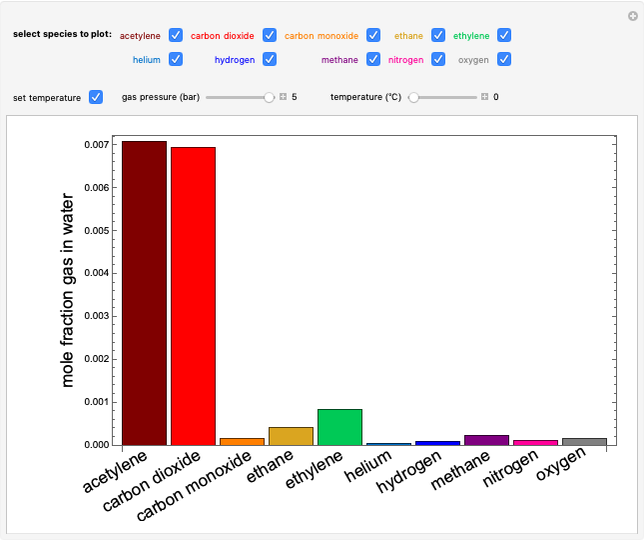
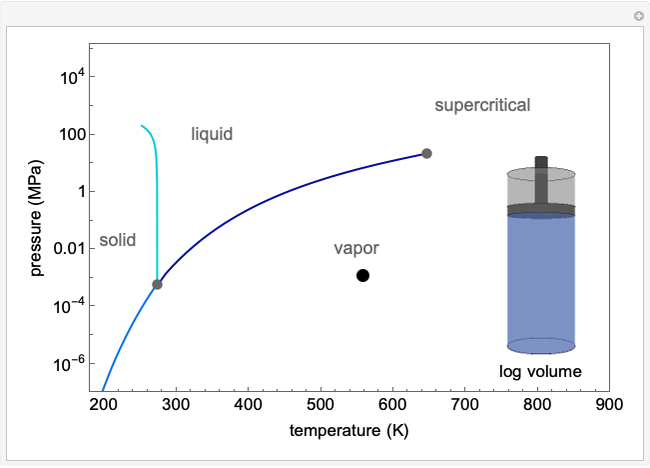
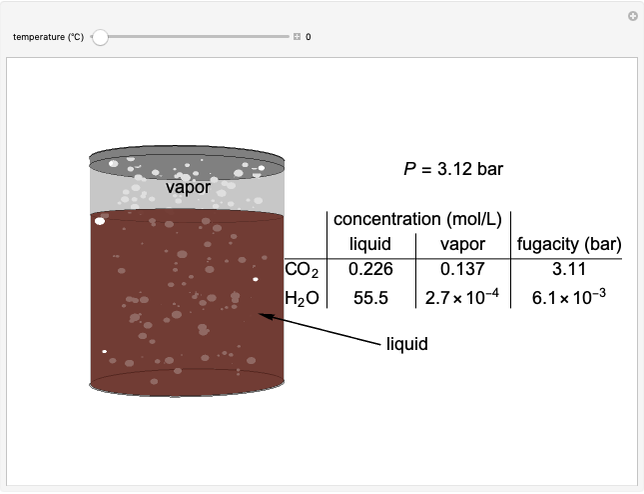
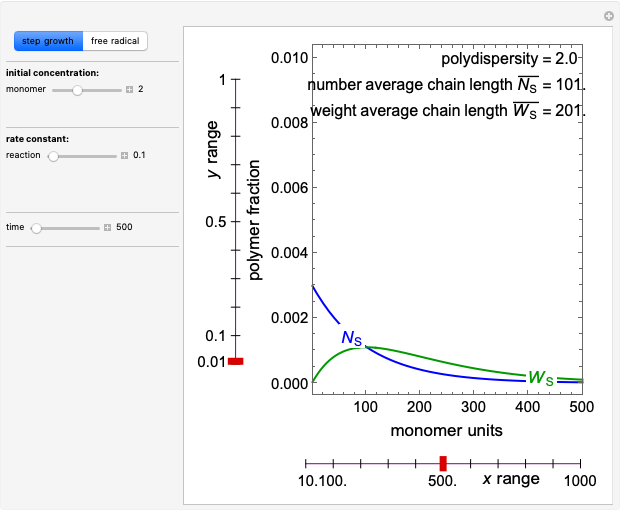
The fugacities of water and carbon dioxide are calculated as a function of temperature for a closed container, which is a model of a can of soda. The concentrations of the two components are calculated in both the liquid and gas phases. As temperature increases, the pressure increases, and therefore the fugacities increase. Note that the  concentration is much lower than the
concentration is much lower than the  concentration in the liquid phase, but the
concentration in the liquid phase, but the  concentration is much higher than the
concentration is much higher than the  concentration in the gas phase. Because the gas phase is assumed to be ideal, the fugacities of
concentration in the gas phase. Because the gas phase is assumed to be ideal, the fugacities of  and
and  in both phases are equal to their gas-phase partial pressures, and thus the
in both phases are equal to their gas-phase partial pressures, and thus the  fugacity is much higher than the
fugacity is much higher than the  fugacity. As the temperature increases, the
fugacity. As the temperature increases, the  concentration in liquid water decreases. However, as the temperature increases the pressure increases, and a higher
concentration in liquid water decreases. However, as the temperature increases the pressure increases, and a higher  pressure increases the
pressure increases the  concentration in water, so the net effect is that
concentration in water, so the net effect is that  concentration in the liquid phase does not change much as the temperature increases.
concentration in the liquid phase does not change much as the temperature increases.
Contributed by: Rachael L. Baumann (August 2014)
With additional contributions by: John L. Falconer and Nick Bongiardina
(University of Colorado Boulder, Department of Chemical and Biological Engineering)
Open content licensed under CC BY-NC-SA
Snapshots
Details
The fugacity of  dissolved in water was calculated using Henry's law and freezing point depression measurements:
dissolved in water was calculated using Henry's law and freezing point depression measurements:
 ,
,
 ,
,
 ,
,
 ,
,
where  is the difference between the freezing point of water and the freezing point of carbon dioxide in water (
is the difference between the freezing point of water and the freezing point of carbon dioxide in water ( and
and  ) (K),
) (K),  is the freezing point depression constant for water ([°C kg]/mol),
is the freezing point depression constant for water ([°C kg]/mol),  is the molality of
is the molality of  ,
,  is Henry's law constant (kg/[mol bar]),
is Henry's law constant (kg/[mol bar]),  is Henry's constant at 273 K,
is Henry's constant at 273 K,  is a constant,
is a constant,  is temperature (K),
is temperature (K),  is the partial pressure of
is the partial pressure of  (bar), and
(bar), and  is the fugacity of
is the fugacity of  (bar).
(bar).
The fugacity of water was calculated from the saturation pressure of water  using the Antoine equation:
using the Antoine equation:
 ,
,
where  is the fugacity of water (bar).
is the fugacity of water (bar).
The screencast video at [2] explains how to use this Demonstration.
References
[1] T. S. Kuntzleman and C. Richards, "Another Method for Determining the Pressure inside an Intact Carbonated Beverage Can (or Bottle)," Journal of Chemical Education, 87(9), 2010 p. 993. doi:10.1021/ed100255g.
[2] Fugacities in a Can of Soda [Video]. (Dec 16, 2020) www.learncheme.com/simulations/thermodynamics/thermo-2/fugacities-in-a-can-of-soda.
Permanent Citation