Hotelling Model of Product Quality Differentiation

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
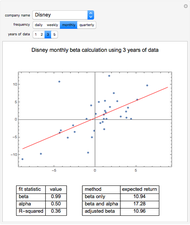
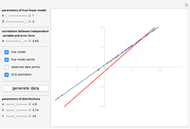
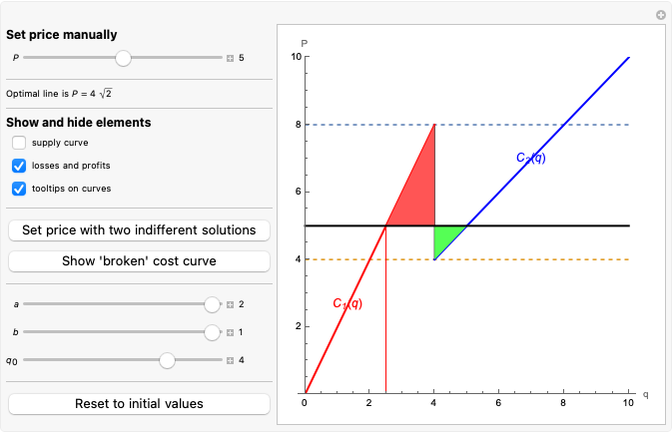
Consider a linear Hotelling model of product differentiation in terms of quality. It is straightforward to model any differentiation as a continuum of all possible qualities between 0 and 1. This interpretation of the original Hotelling location model (1929) is typical of the industrial organization branch of economic theory that studies market structure and competition.
[more]
Contributed by: Timur Gareev (May 2017)
Open content licensed under CC BY-NC-SA
Snapshots
Details
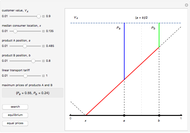
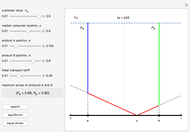
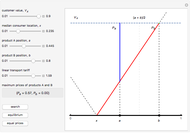
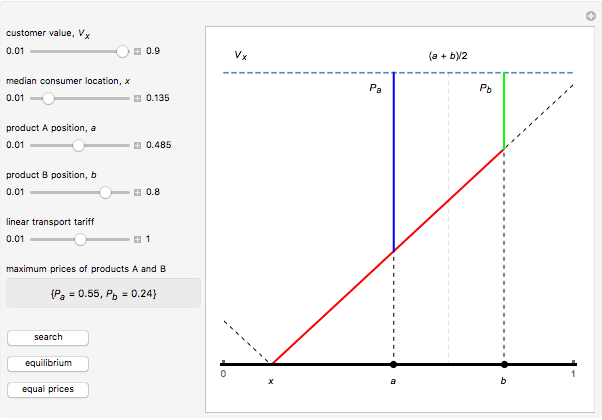
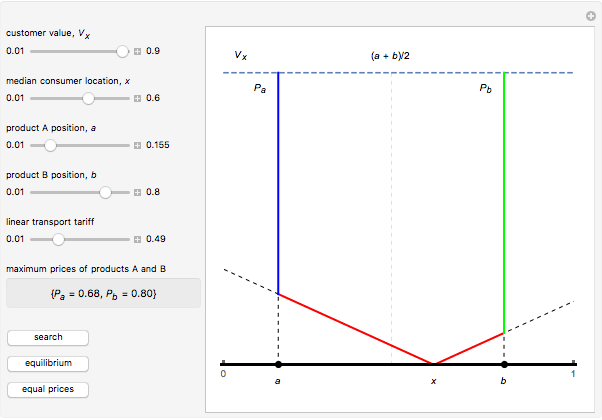
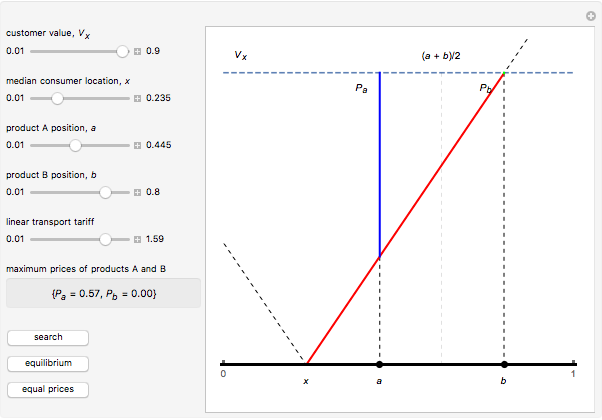
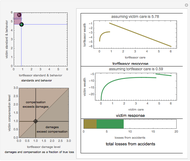
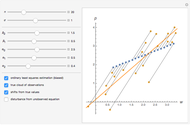
In a linear Hotelling model for product differentiation, consumers are supposed to locate uniformly within the quality continuum  . Each of two firms may choose its position of product with a certain quality (
. Each of two firms may choose its position of product with a certain quality ( and
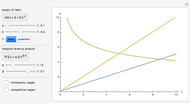
and  , respectively). The difference in quality characterizes "product differentiation". Products are in a sense substitutes and produced with zero marginal costs, so the producers are interested in charging higher prices.
, respectively). The difference in quality characterizes "product differentiation". Products are in a sense substitutes and produced with zero marginal costs, so the producers are interested in charging higher prices.
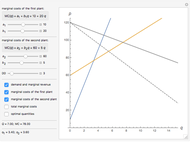
An arbitrary consumer has her value (reservation price) that she is ready to pay for a unit of product. An important feature of this model is that any consumer needs only one unit of product. A key idea of the linear model is that a consumer loses value proportional to the "distance" from her best quality (her location,  ) to the quality of the product that she buys. (Put differently, a product may have a higher price if it better matches the consumer's needs—that is, it is located closer to the consumer's location,
) to the quality of the product that she buys. (Put differently, a product may have a higher price if it better matches the consumer's needs—that is, it is located closer to the consumer's location,  ) The consumer is eager to pay for, say, a product of quality
) The consumer is eager to pay for, say, a product of quality  no more than
no more than  . So if a condition
. So if a condition  holds, the consumer is indifferent between the goods
holds, the consumer is indifferent between the goods  and
and  . Competition in this model means moving quality closer to a median consumer. (Note that the concept of median consumer simply means that half of all consumers are positioned to the left and half to the right of the consumer's position
. Competition in this model means moving quality closer to a median consumer. (Note that the concept of median consumer simply means that half of all consumers are positioned to the left and half to the right of the consumer's position  in the quality space). The implications of the model derive from its constricting assumptions that the transport tariff is linear and customers only buy a unit of the product. This considerably simplifies the algebra involved and results in explicit solutions.
in the quality space). The implications of the model derive from its constricting assumptions that the transport tariff is linear and customers only buy a unit of the product. This considerably simplifies the algebra involved and results in explicit solutions.
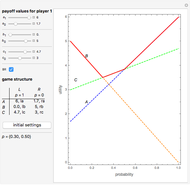
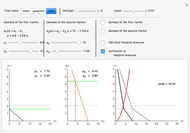
It is interesting that the model predicts a unique Nash equilibrium in the one-short game (if firms know where the median consumer resides), or if we assume a multistage game (where the firms may change quality costless).
You can arbitrarily set producers ( and
and  ) and the consumer (
) and the consumer ( ) locations, customer value
) locations, customer value  and the transport tariff
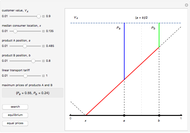
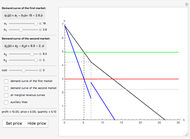
and the transport tariff  with sliders. Then the button "search" will show how producers choose product quality in a multistage game (with a certain number of steps to equilibrium). Click "equilibrium" to see the one-stage final result (this is less intriguing, though). Click "equal prices" to shift the median consumer location
with sliders. Then the button "search" will show how producers choose product quality in a multistage game (with a certain number of steps to equilibrium). Click "equilibrium" to see the one-stage final result (this is less intriguing, though). Click "equal prices" to shift the median consumer location  so that it is at the quality position
so that it is at the quality position  , midway between
, midway between  and
and  .
.
Permanent Citation