Nondiscriminating Monopolist with Two Independent Markets

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
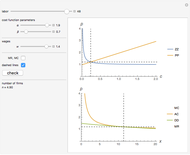
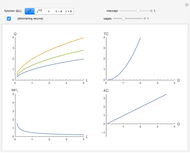
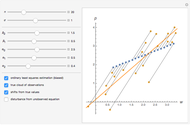
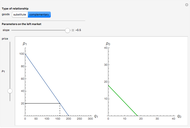
This Demonstration studies an important case in industrial organization: how a nondiscriminating monopolist sets a uniform price in two independent markets. We get the total demand curve by "horizontal summation" of two independent linear demand curves, which arises because of the convention that for demand function  , the dependent variable
, the dependent variable  is plotted along the horizontal axis. As a result, the monopolist faces a broken and discontinuous total marginal revenue curve
is plotted along the horizontal axis. As a result, the monopolist faces a broken and discontinuous total marginal revenue curve  , which is a function of
, which is a function of  . This shows the model's difference from the other important case of a discriminating monopolist.
. This shows the model's difference from the other important case of a discriminating monopolist.
Contributed by: Timur Gareev (November 2015)
Immanuel Kant Baltic Federal University
Open content licensed under CC BY-NC-SA
Snapshots
Details
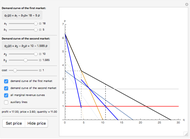
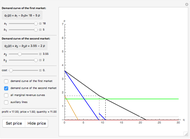
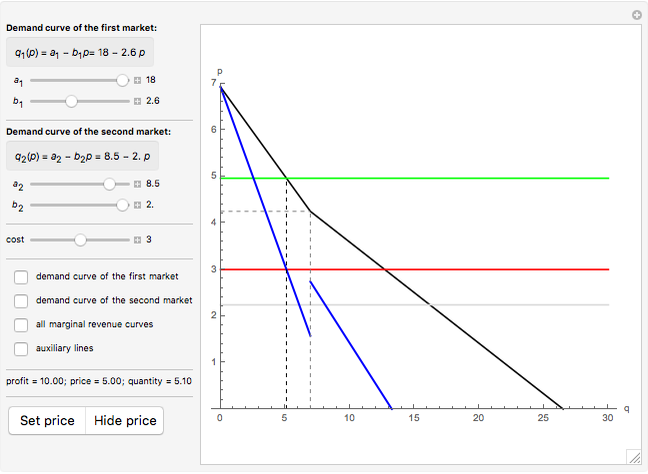
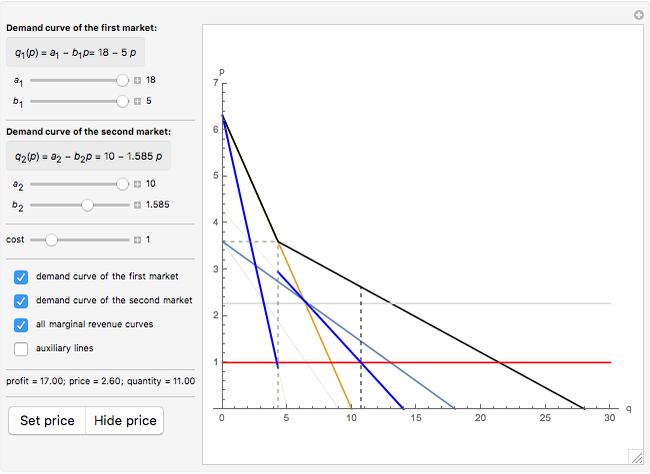
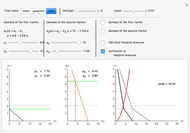
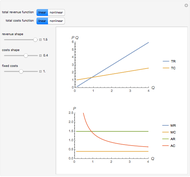
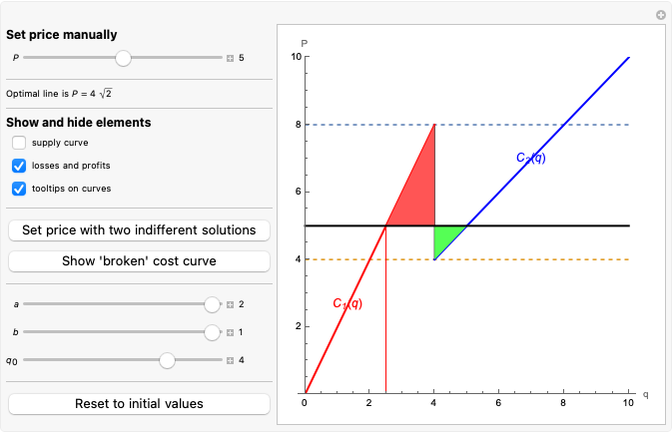
Suppose a monopolist faces two independent markets. In the first market, demand is  , and in the second market, demand is
, and in the second market, demand is  . The monopolist solves the typical problem of setting the price (in conjunction with volume of production) so as to maximize total profit collected from both markets. For whatever reason (legal, technological, marketing, etc.), she is nondiscriminating, which means she sets the unified price
. The monopolist solves the typical problem of setting the price (in conjunction with volume of production) so as to maximize total profit collected from both markets. For whatever reason (legal, technological, marketing, etc.), she is nondiscriminating, which means she sets the unified price  for both markets. As you can see, the monopolist deals with a total demand curve that is piecewise due to the "horizontal summation" of two linear demand curves, because the dependent variable
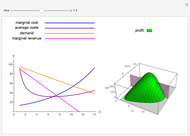
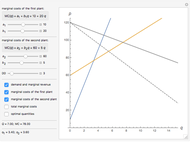
for both markets. As you can see, the monopolist deals with a total demand curve that is piecewise due to the "horizontal summation" of two linear demand curves, because the dependent variable  is plotted along the horizontal axis. It is also instructive to study the total marginal revenue curve that is discontinuous in the case of a broken total demand curve (use hide/show checkboxes to see how to get it). Such behavior of total marginal revenue poses a problem: how to define the optimal price if we have two pieces of
is plotted along the horizontal axis. It is also instructive to study the total marginal revenue curve that is discontinuous in the case of a broken total demand curve (use hide/show checkboxes to see how to get it). Such behavior of total marginal revenue poses a problem: how to define the optimal price if we have two pieces of  that could be equated with a single cost curve. To resolve this problem, we find the indifference level of costs. If the marginal cost curve lies below that level, we use the right piece of
that could be equated with a single cost curve. To resolve this problem, we find the indifference level of costs. If the marginal cost curve lies below that level, we use the right piece of  , and the left piece otherwise. To ease profit calculation, the monopolist's production technology is characterized by constant marginal costs (the red curve is given and can be controlled). All optimal solutions (total profit, price, and quantity) are calculated dynamically on the control panel. Click the "Set price" button to show the optimal price level.
, and the left piece otherwise. To ease profit calculation, the monopolist's production technology is characterized by constant marginal costs (the red curve is given and can be controlled). All optimal solutions (total profit, price, and quantity) are calculated dynamically on the control panel. Click the "Set price" button to show the optimal price level.
Permanent Citation