Inhibition of Enzyme Reactions in Continuous Stirred-Tank Reactor and Batch Reactor

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
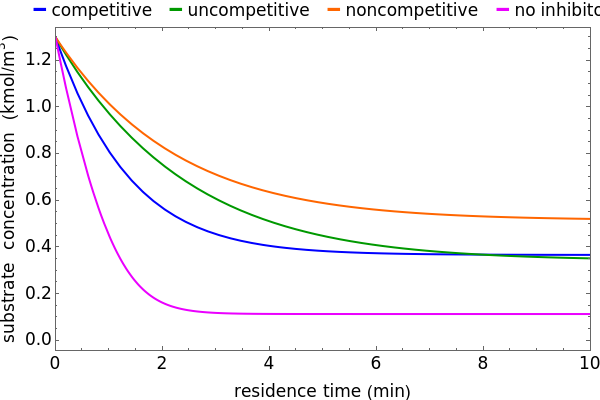
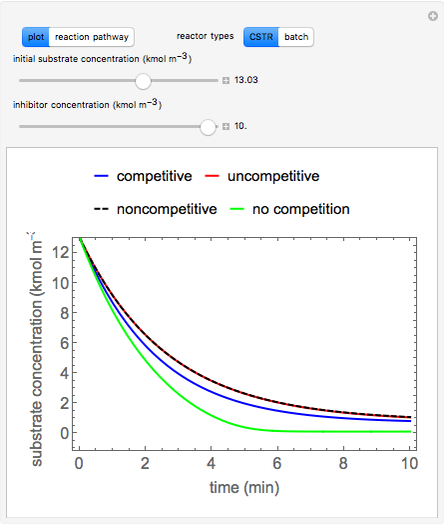
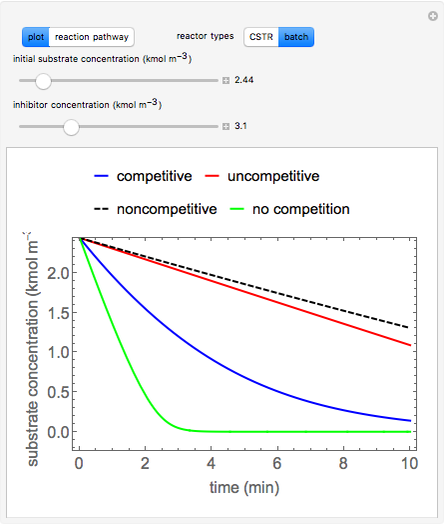
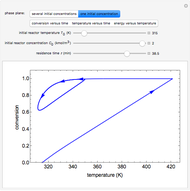
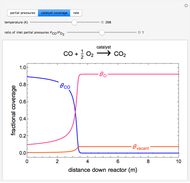
Inhibitors decrease the rates that enzymes bind to substrates and convert them to products. Three types of inhibition (competitive, uncompetitive, noncompetitive/mixed) and reaction with no inhibitor are compared for an enzyme reaction that obeys Michaelis–Menten kinetics. Substrate concentration is plotted versus time for a continuous stirred-tank reactor (CSTR) and for a batch reactor. Change the inhibitor concentration, which remains constant during reaction, with a slider. Set the substrate feed concentration with a slider. Select "inhibition type" to see the reaction steps, pathways, and a brief description of the type of inhibition. In competitive inhibition, the inhibitor attacks the enzyme to form an enzyme-inhibitor complex 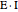 ; adding more substrate minimizes the inhibitor effect. In uncompetitive inhibition, the inhibitor attacks the enzyme-substrate complex
; adding more substrate minimizes the inhibitor effect. In uncompetitive inhibition, the inhibitor attacks the enzyme-substrate complex 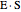 ; adding more substrate does not overcome this type of inhibition since the inhibitor does not compete with the substrate for the enzyme sites. In noncompetitive inhibition, the inhibitor attacks the enzyme to form an enzyme-inhibitor complex
; adding more substrate does not overcome this type of inhibition since the inhibitor does not compete with the substrate for the enzyme sites. In noncompetitive inhibition, the inhibitor attacks the enzyme to form an enzyme-inhibitor complex 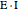 or it attacks the enzyme-substrate complex
or it attacks the enzyme-substrate complex 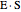 to form an enzyme-substrate-inhibitor complex
to form an enzyme-substrate-inhibitor complex 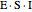 .
.
Contributed by: Rachael L. Baumann (April 2014)
Additional contributions by: John L. Falconer and Nick Bongiardina
(University of Colorado Boulder, Department of Chemical and Biological Engineering)
Open content licensed under CC BY-NC-SA
Snapshots
Details
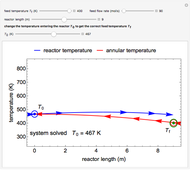
For a continuous-stirred tank reactor (CSTR) the material balance is written in the form of a differential equation:
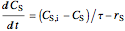 ,
,
for a batch reactor the material balance is:
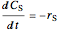 ,
,
where 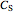 is substrate concentration (
is substrate concentration (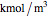 ),
), 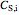 is the feed substrate concentration (
is the feed substrate concentration (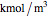 ),
), 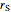 is the rate of substrate consumption for various types of inhibition (
is the rate of substrate consumption for various types of inhibition (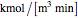 ),
),  is residence time (min), and
is residence time (min), and  is time (min).
is time (min).
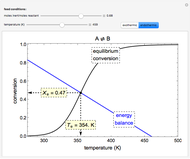
Rate laws are different for each type of inhibition:
competitive: 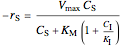 ,
,
uncompetitive: 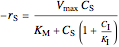 ,
,
noncompetitive (mixed): 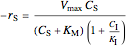 ,
,
no inhibitor: 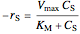 ,
,
where 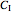 is inhibitor concentration (
is inhibitor concentration (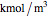 ),
), 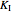 and
and 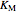 are inhibitor and Michaelis constants (
are inhibitor and Michaelis constants (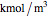 ), and
), and 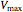 is the rate of reaction (
is the rate of reaction (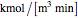 ).
).
Reference
[1] H. S. Fogler, Essentials of Chemical Reaction Engineering, Boston: Pearson Education, 2011 pp. 349–370.
Permanent Citation