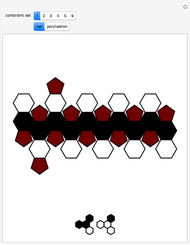
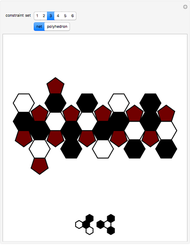
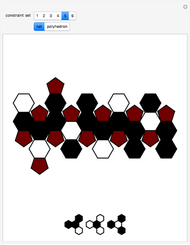
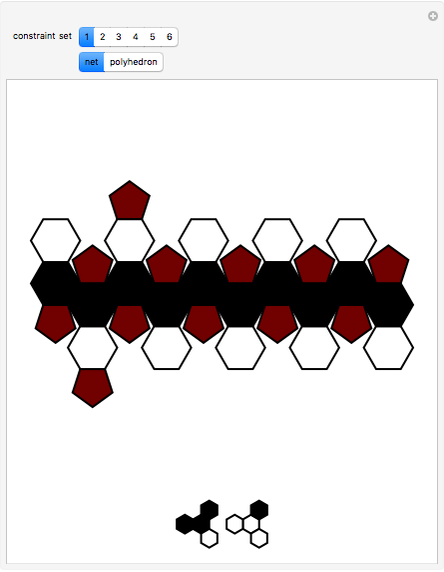
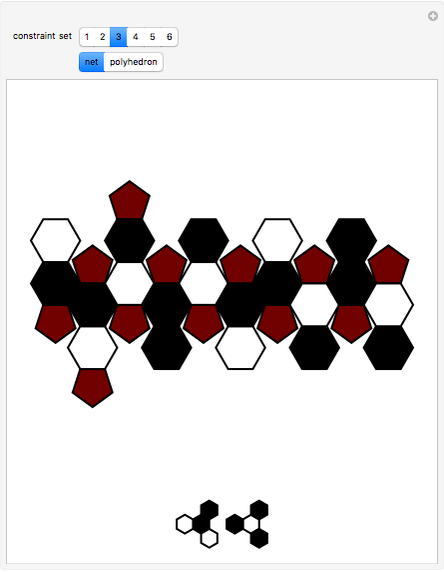
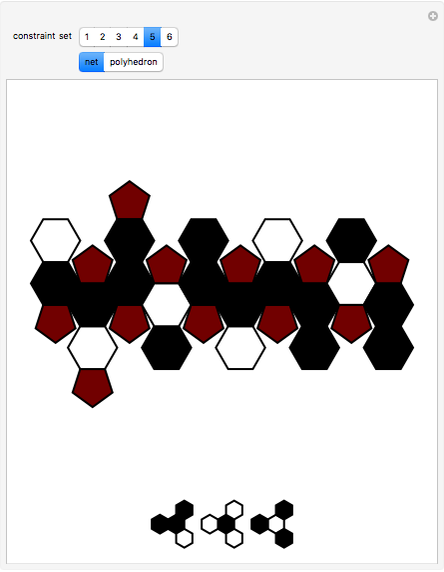
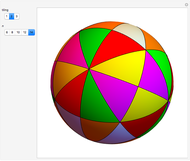
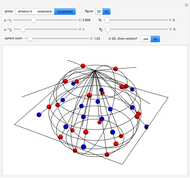
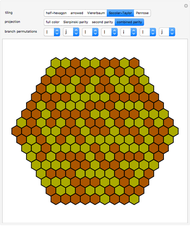
Sets of local constraints can force arrangements of color into ordered patterns (A New Kind of Science, Chapter 5, Systems Based on Constraints (NKS|Online)). Three sets of two constraint-based colorings show parity symmetry under exchange of black and white. With this constraint template, exactly five constraint sets exist so that all twenty hexagons can be colored black or white without contradicting the constraints evaluated on any face. Two sets of two-constraint sets show alternating parity as with the resulting colorings. The remaining constraint set shows identity parity: each of the two-constraint set elements becomes the other after an exchange of black and white. This remarkable constraint set permits two equally preferable colorings.