Pricing Put Options with the Binomial Method

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
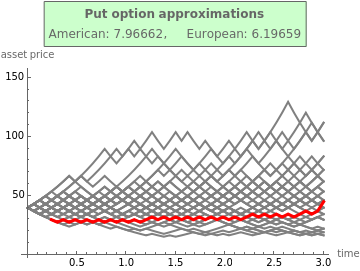
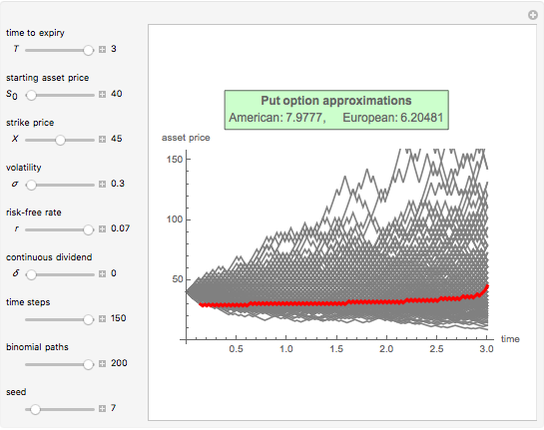
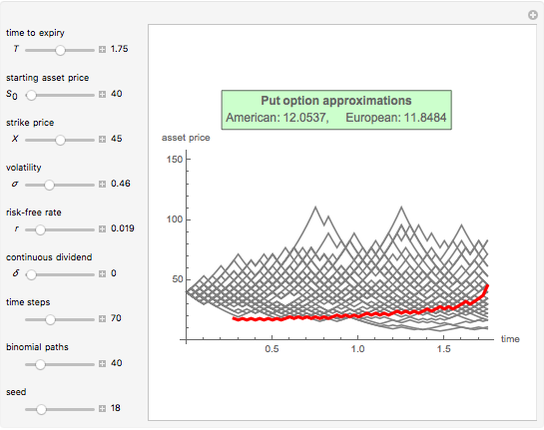
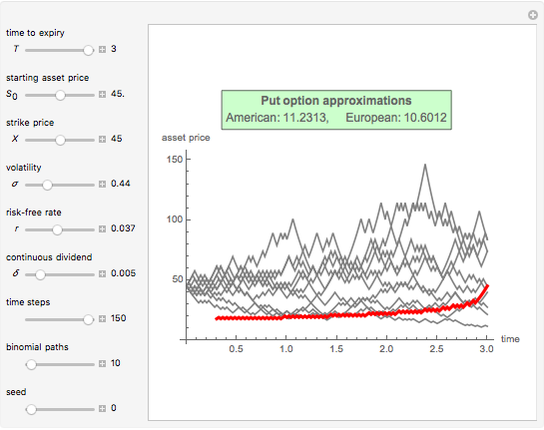
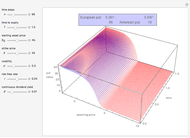
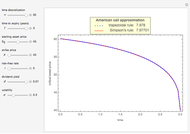
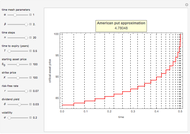
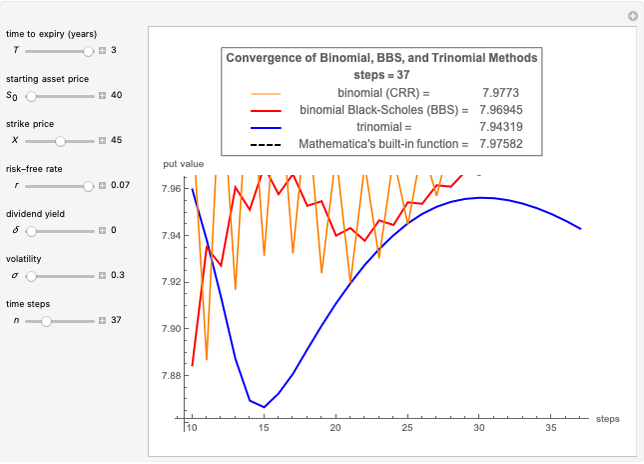
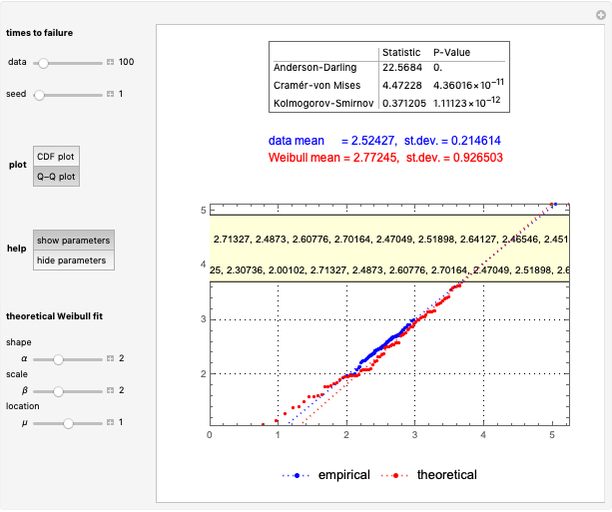
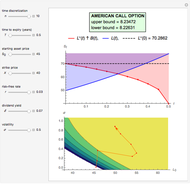
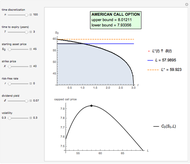
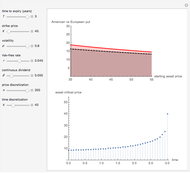
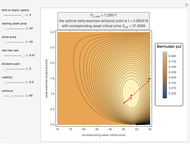
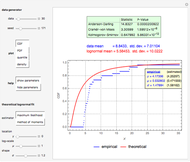
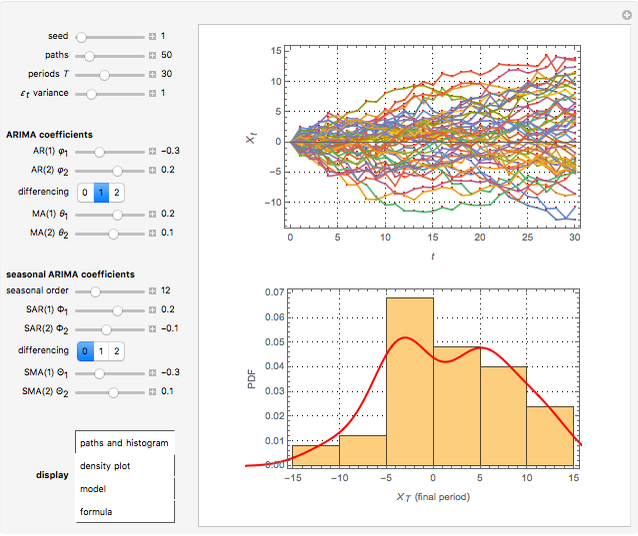
This Demonstration applies the binomial method [1] to estimate the value of a put option. Use the controls to set the option's parameters and the time discretization to approximate the American and the European put. The European put can be exercised only at its maturity, while the American put can be exercised at any time up to maturity. The red line represents the early exercise boundary for the American put. Whenever the asset price drops below this boundary, the American put's intrinsic value becomes greater than its holding value and it is optimal for the holder to exercise the option. Random binomial paths show where the asset price is more likely to move.
Contributed by: Michail Bozoudis (July 2014)
Suggested by: Michail Boutsikas
Open content licensed under CC BY-NC-SA
Snapshots
Details
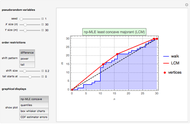
Under the binomial method [1], the underlying asset price is modeled as a recombining tree, where at each node the price can go up and down. These values are found by multiplying the value at the current node by the appropriate factor  or
or 
• 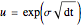
• 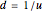
with corresponding probabilities
• 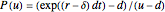
• 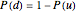
To make sure that these probabilities are in the interval 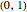 , the condition
, the condition 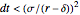 should be satisfied.
should be satisfied.
Once the binomial lattice of all possible asset prices up to maturity has been calculated, the option value is found at each node by working backward from the final nodes to present.
The difference between the binomial and trinomial model [2] is that the option value at each non-final node is determined based on the two later nodes and their corresponding probabilities (as opposed to three). The binomial model is considered to produce less-accurate results than the trinomial model when fewer time steps are modelled.
Reference
[1] J. Cox, S. Ross, and M. Rubinstein, "Option Pricing: A Simplified Approach," Journal of Financial Economics, 7(3), 1979 pp. 229–263.
[2] P. Boyle, "Option Valuation Using a Three Jump Process," International Options Journal, 3, 1986 pp. 7–12.
Permanent Citation