Tail Conditional Expectations

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
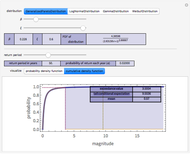
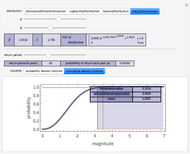
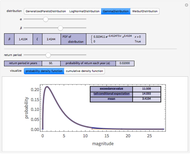
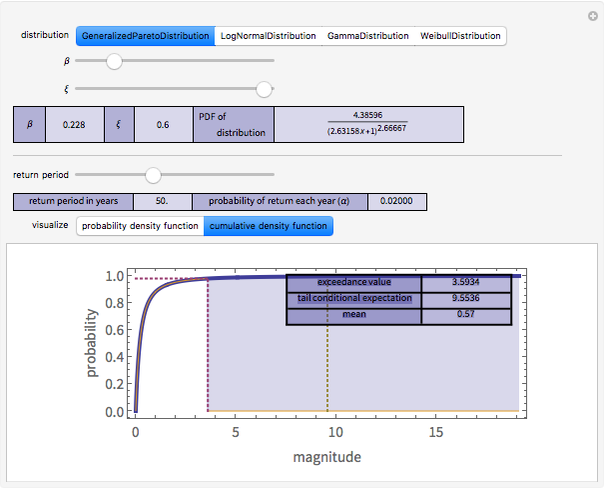
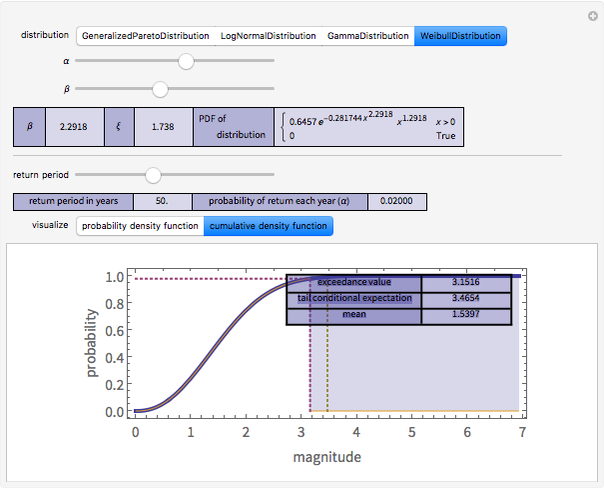
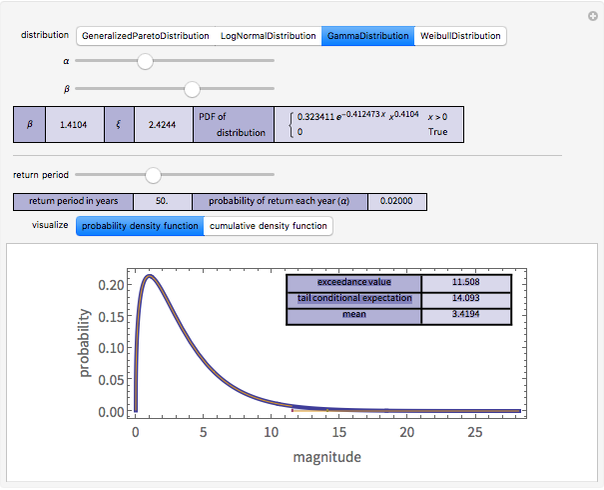
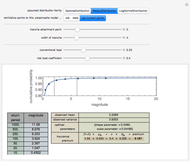
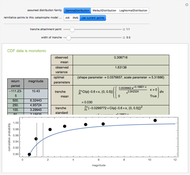
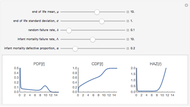
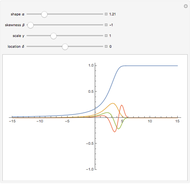
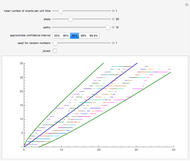
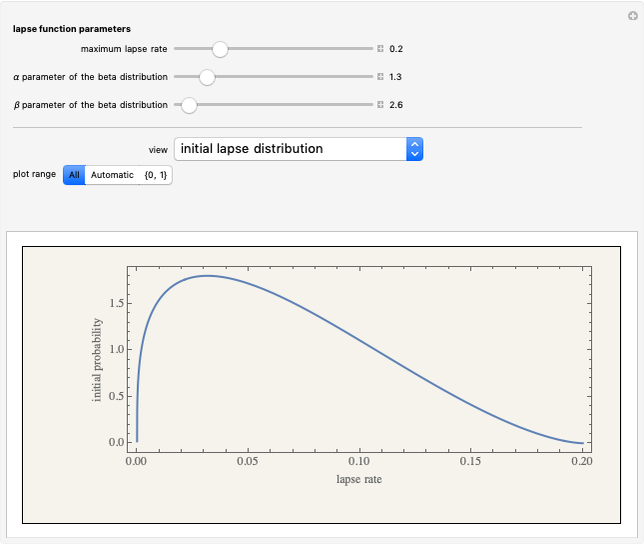
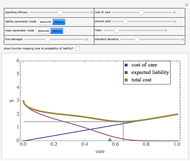
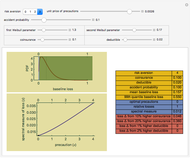
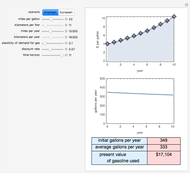
In modeling catastrophes, it is often useful to know how large a loss can be expected to be, given the assumption that the loss is greater than some amount. This value—the so-called "tail conditional expectation"—may be of great relevance to those who insure or reinsure against the highest levels of losses; it is sometimes what is meant by the actuarial term "probable maximum loss". In this Demonstration, you select a family of distributions and two parameters that instantiate a particular distribution from that family. The resulting distribution determines the probability that a loss from some catastrophe will exceed some amount. You can then set the magnitude of the catastrophe you wish to study. A "return period" of 50, for example, means that you wish to study catastrophes that are larger than all of those that will, on average, occur over a 50 year period. The Demonstration responds by drawing the distribution you have selected (you select whether you want to see the probability density function or the cumulative density function): the red dashed line shows the "exceedance value" and the blue line shows the tail conditional expectation, which is computed using numerical integration. An inset grid recapitulates the results.
Contributed by: Seth J. Chandler (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
This Demonstration was influenced in part by Dr. Gordon Woo's article "Natural Catastrophe Probable Maximum Loss," British Actuarial Journal, 8(5), 2002 pp. 943–959.
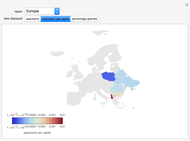
Snapshot 1: If the "damage" random variable is drawn from certain generalized Pareto distributions, the tail conditional expectation can be three times larger than the exceedance value.
Snapshot 2: If the "damage" random variable is drawn from certain Weibull distributions, the tail conditional expectation is not much larger than the exceedance value.
Snapshot 3: A model in which damage is a random variable drawn from a gamma distribution. The tail conditional expectation, though of course larger than the exceedance value, does not hugely exceed the exceedance value.
It becomes apparent from the Demonstration that the tail conditional expectation is quite sensitive to the particular family of distributions from which the random variable is drawn. Since it is often difficult from data alone to decide which family of distributions should be used, beliefs about tail conditional expectations can vary greatly and can probably be refined only by an understanding of the physical processes behind the distributions. This sensitivity to assumptions can make it difficult to resolve debates about the appropriate premiums to compensate for the risks assumed by private or governmental excess insurers.
Permanent Citation
"Tail Conditional Expectations"
http://demonstrations.wolfram.com/TailConditionalExpectations/
Wolfram Demonstrations Project
Published: March 7 2011